Let f(x) = minimum (x+1,√1−x) for all x≤1. Then the area bounded by y=f(x) and the x−axis is
73 sq. units
16 sq. units
116 sq. units
76 sq. units
The correct Answer is:D
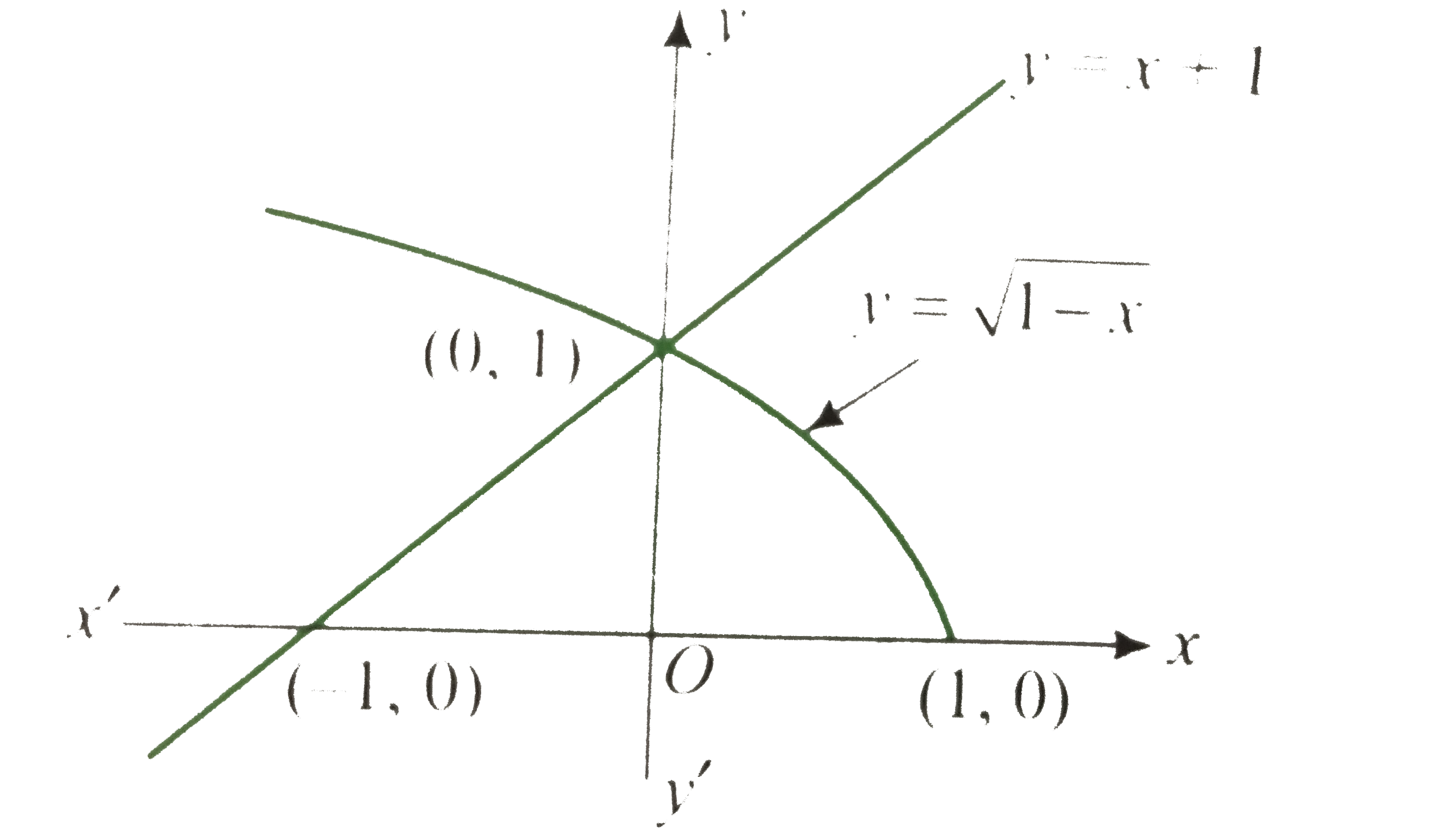
Required area =Shaded region
=1∫0(x2−x1)dy (integrating along y-axis)
=1∫0[(1−y2)−(y−1)]dy
=76 sq. unit
Topper's Solved these Questions
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Multiple)
10 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Comprehension)
21 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.3
7 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Question Bank
29 Videos
- AREA UNDER CURVESBOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank
20 Videos
Similar Questions
Knowledge Check
The area bounded by y=x+1andy=cosx and the x - axis, is
A1 sq. unitsB32 sq. unitsC14 sq. unitsD18 sq. unitsLet f(x)=min(x+1,√1−x)∀x≤1. Then, the area (in sq. units( bounded by y=f(x),y=0andx=0 from y=0 to x=1 is equal to
A13B23C49D1
Consider f(x)= minimum (x+2,√4−x),∀x≤4. If the area bounded by y=f(x) and the x - axis is 22k square units, then the value of k is
View SolutionLet f(x)=min[tanx,cotx,1√3] for all x∈(0,π2)Then the area bounded by y = f (x) and the X - axis is
View SolutionLet f(x) = minimum (tan x, cot x, 1/√3) ∀x∈[0,π/2] . Then area bounded by y = f(x) and the x-axis is log (ab)+πc√d, then a + b - c + d = _______
View SolutionLet f(x)= minimum {ex,3/2,1+e−x},0≤x≤1. Find the area bounded by y=f(x), X-axis and the line x=1.
View SolutionLet f(x) = max (x,x3)∀x∈R. Calculate the area bounded by the curves y = f(x) and the x-axis between the ordinates x = - 1 and x = 1
View SolutionLet f:[−3,1]→R be given as
f(x)={min{(x+6),x2},−3≤x≤0max{√x,x2},0≤x≤1 .
If the area bounded by y=f(x) and x-axis is A, then the value of 6A is equal to ________.
View Solution
