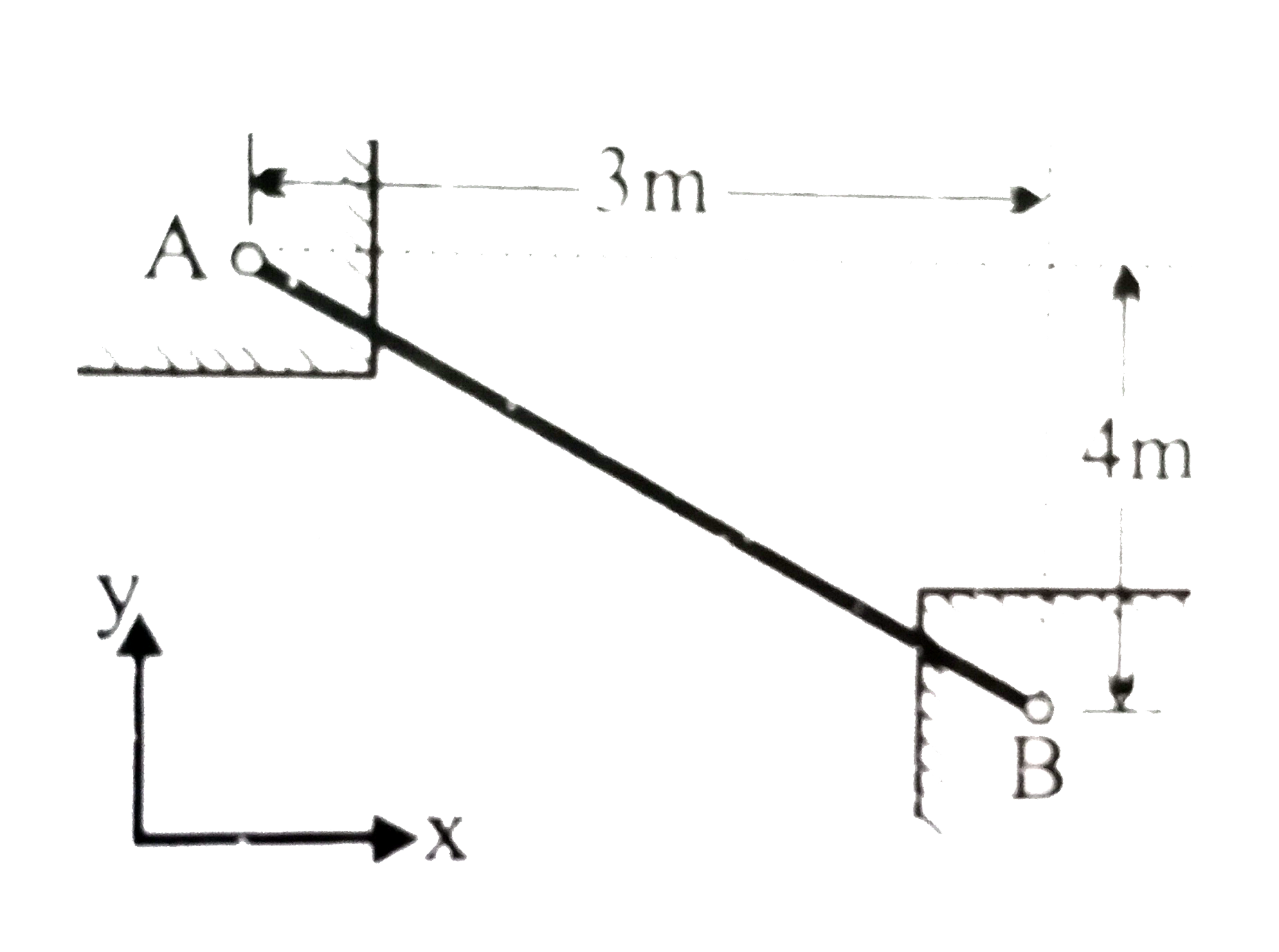
A light cable stretched between the fixed supports A and B is under a tension T of 1000 N. Express the tension as a vector using the unit vectors first, as a force acting on A and second, as a force acting on B.
The correct Answer is:A, B
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - CHEMISTRY
133 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - MATHEMATICS
58 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - MATHS SECTION - 2 [MULTIPLE CORRECT CHOICE TYPE]
6 Videos
- PROBABILITYBOOK - BANSALCHAPTER - PROBABILITYEXERCISE - All Questions
1 Videos
- THERMODYNAMICSBOOK - BANSALCHAPTER - THERMODYNAMICSEXERCISE - Match the column
7 Videos
Similar Questions
Find unit vector in direction of friction force acting on block
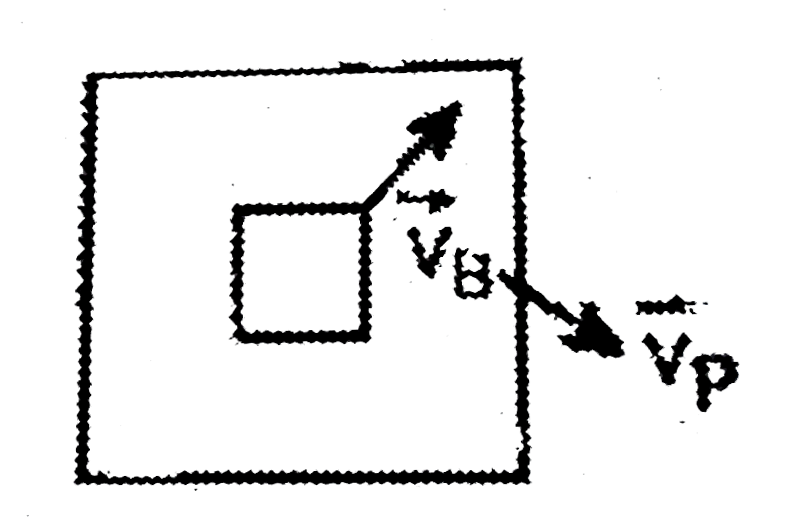
→vp=7ˆi−2ˆj,→vB=3ˆi+ˆj
View SolutionTwo masses of 10kg and 5kg are suspended from a rigid support as shown in figure. The system is pulled down with a force of 150N attached to the lower mass. The string attached to the support breaks and the system accelerates downwards
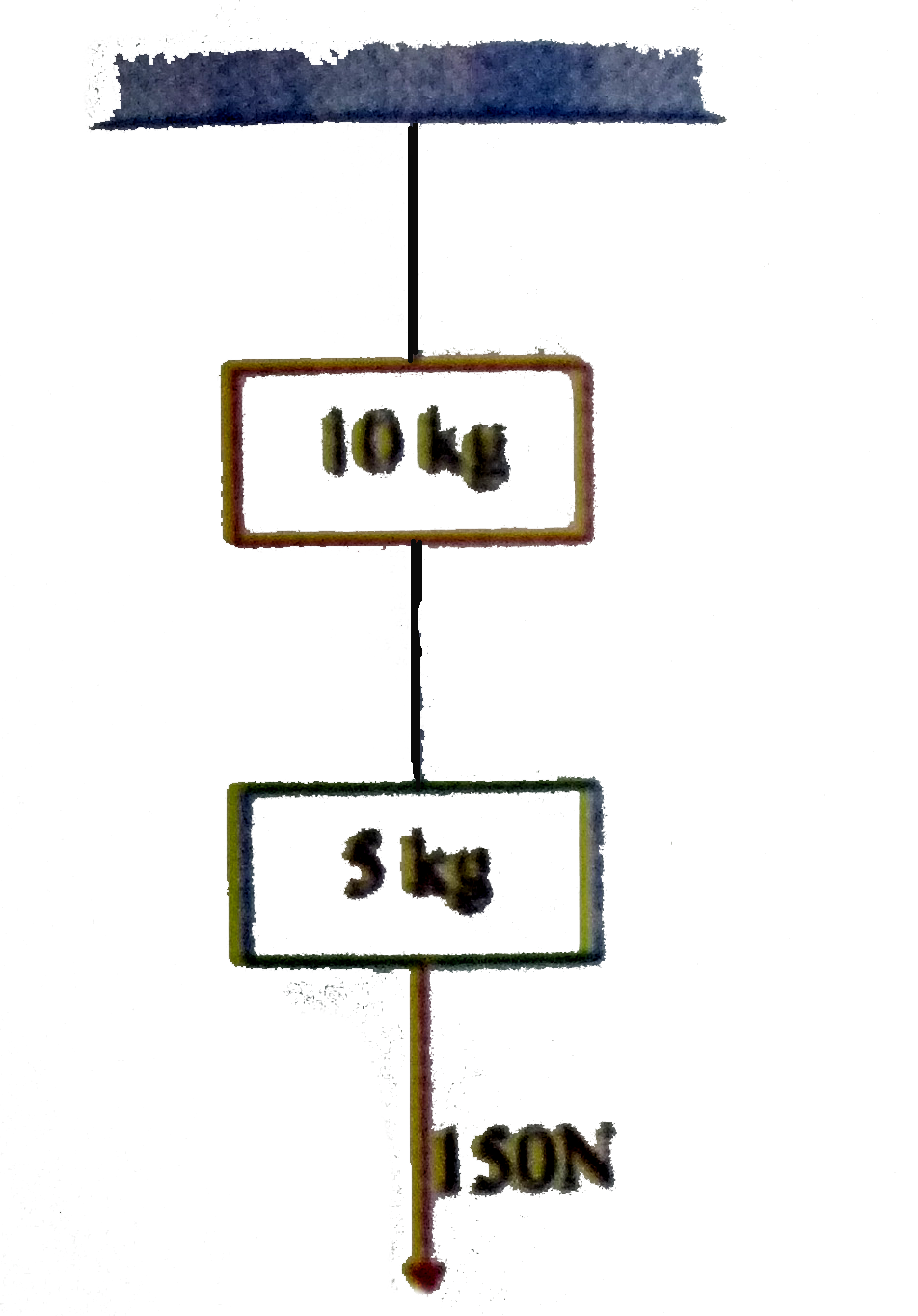
In case the force continues to act. what will be the tension acting between the two masses ? .
View Solution
Knowledge Check
Linear momentum of an object can be expressed as →P=(4cost)ˆi+(4sint)ˆj. Angle between the forces acting on the object and its linear momentum is :
A(π)/(2)B(π)/(4)C(3π)/(4)DπIf the projection of the vector a n b is |a×b| and if 3b=ˆi+ˆj+ˆk then the angle between a and b is
Aπ3Bπ2Cπ4Dπ6Two masses of 10kg and 5kg are suspended from a rigid support as shown in figure. The system is pulled down with a force of 150N attached to the lower mass. The string attached to the support breaks and the system accelerates downwards
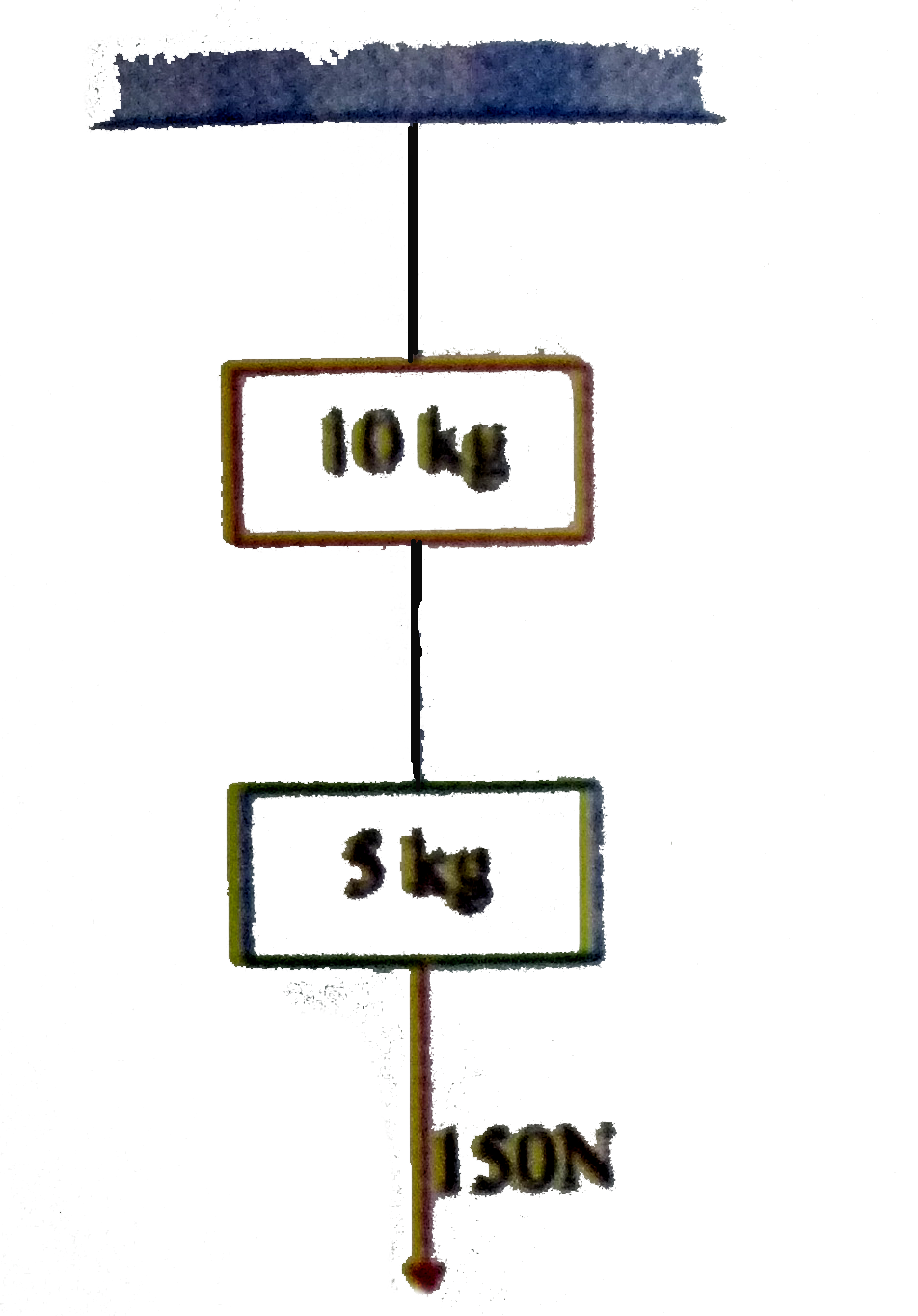
In case the force continues to act. what will be the tension acting between the two masses ? .A20m/s2B10m/s2C5m/s2Dzero
Two masses of 10kg and 5kg are suspended from a rigid support as shown in figure. The system is pulled down with a force of 150N attached to the lower mass. The string attached to the support breaks and the system accelerates downwards
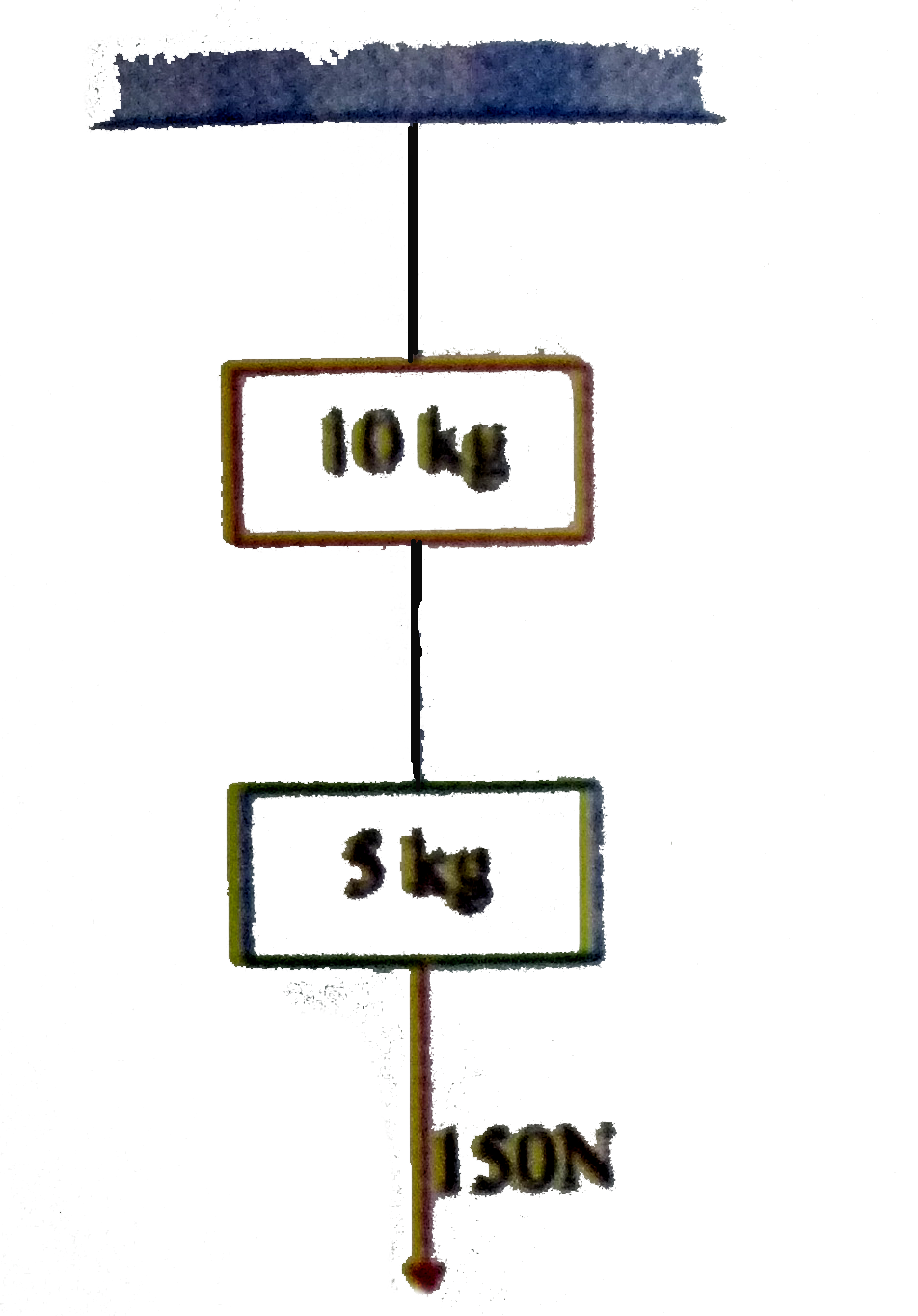
In case the force continues to act. what will be the tension acting between the two masses ? .
View SolutionIf vectors A and B be respectively equal to 3ˆi−4ˆj+5ˆkand2ˆi+3ˆj−4ˆk. Find the unit vector parallel t A + B
View SolutionIf vectors A and B be respectively equal to 3ˆi−4ˆj+5ˆkand2ˆi+3ˆj−4ˆk. Find the unit vector parallel t A + B
View SolutionTwo masses of 10 kg and 5 kg are suspended from a rigid support as shown in figure. The system is pulled down with a force of 150 N attached to the lower mass. The string attached to the support breaks and the system accelerates downwards.
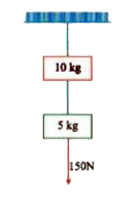
In case the force continues to act. what will be the tension acting between the two masses ?
View SolutionTwo identical strings are stretched at tensions TA and TB. A truning fork is used to set them in vibration. A vibrates in its fundamental mode and B in its second harmonic mode.
View Solution