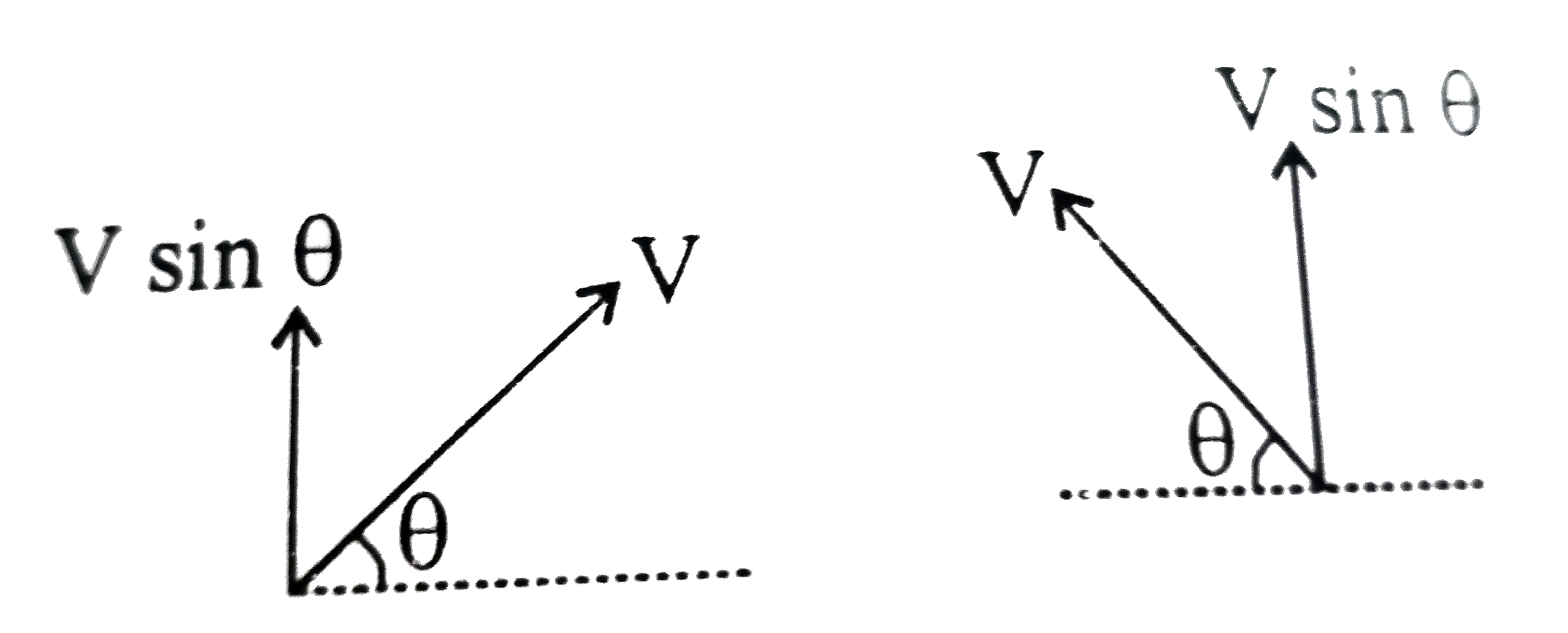
A man swims with same speed relative to river irrespective of whether water is flowing or not. He swims at an angle of θ to bank as shown in 2 figures. Velocity of river flow > Velocity of man in still water.
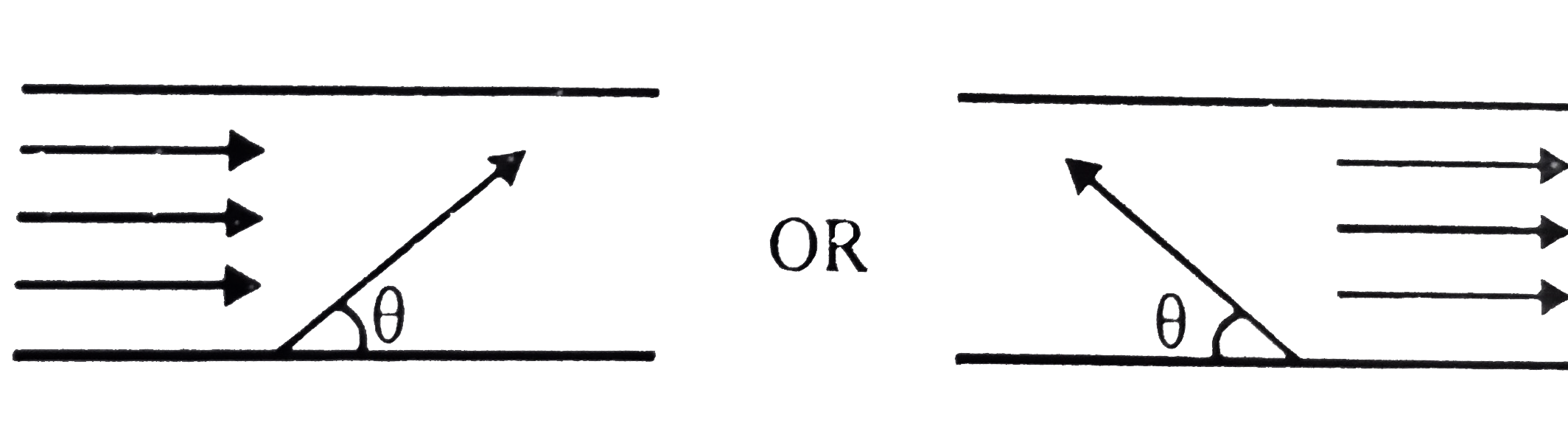
In both situations, he crosses river in same time
In both situation, he crosses the rever with same drift,
To minimize the dirft, he has to swim ⊥ to river flow,
To minimize the time, he has to swim ⊥ to river flow.
The correct Answer is:A, D
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - CHEMISTRY
133 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - MATHEMATICS
58 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - MATHS SECTION - 2 [MULTIPLE CORRECT CHOICE TYPE]
6 Videos
- PROBABILITYBOOK - BANSALCHAPTER - PROBABILITYEXERCISE - All Questions
1 Videos
- THERMODYNAMICSBOOK - BANSALCHAPTER - THERMODYNAMICSEXERCISE - Match the column
7 Videos
Similar Questions
Knowledge Check
A man can swim with a speed v relative to water . There is a river of width d which is flowing at a speed u . A is the point on river bank from where he starts and B is point directly opposite to A on the other side of the river .
AMan can directly reach point B in time d/l.BMan can cross the river in minimum time d/vCMan can reach point B directly in time d√v2−u2DIf u>v then man cannot reach the point B directly .A man who can swim at a velocity v relative to water wants to cross a river of width b, flowing with a speed u.
AThe minimum time in which he can cross the river is bvBHe can reach a point exactly opposite on the bank in time t=b√v2−u2ifv>uCHe cannot reach a point exactly opposite on the bank if u>vDHe cannot reach a point exactly opposite on the bank if v>uA man can swim at 4 m/s in a still water swimming pool. He enters a 200 m wide river at one bank and swims ( w.r.t water) at an angle of 60∘ to the river flow velocity. The river flow velocity is 5 m/s . In how much -time does he cross the river ? Calculate his drift.
A200√3s,500√3mB100√3s,700√3mC50s, 1000 mD1000√3s,200√3m
A man swims with a constant absolute velocity irrespective of the current in water. He can swim from a point P on one bank of river to a point Q just opposite the other in time t when the water is still. He takes time t1 to swim across along the same straight path PQ when the river flows down with a uniform velocity . The river velocity is btt1√(t21−t2)
where b is the breadth PQ of the river.
View SolutionA man can swim at 4 m/s in a still water swimming pool. He enters a 200 m wide river at one bank and swims ( w.r.t water) at an angle of 60∘ to the river flow velocity. The river flow velocity is 5 m/s . In how much -time does he cross the river ? Calculate his drift.
View SolutionA ,man wishing to cross a river flowing with velocity u jumps at an angle θ with the river flow.
(a) Find the net velocity of the man with respect to ground if he can swim with speed v in still water.
(b) In what direction does the boat actually move ?
( c) Find how far from the point directly opposite to the starting point does the boat reach the opposite bank, if the width of the river is d.
View SolutionA ,man wishing to cross a river flowing with velocity u jumps at an angle θ with the river flow.
(a) Find the net velocity of the man with respect to ground if he can swim with speed v in still water.
(b) In what direction does the boat actually move ?
( c) Find how far from the point directly opposite to the starting point does the boat reach the opposite bank, if the width of the river is d.
View SolutionA man swims to and fro along the bank of a river with a velocity v relative to water. If the velocity of flow is u, the average speed of the man (for to and fro motion) is :
View Solution