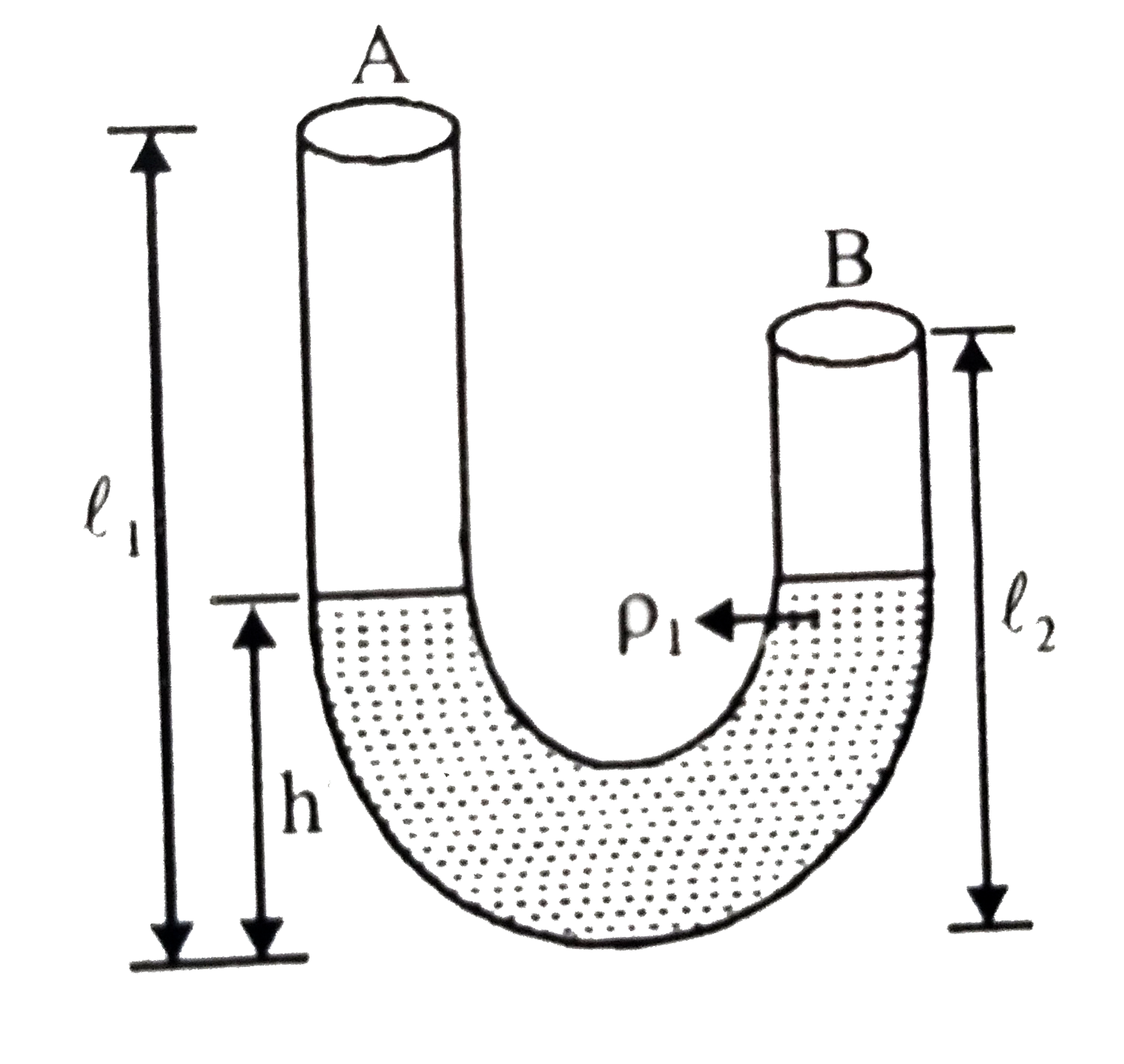
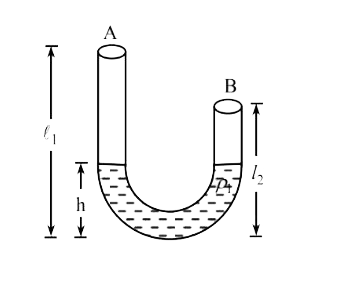
A U-tube having uniform cross-section but unequal arm length l1=100cmandl2=50cm has same liquid of density ρ1 filled in it upto a height h=30 cm as shown in figure. Another liquid of density ρ2=ρ1/2 is poured in arm A. BOth liquids are immiscible. What length of the second liquid ( in cm ) should be poured in A so that second overtone of A is in unison with fundamental tone of B. ( Neglect end correction )
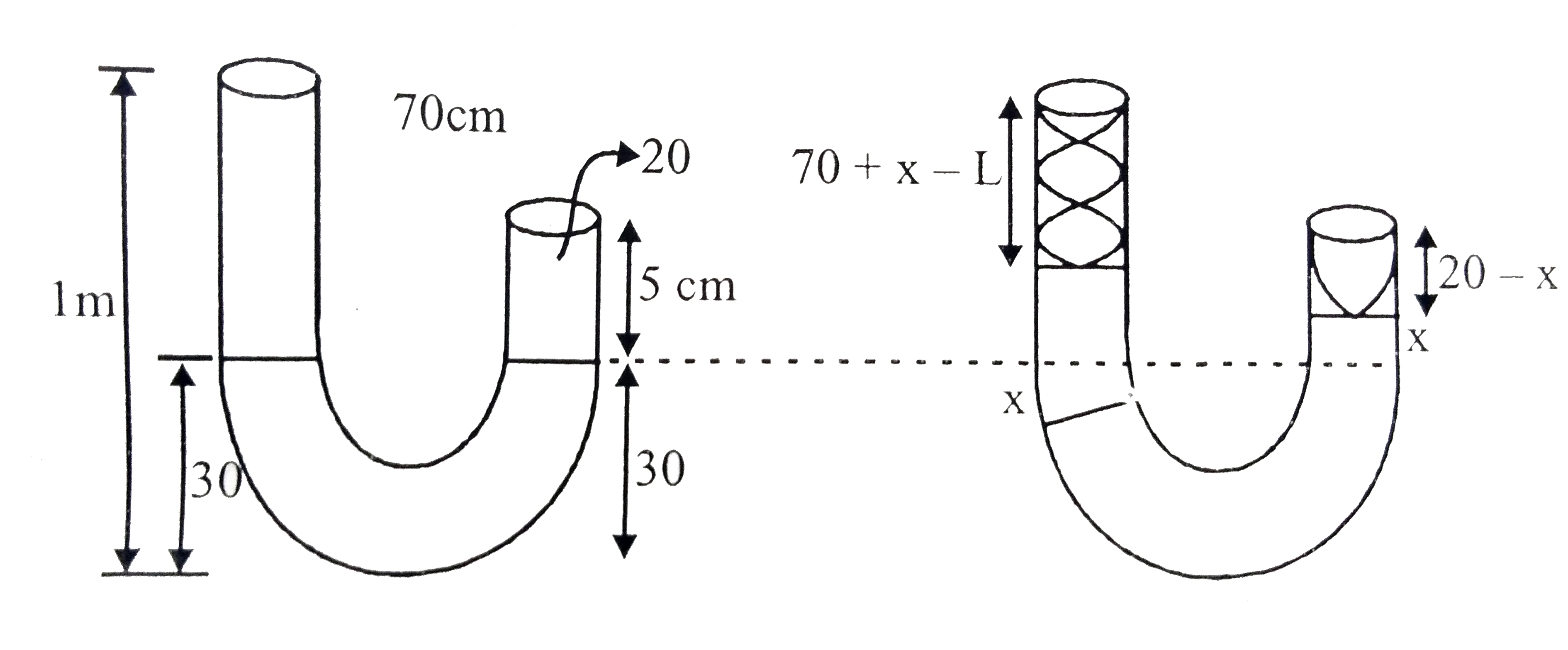
0060
2x×ρ×g=L×ρ2g
⇒L=4x
frequency same ⇒λ=same
20−x=λ4
70+3x=5λ4
70-3x=10-5x
2x=30,x=15
L=4x=60cm
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - CHEMISTRY SECTION-2 Part-C ( Integer type )
10 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - MATHS SECTION-3 Part-C[ Integer type ]
10 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - MATHS SECTION-3 Part-B[ Matrix type ]
2 Videos
- PROBABILITYBOOK - BANSALCHAPTER - PROBABILITYEXERCISE - All Questions
1 Videos
- THERMODYNAMICSBOOK - BANSALCHAPTER - THERMODYNAMICSEXERCISE - Match the column
7 Videos
Similar Questions
A U-tube having uniform cross-section but unequal arm length I1=100 cm and I2=50 cm has same liquid of density ρ1 filled in it upto a height h=30 cm as shown in figure. Another liquid of density ρ2=ρ1/2 is poured in arm. A Both liquids are immiscible What length of the second liquid (in cm) should be poured in A so that second overtone of A is in unison with fundamental tone of B. (Neglect end correction)
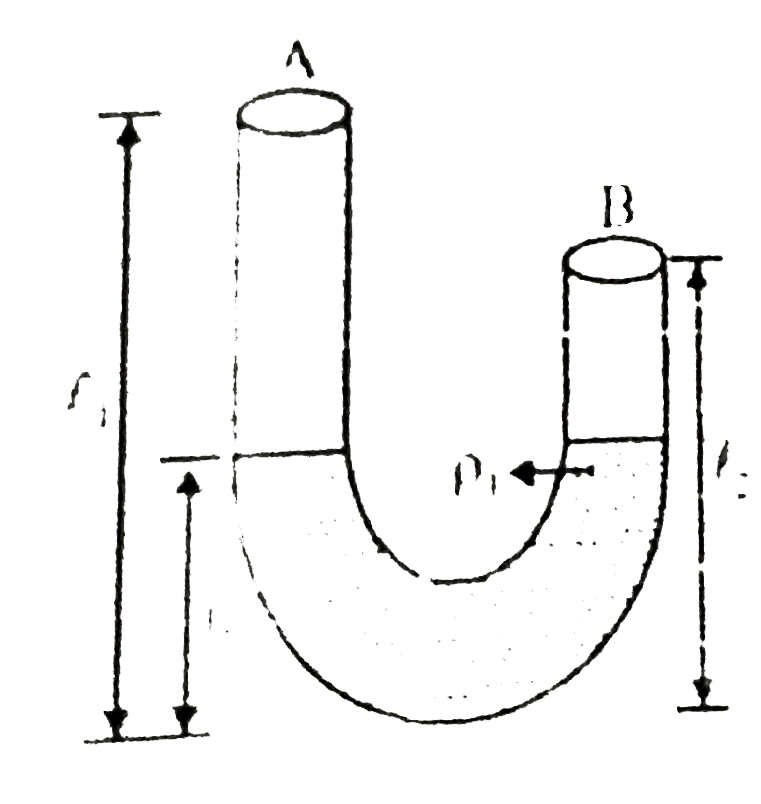
View SolutionA tube U-shaped has a uniform cross-sectionn with arm length l1andl2(l1>l2). Tube has a liquid of density ρ1 filled to a height h. another liquid of density ρ2=(ρ1)14 is poured in arm A. both liquids are immiscible. the length of second liquid that should be poured in A so that first overtone of A is in unisom with fundamental tone of B is:
View Solution
Knowledge Check
A tube U-shaped has a uniform cross-sectionn with arm length l1andl2(l1>l2). Tube has a liquid of density ρ1 filled to a height h. another liquid of density ρ2=(ρ1)14 is poured in arm A. both liquids are immiscible. the length of second liquid that should be poured in A so that first overtone of A is in unisom with fundamental tone of B is:
 A2(J1−3J2−2h)B2(J1−3J2+2h)C2(J1+3J2+2h)D2(J1−3J2+2h)
A2(J1−3J2−2h)B2(J1−3J2+2h)C2(J1+3J2+2h)D2(J1−3J2+2h)A body floats in a liquid A of density ρ1 with a part of it submerged inside liquid while in liquid B of density ρ2 totally submerged inside liquid. The densities ρ1 and ρ2 are related as :
Aρ1=ρ2Bρ1<ρ2Cρ1>ρ2Dnothing can be saidThree different liquids are filled in a U-tube as shown in figure. Their densities are ρ1,ρ2 and ρ3 respectively. From the figure we may conclude that
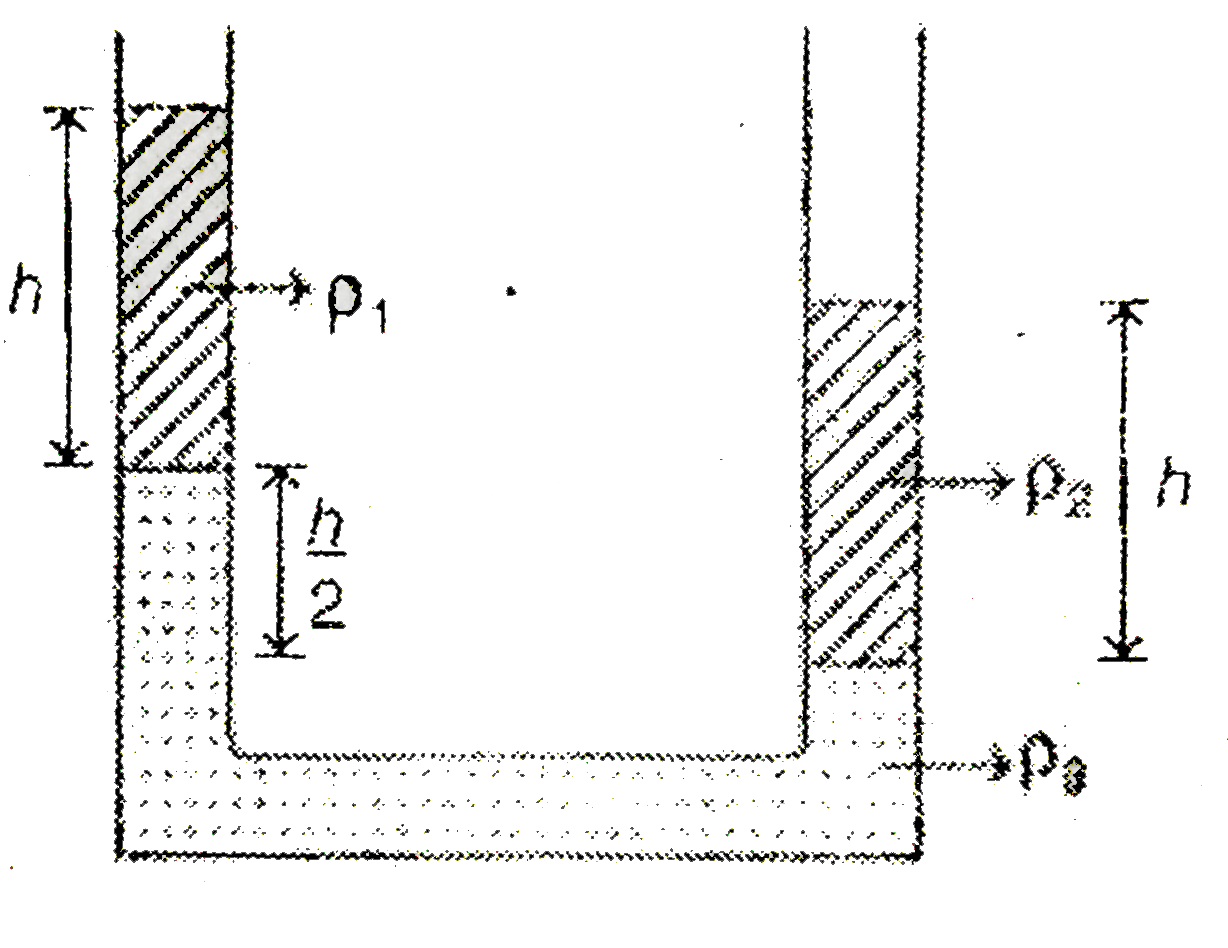 Aρ2>ρ1Bρ1>ρ2Cρ3=2(ρ2−ρ1)Dρ3=ρ2+ρ12
Aρ2>ρ1Bρ1>ρ2Cρ3=2(ρ2−ρ1)Dρ3=ρ2+ρ12
A body weighs W1 in a liquid of density ρ1 and W2 in a liquid of density ρ2. What is the weight of body in a liquid of density ρ3?
View SolutionA body weighs W1 in a liquid of density ρ1 and W2 in a liquid of density ρ2. What is the weight of body in a liquid of density ρ3?
View SolutionA non viscous liquid of density ρ is filled in a tube with A as the area of cross section, as shown in the figure. If the liquid is slightly depressed in one of the arms, the liquid column oscillates with a frequency
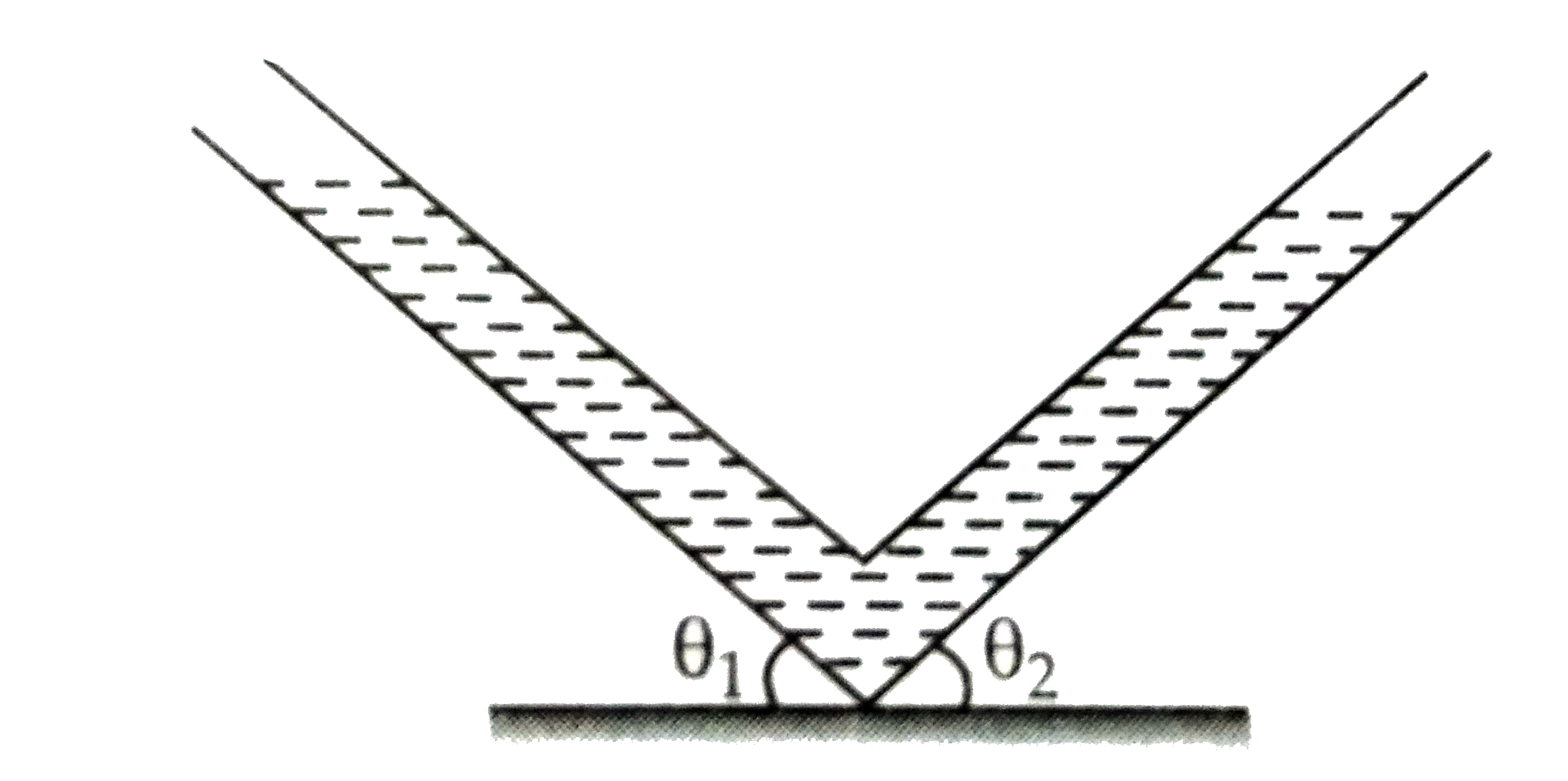
View SolutionThree different liquids are filled in a U-tube as shown in figure. Their densities are ρ1,ρ2 and ρ3 respectively. From the figure we may conclude that
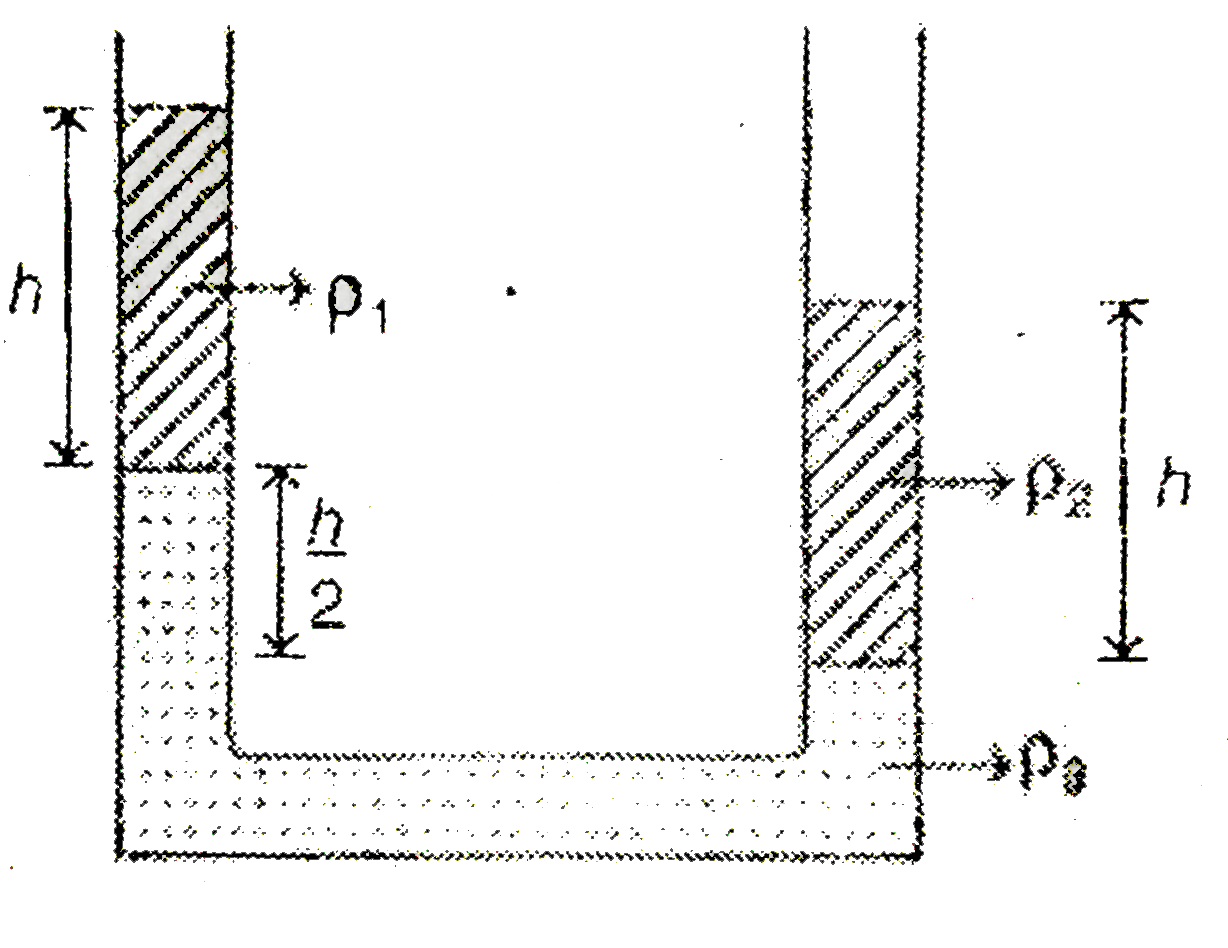
View SolutionA body of density ρ floats with volume V1 of its total volume V immersed in a liquid of density ρ1 and with the remainder of volume V2 immersed in another liquid of density ρ2 where ρ1>ρ2. Find the volume immersed in two liquids. (V1 and V2).
View Solution
