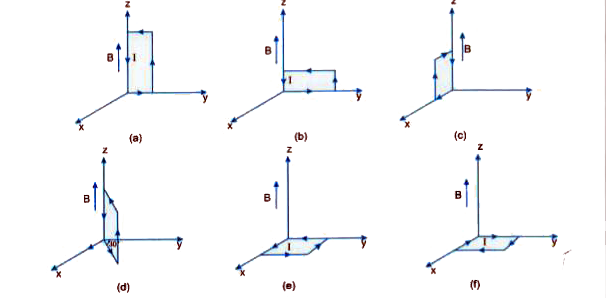
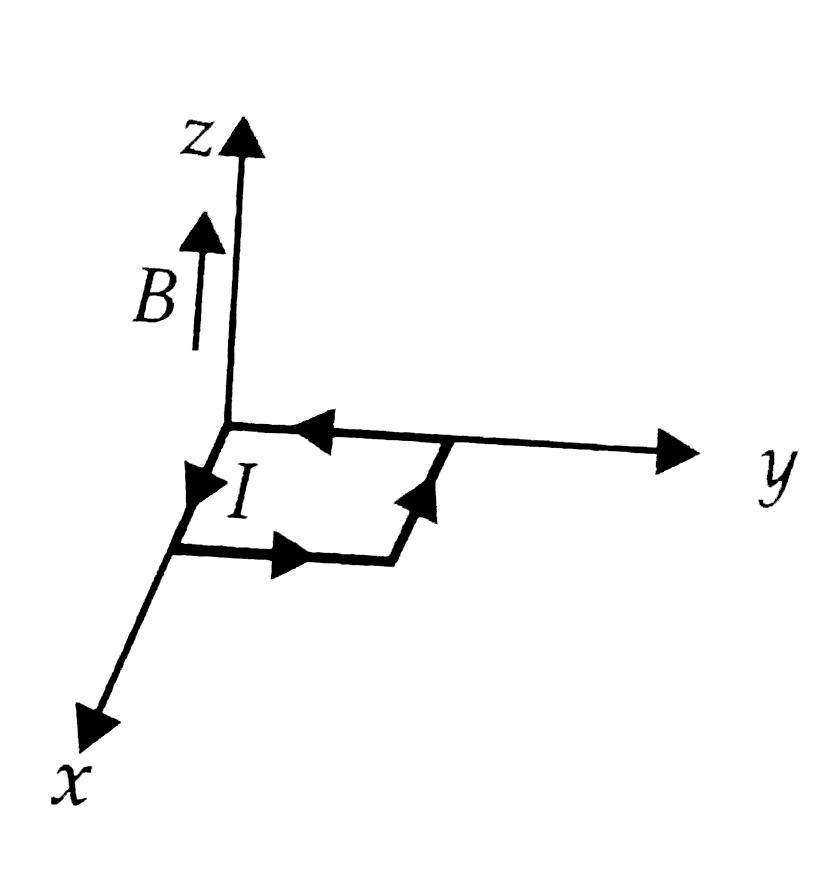
A uniform magnetic field B of0.3 T is along the positive Z-direction. A rectangular loop (abed) of sides 10cm×5cm carries a current I of 12 A. Out of the following different orientations which one corresponds to stable equilibrium?
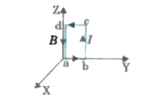
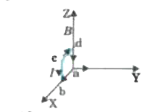
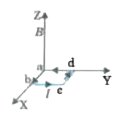
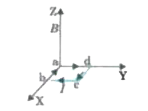
The correct Answer is:c
To determine which orientation of a rectangular loop carrying a current in a uniform magnetic field corresponds to stable equilibrium, we can follow these steps:
Step 1: Understand the Magnetic Moment
The magnetic moment →M of a current-carrying loop is given by:
→M=I⋅A
where I is the current and A is the area vector of the loop. The direction of →M is determined by the right-hand rule: if you curl the fingers of your right hand in the direction of the current, your thumb points in the direction of →M.
Step 2: Determine the Torque on the Loop
The torque →τ experienced by the loop in a magnetic field →B is given by:
→τ=→M×→B
For stable equilibrium, the torque should be zero, which occurs when →M and →B are parallel (0 degrees) or anti-parallel (180 degrees).
Step 3: Analyze Each Orientation
1. First Orientation: Current is anti-clockwise.
- →M points out of the plane (positive Z-direction).
- →B is also in the positive Z-direction.
- Since both are parallel, torque →τ=0. This is a stable equilibrium.
2. Second Orientation: Current flows in a different direction.
- Determine the direction of →M using the right-hand rule.
- If →M is in the negative Y-direction and →B is in the positive Z-direction, then →M and →B are not parallel, resulting in a non-zero torque. This is not stable.
3. Third Orientation: Current flows in a way that →M is in the positive Z-direction and →B is also in the positive Z-direction.
- Thus, →M and →B are parallel, resulting in zero torque. This is stable.
4. Fourth Orientation: Current flows such that →M is in the negative Z-direction and →B is in the positive Z-direction.
- Here, →M and →B are anti-parallel, resulting in zero torque, but this is an unstable equilibrium because the system has higher potential energy.
Conclusion
The orientation that corresponds to stable equilibrium is the first orientation, where the magnetic moment is parallel to the magnetic field.
The magnetic moment →M of a current-carrying loop is given by:
→M=I⋅A
where I is the current and A is the area vector of the loop. The direction of →M is determined by the right-hand rule: if you curl the fingers of your right hand in the direction of the current, your thumb points in the direction of →M.
The torque →τ experienced by the loop in a magnetic field →B is given by:
→τ=→M×→B
For stable equilibrium, the torque should be zero, which occurs when →M and →B are parallel (0 degrees) or anti-parallel (180 degrees).
1. First Orientation: Current is anti-clockwise.
- →M points out of the plane (positive Z-direction).
- →B is also in the positive Z-direction.
- Since both are parallel, torque →τ=0. This is a stable equilibrium.
- Determine the direction of →M using the right-hand rule.
- If →M is in the negative Y-direction and →B is in the positive Z-direction, then →M and →B are not parallel, resulting in a non-zero torque. This is not stable.
- Thus, →M and →B are parallel, resulting in zero torque. This is stable.
- Here, →M and →B are anti-parallel, resulting in zero torque, but this is an unstable equilibrium because the system has higher potential energy.
The orientation that corresponds to stable equilibrium is the first orientation, where the magnetic moment is parallel to the magnetic field.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - COMPETITION FILE (C. MULTIPLE CHOICE QUESTIONS)28videos
MOVING CHARGES AND MAGNETISM
- BOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - COMPETITION FILE (D. MULTIPLE CHOICE QUESTIONS)10videos
MOVING CHARGES AND MAGNETISM
- BOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - COMPETITION FILE (A. MULTIPLE CHOICE QUESTIONS)38videos
MOVING CHARGES AND MAGNETISM
- BOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION16videos
MAGNETISM AND MATTER
- BOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION15videos
NUCLEI
Similar Questions
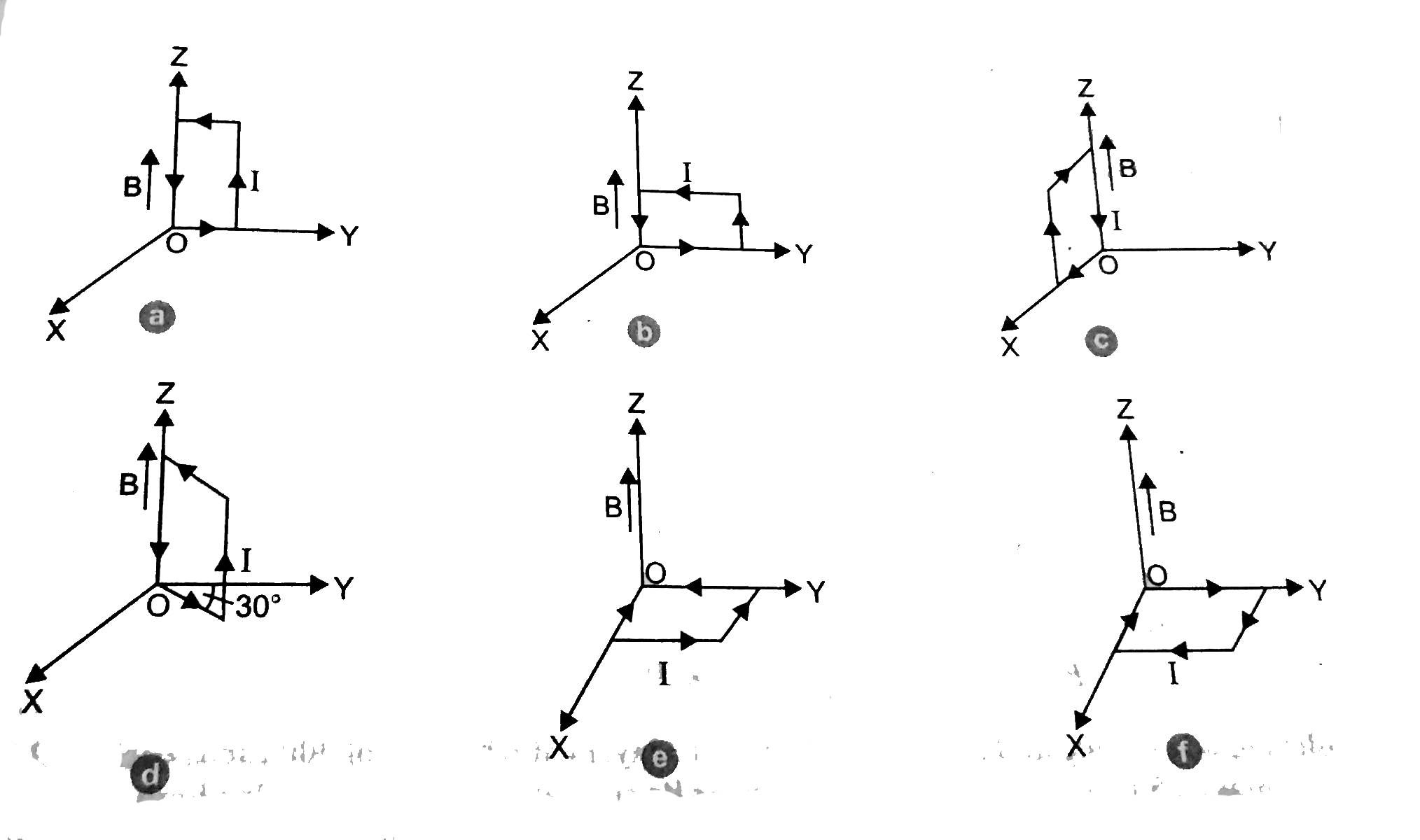
A unform magnetic field of 3000 G is established along the positive z-direction. A rectangular loop of sides 10 cm and 5 cm carries a current of 12 A . What is the torque on the loop in the different cases shown in the fig ? What is the force on each case? Which case corresponds to stable equilibrium ?
 View Solution
View SolutionA uniform magnetic field of 3000G is established along the positive z-direction. A rectangular loop of sides 10cm and 5cm carries a current 12A. What is the torque on the loop in the different cases shown in the figure. What is the force on each case? Which case corresponds to stable equilibrium?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A uniformi magnetic field of 3 T is established along the positive Z-direction. A rectangular loop in XY plane of sides 10 cm and 5 cm carries a current of 11 A as shown The torque on the loop is:
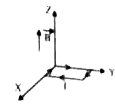 A+1.8×10−2ˆjNmB−1.8×10−2ˆjNmCZeroD−1.8×10−2ˆjNm
A+1.8×10−2ˆjNmB−1.8×10−2ˆjNmCZeroD−1.8×10−2ˆjNm - Question 2 - Select One
A uniform magnetic field of 1000 G is established along the positive z-direction. A rectangular loop of sides 10 cm and 5 cm carries a current of 12 A. What is the torque on the loop as shown in the figure?
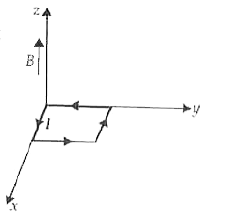 AzeroB1.8×10−2NmC1.8×10−3NmD1.8×10−4Nm
AzeroB1.8×10−2NmC1.8×10−3NmD1.8×10−4Nm - Question 3 - Select One
A uniform magnetic field of 1000 G is established along the positive z-direction. A rectangular loop of sides 10 cm and 5 cm carries a current of 12 A. What is the torque on the loop as shown in the figure?
 AZeroB1.8×10−2NmC1.8×10−3NmD1.8×10−4Nm
AZeroB1.8×10−2NmC1.8×10−3NmD1.8×10−4Nm
A square shaped plane coil of area 100cm2 turns a carries a steady current of 5 A . It is placed in a uniform magnetic field of 0.2 T acting perpendicular to the plane of the coil. Calculate the torque on the coil when its when makes an angle of 60∘ with the direction of the field. In which orinetation will the coil be in stable equilibrium?
View SolutionA rectangular loop of dimensions (a×b) carries a current i. A uniform magnetic field →B=B0ˆi exists in space. Then :
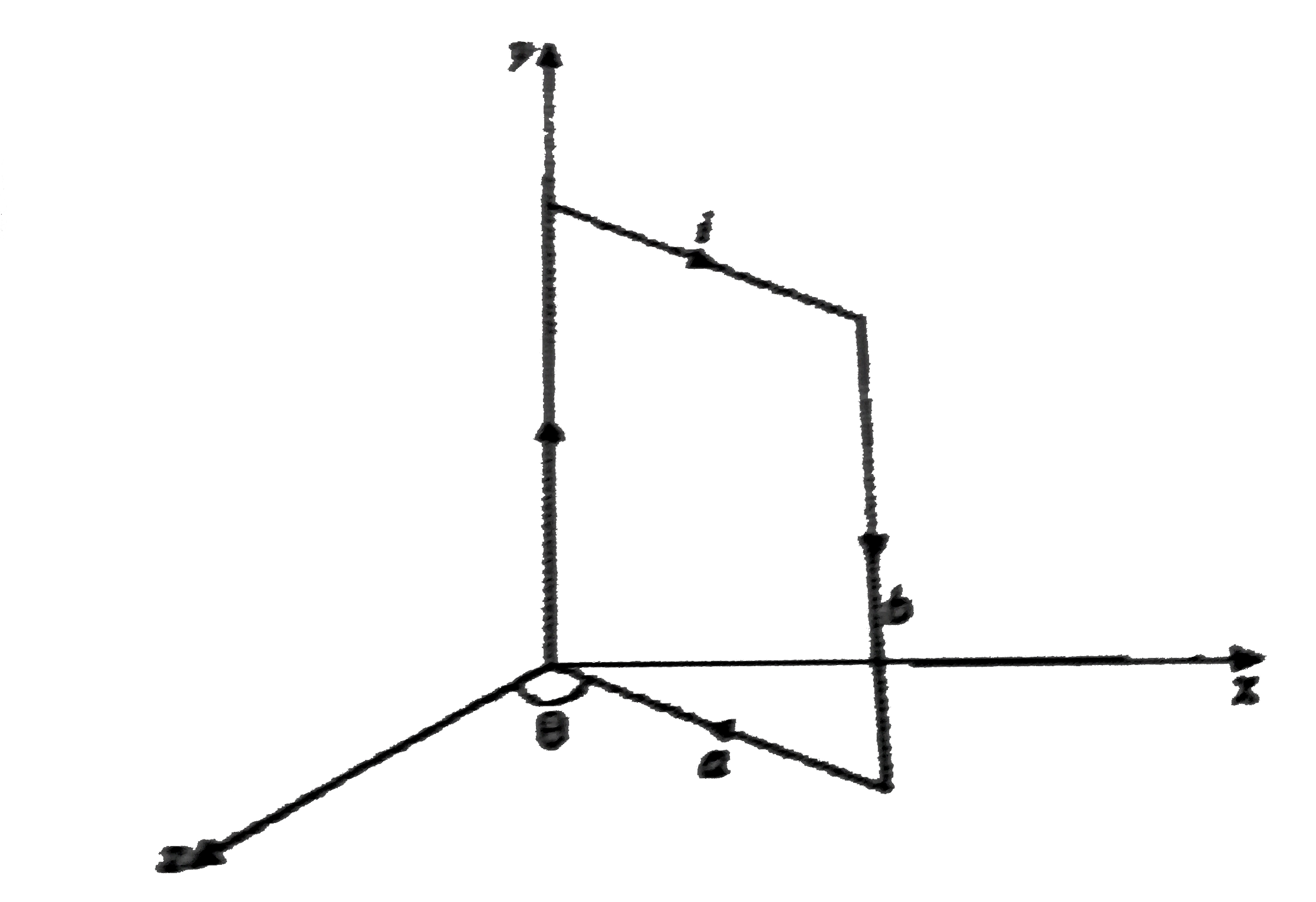 View Solution
View SolutionA short bar magnet placed with its axis at 30∘ to a uniform magnetic field of 0.02 T experiences a torque of 0.060 Nm. (i) Calculate magnetic moment of the magnet, and (ii) find out what orientation of the magnet corresponds to its stable equilibrium in the magnetic field.
View SolutionA triangular loop of side 1 carries a current I. It is placed in a magnetic field B such that the plane of the loop is in the direction of B. The torque on the loop is
View SolutionA rectangular loop of sides 20cm and 10cm carries a current of 5.0A. A uniform magnetic field of magnitude 2T exists parallel to the longer side of the loop. The torque acting on the loop is
View Solution

