P is a point outside a circle and is 13 cm away from its centre. A secant drawn from the point P intersects the circle at points A and B in such a way that PA=9 cm and AB=7 cm. The radius of the circle is
5.5 cm
5 cm
4 cm
4.5 cm
The correct Answer is:B
To find the radius of the circle given the conditions of the problem, we can follow these steps:
1. Identify the Given Information:
- Distance from point P to the center O of the circle, OP=13 cm.
- Length from P to A, PA=9 cm.
- Length of segment AB, AB=7 cm.
2. Calculate PB:
- Since PB=PA+AB, we can calculate:
PB=PA+AB=9cm+7cm=16cm.
3. Use the Intersecting Secant Theorem:
- According to the theorem, we have:
PK×PQ=PA×PB.
- Here, PK=OP−OK and PQ=OP+OK, where OK is the radius R of the circle.
4. Express PK and PQ in terms of R:
- Thus, we can express:
PK=13−R,
PQ=13+R.
5. Substitute the Values into the Theorem:
- Now substituting the values into the theorem:
(13−R)(13+R)=9×16.
6. Simplify the Left Side:
- The left side can be simplified using the difference of squares:
132−R2=144.
- Calculating 132:
169−R2=144.
7. Rearranging the Equation:
- Rearranging gives:
R2=169−144,
R2=25.
8. Finding the Radius R:
- Taking the square root of both sides:
R=√25=5cm.
Final Answer:
The radius of the circle is R=5cm.
---
- Distance from point P to the center O of the circle, OP=13 cm.
- Length from P to A, PA=9 cm.
- Length of segment AB, AB=7 cm.
- Since PB=PA+AB, we can calculate:
PB=PA+AB=9cm+7cm=16cm.
- According to the theorem, we have:
PK×PQ=PA×PB.
- Here, PK=OP−OK and PQ=OP+OK, where OK is the radius R of the circle.
- Thus, we can express:
PK=13−R,
PQ=13+R.
- Now substituting the values into the theorem:
(13−R)(13+R)=9×16.
- The left side can be simplified using the difference of squares:
132−R2=144.
- Calculating 132:
169−R2=144.
- Rearranging gives:
R2=169−144,
R2=25.
- Taking the square root of both sides:
R=√25=5cm.
The radius of the circle is R=5cm.
Topper's Solved these Questions
- BOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.145videos
GEOMETRY
- BOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.2100videos
GEOMETRY
- BOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - EXERCISE(LEVEL 2)52videos
GEOMETRY
- BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 240videos
FUNDAMENTALS
- BOOK - ARIHANT SSCCHAPTER - HCF AND LCMEXERCISE - Exercise Higher Skill Level Questions18videos
HCF AND LCM
Similar Questions
P is a point out side a circle, which is 13 cm from the centre. A line drawn from point P intersects the circle at point A and B such that PA = 9 cm and AB = 7 cm. Then find the radius of the circle.
View SolutionDraw a circle of radius 4 cm. From the point 7 cm away from its centre, construct the pair of tangents to the circle.
View Solution
Knowledge Check
- Question 1 - Select One
P is a point outside a circle and is 26cm away from its centre.A secant PAB drawn from intersects the circle at points A and B such that PB = 32 cm and PA = 18 cm. The radius of the circle ( in cm ) is :
A12B8C10D13 - Question 2 - Select One
P is a point outside a circle with centre O, and it is 14 cm away from the centre. A secant PAB drawn from P intersects the circle at the points A and B such that PA = 10 cm and PB = 16 cm. The diameter of the Circle is:
A10 cmB13 cmC12 cmD11 cm - Question 3 - Select One
P is a point outside the circle at a distance of 6.5 cm from centre O of the circle . PR be a secant such that it intersects the circle at Q and R . If PQ=4.5 cm and QR=3.5 cm , then what is the radius ( in cm ) of the circle ?
A1.5B2C2.5D3
From a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the centre is 25 cm. The radius of circle is :
View Solution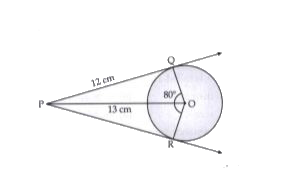
A point P is 13 cm from the centre of the circle. The two tangent PQ and PR are drawn from the point P, The length of the tangent drawn from P to the circle is 12 cm.
Find the radius of the circle.View SolutionFrom a point Q, 13 cm away from the centre of a circle, the length of tangent PQ to the circle is 12 cm. The radius of the circle (in cm) is
View SolutionP is a point outside the circle at a distance of 6.5 cm from centre O of the circle . PR be a secant such that it intersects the circle at Q and R . If PQ=4.5 cm and QR=3.5 cm , then what is the radius ( in cm ) of the circle ?
View SolutionFrom a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the centre is 25 cm. The radius of circle is :
View Solution

