P is a point out side a circle, which is 13 cm from the centre. A line drawn from point P intersects the circle at point A and B such that PA = 9 cm and AB = 7 cm. Then find the radius of the circle.
a)5 cm
b)4 cm
c)4.5 cm
d)5.5 cm
To solve the problem step by step, we will use the Power of a Point theorem, which states that for a point outside a circle, the product of the lengths of the segments from the point to the points of intersection with the circle is equal to the square of the length of the tangent segment from the point to the circle.
Step 1: Identify the given information
- Distance from point P to the center O of the circle (OP) = 13 cm
- Length of segment PA = 9 cm
- Length of segment AB = 7 cm
Step 2: Calculate the length of segment PB
Since PB = PA + AB,
PB=9cm+7cm=16cm
Step 3: Define the radius of the circle
Let the radius of the circle be R.
Step 4: Use the Power of a Point theorem
According to the Power of a Point theorem:
PA×PB=PO2−R2
Where:
- PA=9cm
- PB=16cm
- PO=13cm
Step 5: Substitute the known values into the equation
Substituting the values we have:
9×16=132−R2
Step 6: Calculate the left side
Calculating the left side:
9×16=144
Step 7: Calculate the right side
Calculating the right side:
132=169
So, we have:
144=169−R2
Step 8: Rearrange the equation to solve for R2
Rearranging gives:
R2=169−144
R2=25
Step 9: Take the square root to find R
Taking the square root of both sides:
R=√25
R=5cm
Conclusion
The radius of the circle is R=5cm.
---
- Distance from point P to the center O of the circle (OP) = 13 cm
- Length of segment PA = 9 cm
- Length of segment AB = 7 cm
Since PB = PA + AB,
PB=9cm+7cm=16cm
Let the radius of the circle be R.
According to the Power of a Point theorem:
PA×PB=PO2−R2
Where:
- PA=9cm
- PB=16cm
- PO=13cm
Substituting the values we have:
9×16=132−R2
Calculating the left side:
9×16=144
Calculating the right side:
132=169
So, we have:
144=169−R2
Rearranging gives:
R2=169−144
R2=25
Taking the square root of both sides:
R=√25
R=5cm
The radius of the circle is R=5cm.
Topper's Solved these Questions
Similar Questions
If the tangent at a point P to a circle with centre O cuts a line through O at Q such that PQ=24cm and OQ=25cm . Find the radius of the circle.
View SolutionIn the given figure, TB is a chord which passes through the centre of the circle. PT is a tangent to the circle at the point T on the circle. If PT = 10 cm, PA = 5 cm and AB = x cm. then the radius of the circle is:
View Solution
Knowledge Check
P is a point outside a circle and is 13 cm away from its centre. A secant drawn from the point P intersects the circle at points A and B in such a way that PA=9 cm and AB=7 cm. The radius of the circle is
A5.5 cmB5 cmC4 cmD4.5 cmP is a point outside a circle and is 26cm away from its centre.A secant PAB drawn from intersects the circle at points A and B such that PB = 32 cm and PA = 18 cm. The radius of the circle ( in cm ) is :
A12B8C10D13P is a point outside a circle with centre O, and it is 14 cm away from the centre. A secant PAB drawn from P intersects the circle at the points A and B such that PA = 10 cm and PB = 16 cm. The diameter of the Circle is:
A10 cmB13 cmC12 cmD11 cm
Two tangents are drawn from a point P on the circle at point A and B. O is the centre of the circle. If ∠AOP=60∘ then find ∠APB.
View SolutionFrom a point P, 10cm away from the centre of a circle, a tangent PT of length 8cm is drawn. Find the radius of the circle.
View Solution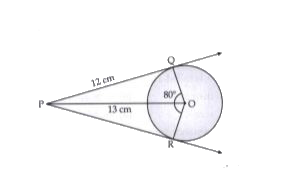
A point P is 13 cm from the centre of the circle. The two tangent PQ and PR are drawn from the point P, The length of the tangent drawn from P to the circle is 12 cm.
Find the radius of the circle.
View SolutionLength of a tangent drawn to a circle with radius 3 cm from a point 4 cm from the centre of the circle is
View SolutionPoint A is situated at a distance of 6.5 cm from the centre of a circle. The length of tangent drawn from point A to the circle is 6 cm. What is the radius of the circle?
View Solution
