Let ABCD be a parallelogram and ABEF be a rectangle with EF lying along the line CD . If AB=7cm and BE=6.5cm , then the area of the parallelogram is 11.375cm2 (b) 22.75cm2 (c) 45cm2 (d) 45.5cm2
Step by step video & image solution for Let A B C D be a parallelogram and A B E F be a rectangle with E F lying along the line C D . If A B=7c m and B E=6. 5 c m , then the area of the parallelogram is 11. 375 c m^2 (b) 22. 75 c m^2 (c) 45 c m^2 (d) 45. 5 c m^2 by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In Fig. 21, if AB=35 cm, AD=20 cm and area of the parallelogram is 560 cm2 find LB.
View SolutionA rectangle and a parallelogram are drawn between the same parallel lines on a common base of 10 cm. If the perimeter of the rectangle is 36 cm, then the area of the parallelogram is 60cm2 (b) 80cm2 (c) 81cm2 (d) 100cm2
View SolutionABCD is a rectangle with O as any point in its interior. If ar (AOD)=3 cm2and ar (BOC)=6 cm2, then area of rectangle ABCD is: 9cm2 (b) 12 cm2 (c) 15cm2 (d) 18 cm2,
View SolutionABCDEF , ar(ABC)=9cm2 , ar(DEF)=16cm2 . If BC=2.1cm , then the measure of EF is 2.8cm (b) 4.2cm (c) 2.5cm (d) 4.1cm
View SolutionA rectangle and a parallelogram have equal areas. If the sides of the rectangle are 10 m and 12 m and the base of the parallelogram is 20 m, then the altitude of the parallelogram is (a) 3 m (b) 5 m (c) 6 m (d) 7 m
View SolutionThe area of a circle is 220 cm2 . The area of a square inscribed in it is (a) 49 cm2 (b) 70 cm2 (c) 140 cm2 (d) 150 cm2
View SolutionIn Figure, ABCD is a rectangle in which CD=6cm,AD=8cm Find the area of parallelogram CDEF
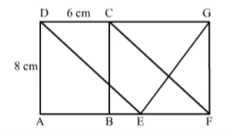
View SolutionIn Fig. 21, ABCD is a parallelogram, DL⊥AB. If AB=20 cm , AD=13 cm and area of the parallelogram is 100 cm2 find AL.
View SolutionThe hour hand of a clock is 6 m long. The area swept by it between 11.20 am and 11.55 am is (a) 2.75 cm2 (b) 5.5 cm2 (c) 11 cm2 (d) 10 cm2
View SolutionABCDEF . If BC=3cm , EF=4cm and ar(ABC)=54cm2 , then ar(DEF)= 108cm2 (b) 96cm2 (c) 48cm2 (d) 100cm2
View Solution
