A metal disc of radius R resistivity ρ thickness y is subjected into a vertical magnetic field of induction B =B0 Sin ωt where ω=2πf. Find the total power loss in the metal disc.
To find the total power loss in the metal disc subjected to a varying magnetic field, we can follow these steps:
Step 1: Understanding the Problem
We have a metal disc of radius R, resistivity ρ, and thickness y placed in a vertical magnetic field given by:
B(t)=B0sin(ωt)
where ω=2πf.
Step 2: Induced EMF Calculation
Since the magnetic field is changing with time, an electromotive force (EMF) will be induced in the disc. The induced EMF (ϵ) can be calculated using Faraday's law of electromagnetic induction:
ϵ=−dΦdt
where Φ is the magnetic flux.
Step 3: Magnetic Flux Calculation
The magnetic flux Φ through the disc is given by:
Φ=B⋅A=B0sin(ωt)⋅πR2
where A=πR2 is the area of the disc.
Step 4: Differentiate to Find EMF
Now, we differentiate the magnetic flux to find the induced EMF:
ϵ=−ddt(B0sin(ωt)⋅πR2)=−πR2B0ωcos(ωt)
Step 5: Calculate Resistance of the Element
Consider a small ring of thickness dr at a distance r from the center. The resistance R0 of this ring can be expressed as:
R0=ρlA
where l is the length of the ring (circumference) and A is the area of the ring. For the ring:
l=2πr,A=dr⋅y
Thus,
R0=ρ(2πr)dr⋅y
Step 6: Calculate Power Loss in the Element
The power loss dP in this small ring due to the induced current can be expressed as:
dP=ϵ2R0
Substituting the values of ϵ and R0:
dP=(πR2B0ωcos(ωt))2ρ(2πr)dr⋅y=π2R4B20ω2cos2(ωt)⋅dr⋅y2ρr
Step 7: Integrate to Find Total Power Loss
Now, we integrate dP from r=0 to r=R:
P=∫R0dP=∫R0π2R4B20ω2cos2(ωt)⋅dr⋅y2ρr
This integral evaluates to:
P=π2B20ω2y2ρ∫R0R4cos2(ωt)⋅dr
Step 8: Average Power Calculation
To find the average power over one cycle, we use the average value of cos2(ωt), which is 12:
⟨P⟩=π2B20ω2yR44ρ
Final Result
Thus, the total average power loss in the metal disc is given by:
⟨P⟩=π3B20f2R4y4ρ
We have a metal disc of radius R, resistivity ρ, and thickness y placed in a vertical magnetic field given by:
B(t)=B0sin(ωt)
where ω=2πf.
Since the magnetic field is changing with time, an electromotive force (EMF) will be induced in the disc. The induced EMF (ϵ) can be calculated using Faraday's law of electromagnetic induction:
ϵ=−dΦdt
where Φ is the magnetic flux.
The magnetic flux Φ through the disc is given by:
Φ=B⋅A=B0sin(ωt)⋅πR2
where A=πR2 is the area of the disc.
Now, we differentiate the magnetic flux to find the induced EMF:
ϵ=−ddt(B0sin(ωt)⋅πR2)=−πR2B0ωcos(ωt)
Consider a small ring of thickness dr at a distance r from the center. The resistance R0 of this ring can be expressed as:
R0=ρlA
where l is the length of the ring (circumference) and A is the area of the ring. For the ring:
l=2πr,A=dr⋅y
Thus,
R0=ρ(2πr)dr⋅y
The power loss dP in this small ring due to the induced current can be expressed as:
dP=ϵ2R0
Substituting the values of ϵ and R0:
dP=(πR2B0ωcos(ωt))2ρ(2πr)dr⋅y=π2R4B20ω2cos2(ωt)⋅dr⋅y2ρr
Now, we integrate dP from r=0 to r=R:
P=∫R0dP=∫R0π2R4B20ω2cos2(ωt)⋅dr⋅y2ρr
This integral evaluates to:
P=π2B20ω2y2ρ∫R0R4cos2(ωt)⋅dr
To find the average power over one cycle, we use the average value of cos2(ωt), which is 12:
⟨P⟩=π2B20ω2yR44ρ
Thus, the total average power loss in the metal disc is given by:
⟨P⟩=π3B20f2R4y4ρ
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - SOLVED PROBLEMS ( OBJECTIVE)15videos
ELECTROMAGNETIC INDUCTION
- BOOK - FIITJEECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Comprehension Based Type Comprehension -I3videos
ELECTROMAGNETIC INDUCTION
- BOOK - FIITJEECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE9videos
ELECTROMAGNETIC INDUCTION
- BOOK - FIITJEECHAPTER - ELASTICITY AND WAVES EXERCISE - Assignment Problems (Objective) Level-II15videos
ELASTICITY AND WAVES
- BOOK - FIITJEECHAPTER - ELECTROMAGNETIC INDUCTION AND AC CURRENTEXERCISE - Example15videos
ELECTROMAGNETIC INDUCTION AND AC CURRENT
Similar Questions
A circular brass loop of radius a and resistance R is placed with it plane perpendicular to a magnetic field, which varies with time as B=B0sinωt. Obtain the expression for the induced current in the loop.
View SolutionA metal disc of radius R rotates with an angular velcoity ω about an axis perpendicular to its plane passing through its centre in a magnetic field B acting perpendicular to the plane of the disc. Calculate the induced emf between the rim and the axis of the disc.
View Solution
Knowledge Check
- Question 1 - Select One
A metallic ring of radius a and resistance R is held fixed with its axis along a spatially uniform magnetic field whose magnitude is B0 sin (ωt). Neglect gravity. Then,
Athe current in the ring oscillates with a frequency of 2ω .Bthe joule hearting loss in the ring is proportional to a2,Cthe force per unit length on the ring will be proportional to B20.Dthe net force on the ring is non-zero - Question 2 - Select One
A metal ring of radius r=0.5m with its plane noraml a unifrom magnetic field B of induction 0.2T carries a current I=100A The tension in newtons develoed in the ring is
A100B50C25D10 - Question 3 - Select One
A particle excuting SHM has equation y=Asin(ωt+π6) where ω=2πT, then find the possible time t when it is at y=−A2
AT2B5T6C3T2D11T6
On a smooth horizontal table a disc is placed with non conducting ring with uniformly distributed charge q fixed on its circumference. Both disc and ring are of mass m and radius r. If a uniform magnetic field of induction B which is symmetric with centre of disc is switched on in vertically downward direction, find the angular speed attained by disc due to this.
View SolutionA circular disc of radius 20 cm is rotating with a constant angular speed of 2.0rad/s in a uniform magnetic field of 0.2 T. Find the e.m.f. induced between the centre and rim of the disc. Given magnetic field is along the axis of rotation of disc.
View SolutionA metal disc of radius 200 cm is rotated at a constant angular speed of rads−1 in a plane at right angles to an external field of magnetic induction 0.05Wbm−2. Find the e.m.f. induced between the centre and a point on the rim.
View SolutionA circular disc of radius 0.2m is placed in a uniform magnetic fied of induction 1π(Wbm2)
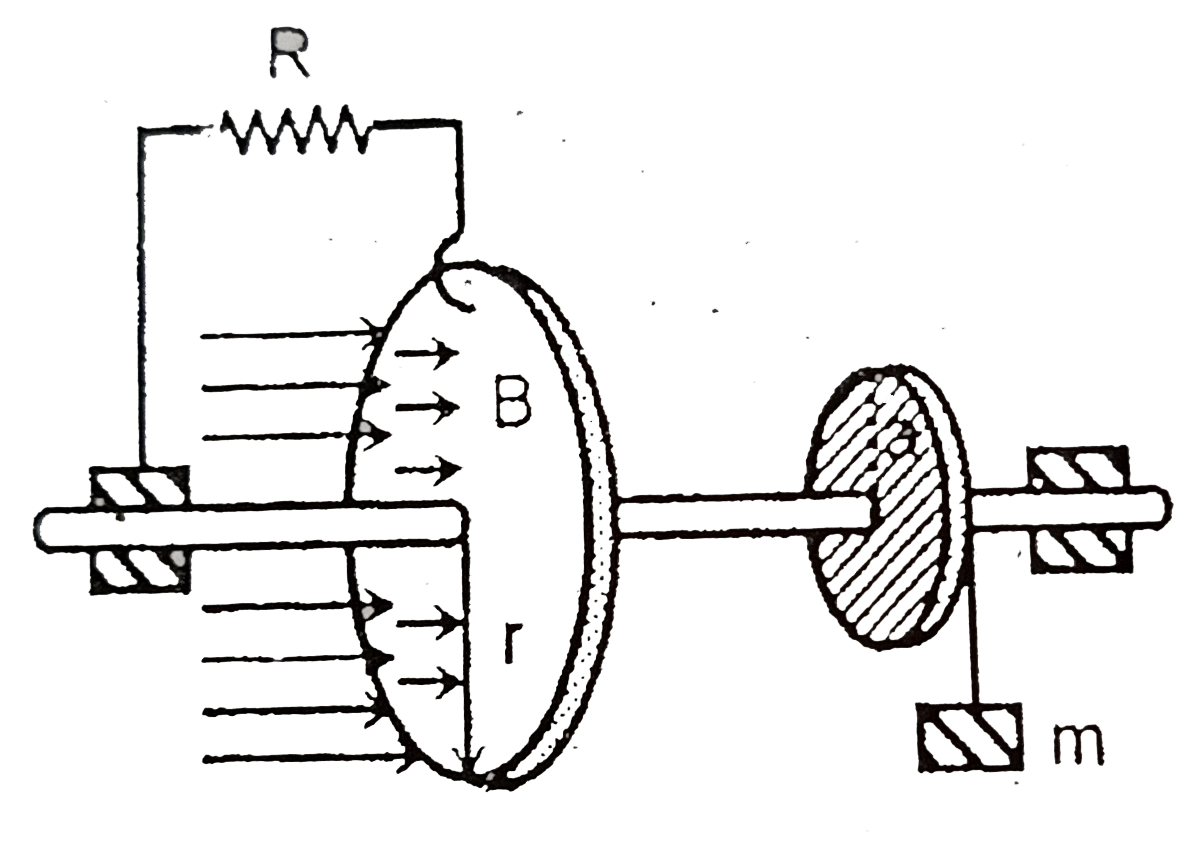
in such a way that its axis makes an angle of 60∘ with The magnetic flux linked with the disc isView SolutionA metallic disc of radius r is made of a material of negligible resistance and can rotate about a conducting horizontal shaft. A smaller non conducting disc of radius a is fixed onto the same shaft and has a massless cord wrapped around it, which is attached to a small object of mass m as shown. Two ends of a resistor of resistance R are connected to the perimeter of the disc and to the shaft by sliding contacts. The system is then placed into a uniform horizontal magnetic field B and the mass m is released. Find the terminal angular velocity with which the disc will rotate finally.
(Take r=10cm, a=2cm, R=1100Ω, B=0.2T, m=50gm, g=10m/s2)
 View Solution
View Solution

