A cylindrical vessel containing liquid is placed on the floor of an elevator. When the elevator is mad to acceleration equal to g, disscuss how the centre of gravity of the system containg vessel and liquid changes. Also, discuss how the centre of gravity of the system containing vessel and liquid changes when the elevator accelerates in the downward direction. Also discuss how the 'weight' would vary in each case.
(i) Please consider the factors that effect the centre of gravity of a regular and irregular bodies
(ii) Consider the point as centre of gravity (G) of a body is affected, when elevator accelerates in upwards direction and downward direction
Topper's Solved these Questions
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GRAVITATIONEXERCISE - LEVEL-310videos
GRAVITATION
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GRAVITATIONEXERCISE - LEVEL-144videos
GRAVITATION
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - ElectricityEXERCISE - Level 310videos
Electricity
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - HEATEXERCISE - All Questions115videos
HEAT
Similar Questions
An ideal gas of molar mass M is contained in a very tall vertical cylindrical vessel in the uniform gravitational field in which the free-fall acceleration equals to T, find the height at which the centre of gravity of the gas is located.
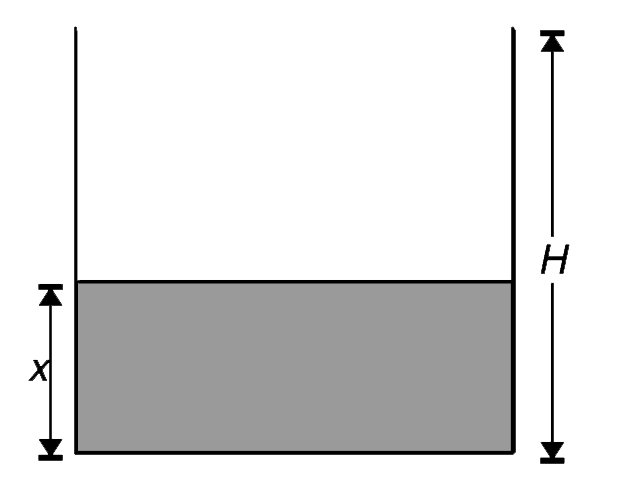
View SolutionA cylindrical container has mass M and height H. the centre of mass of the empty container is at height H2 from the base. A liquid, when completley filled in the container, has mass M2 this liquid is poured in the empty contaienr.
(a) How does the centre of mass of the system (container+liquid) move as the height (x) of liquid column changes from zero to H? Explain your answer qualitatively. Draw a graph showing the variation of height of centre of mass of the system (xcm) with x.
(b) Find the height of liquid column x for which the centre of mass is at its lowest position.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One or More
Le Chatelier's Principle
If a system at equilibrium is subjected to a change of any one of the factors such as concentration, pressure or temperature, the system adjusts itself in such a way as to nullify the effect of that change.
Change of pressure : If a system consists of gases, then the concentration of all the components can be alterd by changing the pressure. To increase the pressure on the system,the volume has to be decreased proportionally. The total number of mols per unit volume will now be more and the equilibrium will shift in the direction in which there is a decrease in number of moles i,e. towards the direction in which there can be decrease in pressure.
Effect of pressure on melting point : There are two types of solids:
(a) Solids whose volume decreases on melting e.g., ice,diamond carborundum magnesium nitride and quratz.
Solids (higher volume)⇔Liquid (lower volume) The process of melting is facilitated at high pressure, thus, melting point is lowerd.
(b) Solids whose volume increase on melting e.g.,Fe,Cu,Ag,Au,etc.
Solid (lower volume)⇔ Liquid (higher volume) In this case the process of melting become difficult at high pressure, thus melting point becomes high.
(c) Solubility of substances : When solid substances are dissolved in water, either heat is evolved (exothermic) or heat is absorbed (endothermic).
KCI+aq⇔KCI(aq)−heat
In such cases, solubility increase with increase in temperature. Consider the case of KOH, when this is dissolved,heat is evolved.
KOH+aq⇔KOH(aq)+heat
In such cases, solubility decrease with increase in temperature.
(d) Solubility of gases in liquids : When a gas dissolves in liquid,there is decrease in volume. Thus increase of pressure will favour the dissolution of gas in liquid.
For the reaction 12N2(g)+12O2(g)⇔NO(g)
If pressure is increased by reducing the volume of the container then:Atotal pressure at equilibrium will changeBconcentration of all the component at equilibrium will change .Concentration of all the component at equilibrium will remain change.Dequilibrium will shift in the forward direction.
An elecator is going up with an upward acceleration of 1m/s2. At the instant when its velocity is 2m/s, a stone is projected upward from its floor with a speed of 2m/s relative to the elevator, at an elevation of 30∘.
(a) Calculate the time taken by the stone to return to the floor.
(b) Sketch the path of the projectile as observed by an observer outside the elevator.
(c) If the elevator was moving with a downward acceleration equal to g, how would the motion be altered?View SolutionAn elecator is going up with an upward acceleration of 1m/s2. At the instant when its velocity is 2m/s, a stone is projected upward from its floor with a speed of 2m/s relative to the elevator, at an elevation of 30∘.
(a) Calculate the time taken by the stone to return to the floor.
(b) Sketch the path of the projectile as observed by an observer outside the elevator.
(c) If the elevator was moving with a downward acceleration equal to g, how would the motion be altered?View SolutionOn the basis of change of density with the change in temperature, explain how do the liquids get heated up, when heated in a vessel.
View SolutionTwo identical cylindrical vessel with their bases at the same level each contain a liquid of density ρ. The height of the liquid in one vessel is h1 and in the other is h2 the area of either base is A. What is the work done by gravity is equalising the levels when the two vessels are connected?
View SolutionTwo identical cylindrical vessels with their bases at the same level each, contain a liquid of density ρ. The area of either base is A but in one vessel the liquid heigth is h1 and in the other liquid height is h2(h2<h1). If the two vessel are connected, the work done by gravity in equalizing the level is
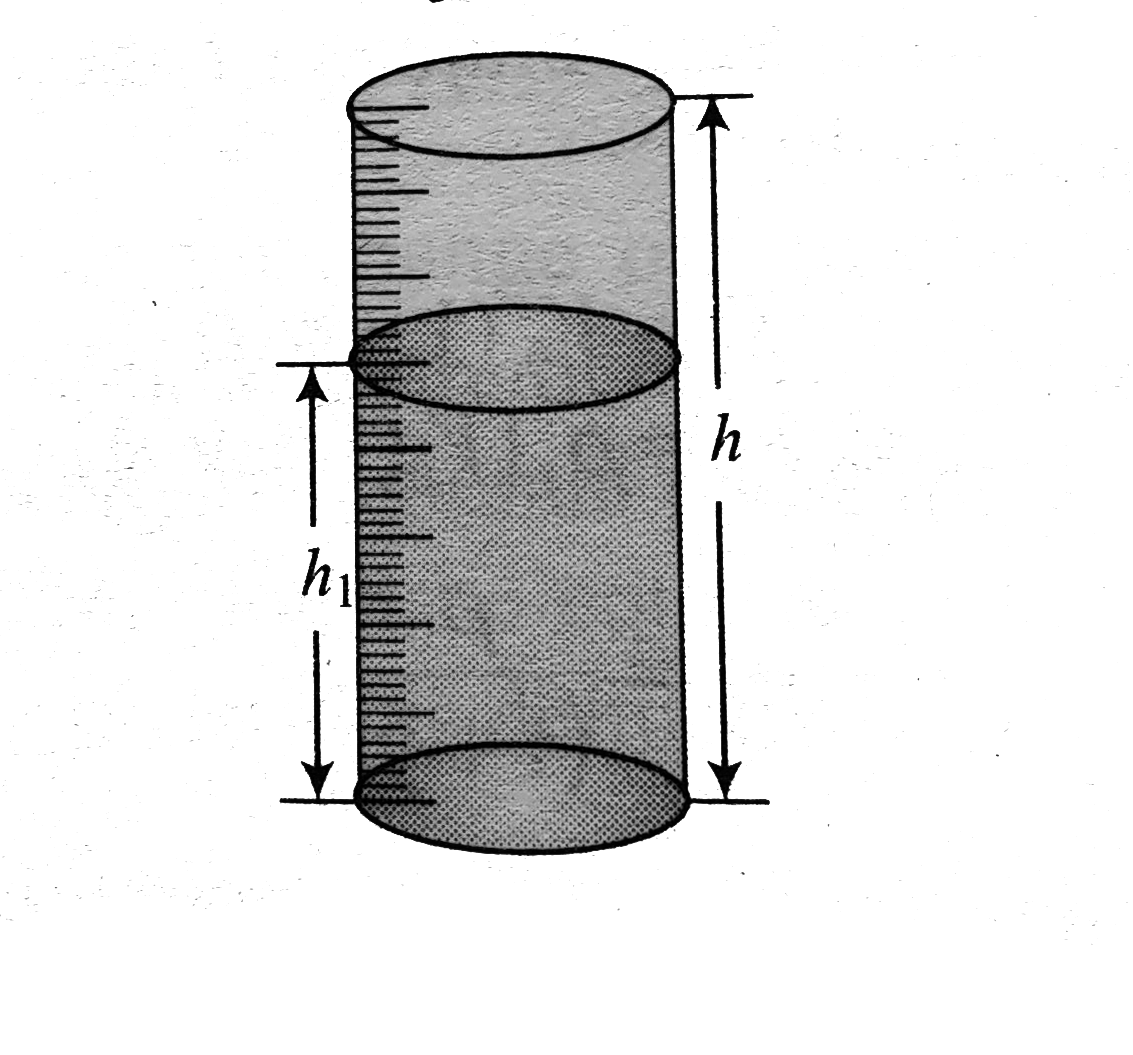
View SolutionConsider a cylindrical container of cross-section area A length h and having coefficient of linear expansion αc. The container is filled by liquid of real expansion coefficient γL up to height h1. When temperature of the system is increased by Δθ then
(a). Find out the height, area and volume of cylindrical container and new volume of liquid.
(b). Find the height of liquid level when expansion of container is neglected.
(c). Find the relation between γL and αc for which volume of container above the liquid level
(i) increases
(ii). decreases
(iii). remains constant.
(d). On the surface of a cylindrical container a scale is attached for the measurement of level of liquid of liquid filled inside it. If we increase the temperature of the temperature of the system by Δθ, then
(i). Find height of liquid level as shown by the scale on the vessel. Neglect expansion of liquid.
(ii). Find the height of liquid level as shown by the scale on the vessel. Neglect expansion of container.
 View Solution
View SolutionConsider a cylindrical container of cross-section area A length h and having coefficient of linear expansion αc. The container is filled by liquid of real expansion coefficient γL up to height h1. When temperature of the system is increased by Δθ then
(a). Find out the height, area and volume of cylindrical container and new volume of liquid.
(b). Find the height of liquid level when expansion of container is neglected.
(c). Find the relation between γL and αc for which volume of container above the liquid level
(i) increases
(ii). decreases
(iii). remains constant.
(d). On the surface of a cylindrical container a scale is attached for the measurement of level of liquid of liquid filled inside it. If we increase the temperature of the temperature of the system by Δθ, then
(i). Find height of liquid level as shown by the scale on the vessel. Neglect expansion of liquid.
(ii). Find the height of liquid level as shown by the scale on the vessel. Neglect expansion of container.
 View Solution
View Solution
