Doubtnut Promotions Sticky

The area of the region A={(x,y),0≤y≤x|x|+1and−1≤x≤1} in sq. units, is

A

2

B

43

C

13

D

23

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:A

We have,
A={(x,y):0≤y≤x|x|+1and−1≤x≤1}
When x≥0, then 0≤y≤x2+1
and when x<0, then 0≤y≤−x2+1
Now, the required region is the shaded region.
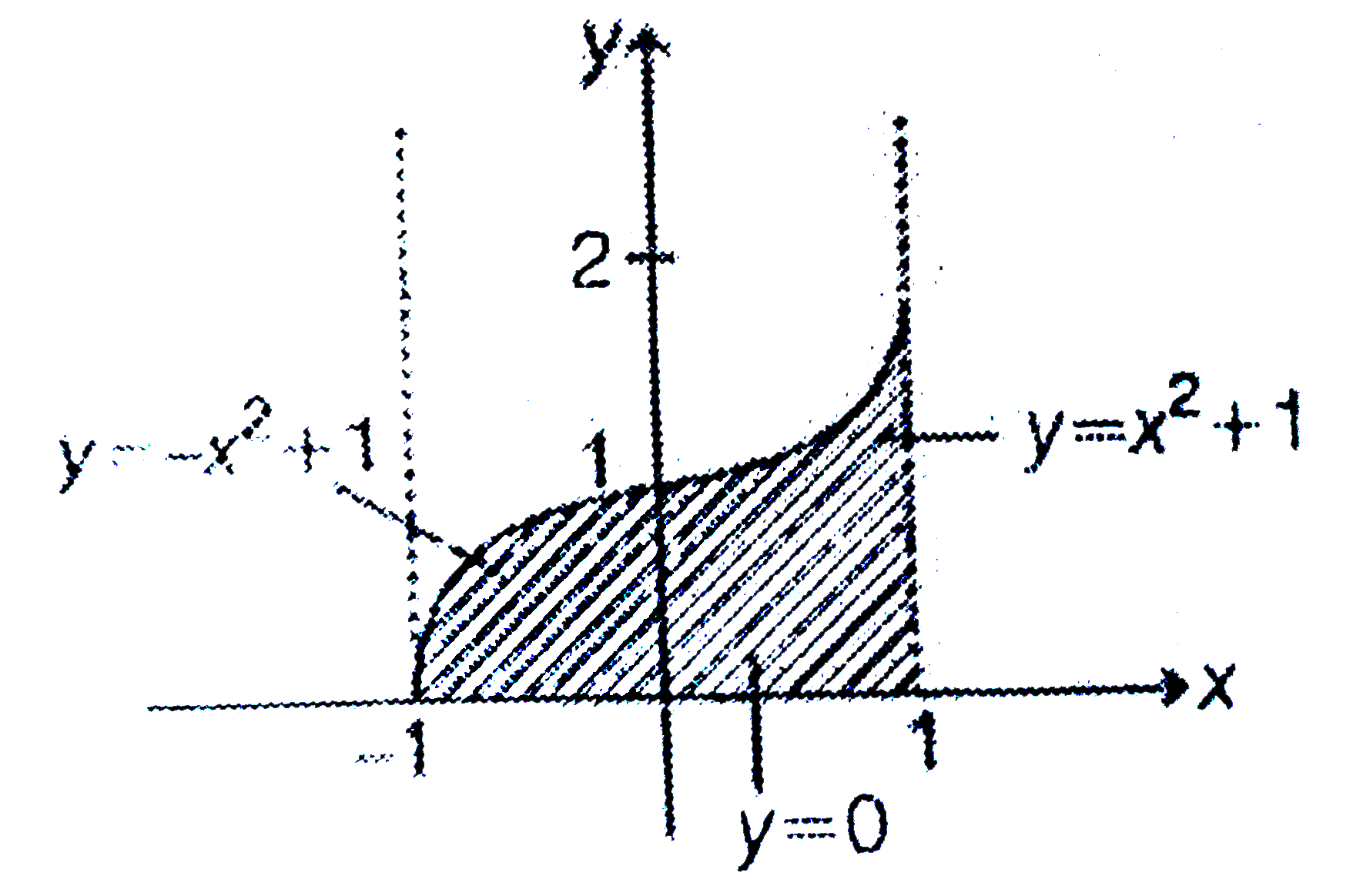
ocr_image
[∵y=x2+1⇒x2=(y−1), parabola with vertex (0, 1) and
y=−x2+1⇒x2=−(y−1),
Parabola with vertex (0, 1) but open downward ]
We need to calculate the shaded area, which is equal to
∫0−1(−x2+1)dx+∫10(x2+1)dx
=[−x33+x]0−1+[x33+x]10
=(0−[−(−1)33+(−1)])+([13+1]−0)
=−(13−1)+43
=23+43=2

|