The velocity-time graph for the vertical component of the velocity of a body thrown upwards from the ground and landing on the roof of a building is given in the figure. The height of the building is

50m
40m
20m
30m
The correct Answer is:B
Height= algebraic sum of the area under velocity time graph
Topper's Solved these Questions
- BOOK - DISHA PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Exercise-170videos
MOTION IN A STRAIGHT LINE
- BOOK - DISHA PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Exercise -2 : CONCEPT APPLICATOR28videos
MOTION IN A PLANE
- BOOK - DISHA PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - EXERCISE - 2 : Concept Applicator25videos
MOVING CHARGES AND MAGNETISM
Similar Questions
Knowledge Check
- Question 1 - Select One
The velocity time graph for the veticaly component of the velocity of a body thrown upwards from the ground and landing on the roof of a building is given in the figure.The height of the building is:
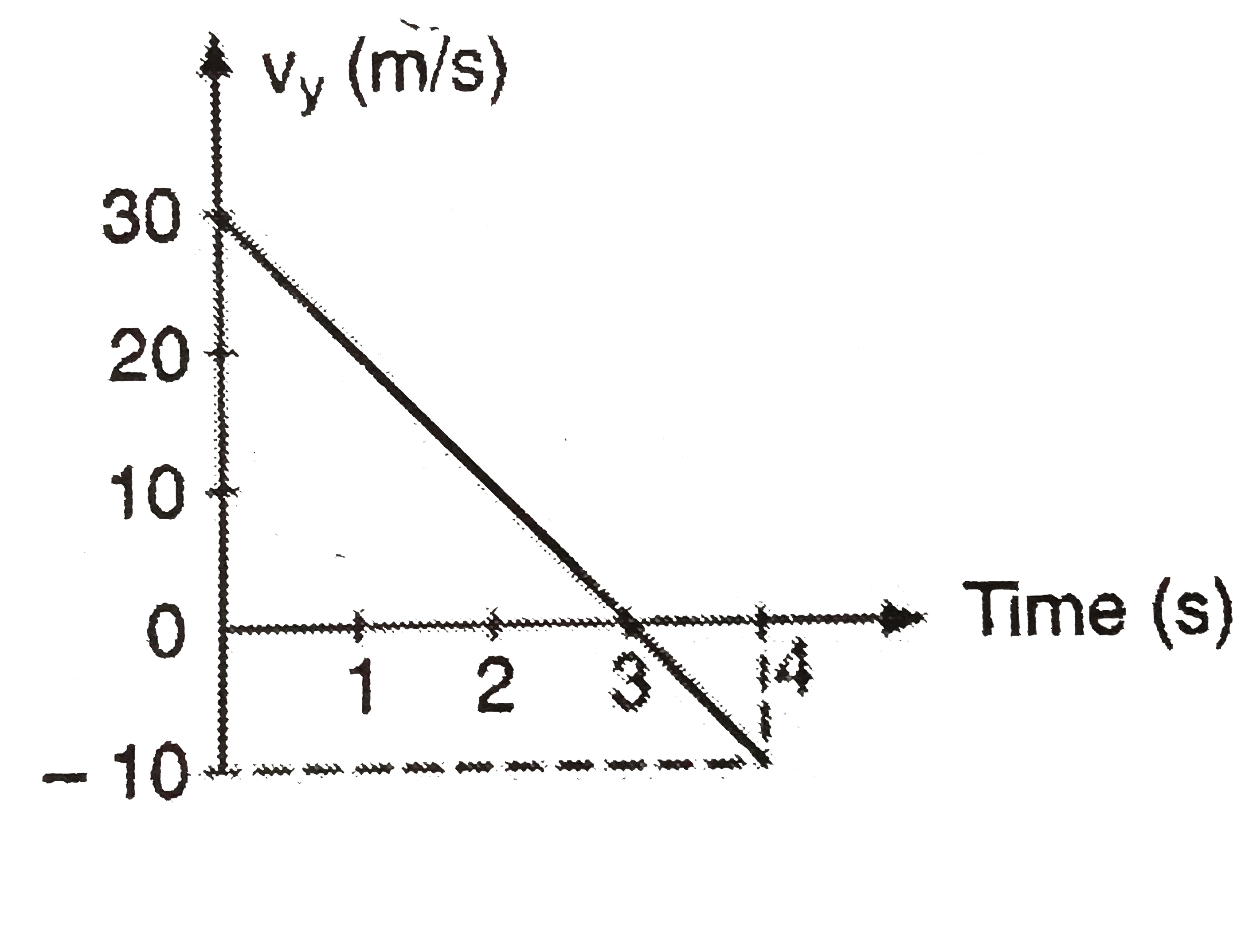 A50 mB40 mC20 mD30 m
A50 mB40 mC20 mD30 m - Question 2 - Select One
Velocity-time graph of a body thrown vertically up is
Aa straight lineBa parabolaCa hyperbolaDcircle - Question 3 - Select One
A stone is thrown downwards with velocity 10 m/s from the roof of a builng and it reaches the ground with speed 40 m/s. the height of the building would be
A75 mB100 mC125 mD150 m
A stone is thrown vertically upwards from the top of a building, with a speed of 14.7m/s. if it returns to the earth in 8s, calculate the height of the building.
View SolutionWhat is the area under the velocity-time curve in the case of a body projected vertically upwards from the ground after reaching the ground ?
View SolutionDraw a velocity versus time graph of a stone thrown vertically upwards and then coming downwards after attaining the maximum height .
View SolutionIf a ball is thrown up with a velocity v from the top of a building the ball reaches the ground with a velocity 3u. Find the height of the building.
View Solution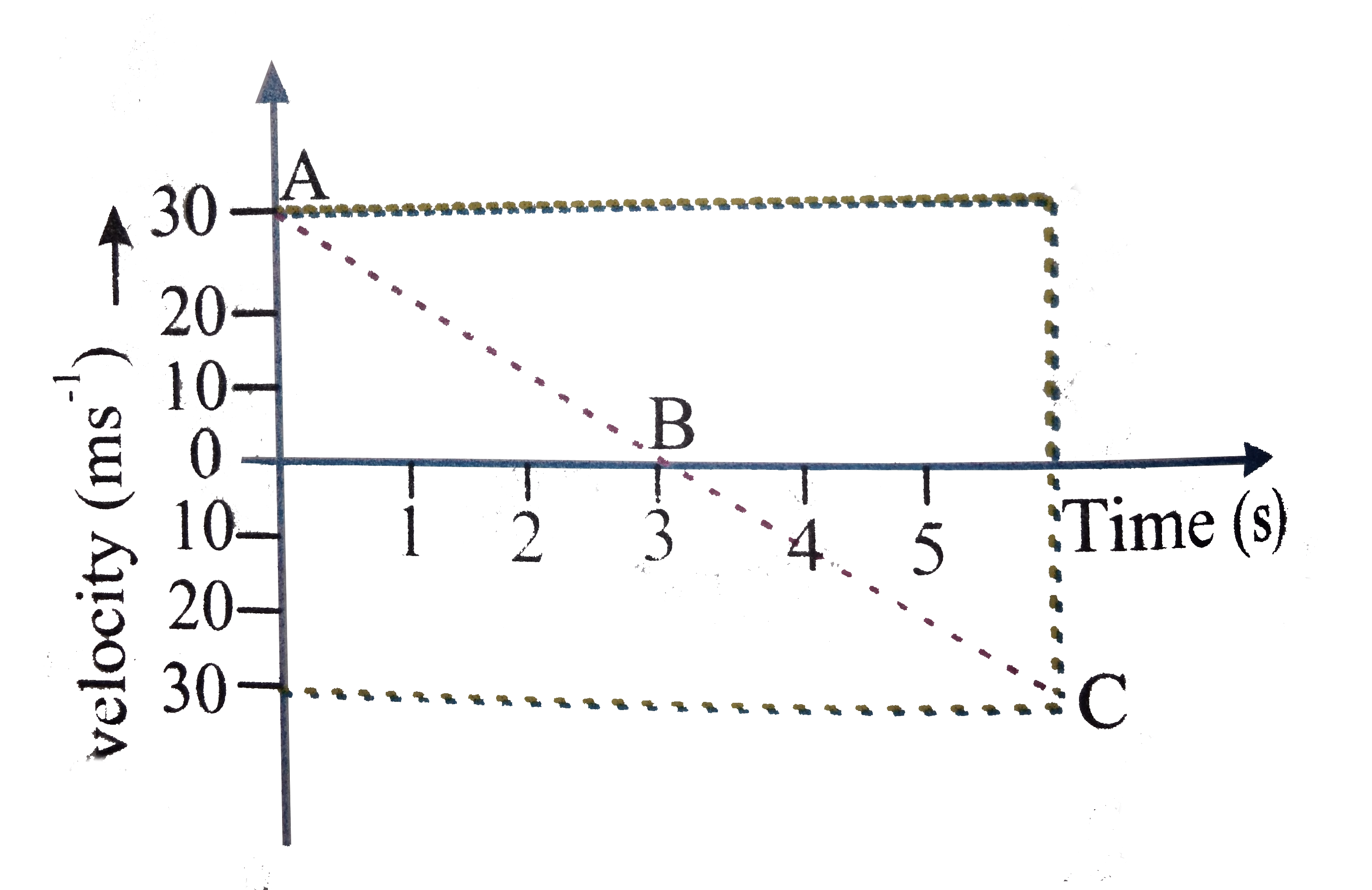
The velocity-time graph of a stone thrown vertically upward with an initial velocity of 30ms−1 is shown in the figure. The velocity in the upward direction is taken as positive and that in the downward direction as negative. What is the maximum height to which the stone rises?View Solution

