On P-V coordinates, the slope of an isothermal curve of a gas at a pressure P = IMPa and volume V= 0.0025 m3 is equal to −400Mpa/m3. If Cp/Cv=1.4 , the slope of the adiabatic curve passing through this point is :
−56Mpa/m3
−400Mpa/m3
−560Mpa/m3
None of these
The correct Answer is:C
To find the slope of the adiabatic curve passing through the given point on the P-V diagram, we can use the relationship between the slopes of the isothermal and adiabatic curves.
1. Identify the Given Values:
- Slope of the isothermal curve (m_isothermal) = -400 MPa/m³
- Value of CpCv (gamma, γ) = 1.4
2. Use the Relationship Between Slopes:
The slope of the adiabatic curve (m_adiabatic) is related to the slope of the isothermal curve by the formula:
madiabatic=γ×misothermal
3. Substitute the Known Values:
Substitute the values we have into the equation:
madiabatic=1.4×(−400MPa/m3)
4. Calculate the Slope of the Adiabatic Curve:
madiabatic=−560MPa/m3
5. Conclusion:
The slope of the adiabatic curve passing through the point where P=1MPa and V=0.0025m3 is:
madiabatic=−560MPa/m3
- Slope of the isothermal curve (m_isothermal) = -400 MPa/m³
- Value of CpCv (gamma, γ) = 1.4
The slope of the adiabatic curve (m_adiabatic) is related to the slope of the isothermal curve by the formula:
madiabatic=γ×misothermal
Substitute the values we have into the equation:
madiabatic=1.4×(−400MPa/m3)
madiabatic=−560MPa/m3
The slope of the adiabatic curve passing through the point where P=1MPa and V=0.0025m3 is:
madiabatic=−560MPa/m3
Topper's Solved these Questions
- THERMODYNAMICS BOOK - DISHA PUBLICATIONCHAPTER - THERMODYNAMICS EXERCISE - EXERCISE-1 : CONCEPT BUILDER (Topic-3 CARNOT ENGINE, REFIGERATOR AND SECOND LAW OF THERMODYNAMICS)
15 Videos
- THERMAL PROPERTIES OF MATTER BOOK - DISHA PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTER EXERCISE - Exercise-2 (Concept Applicator)
28 Videos
- WAVE OPTICSBOOK - DISHA PUBLICATIONCHAPTER - WAVE OPTICSEXERCISE - EXERCISE-2 : CONCEPT APPLICATOR
30 Videos
Similar Questions
Knowledge Check
On P-V coordinates, the slope of an isothermal curve of a gas at a pressure P = IMPa and volume V= 0.0025 m3 is equal to −400Mpa/m3. If Cp/Cv=1.4 , the slope of the adiabatic curve passing through this point is :
A−56Mpa/m3B−400Mpa/m3C−560Mpa/m3DNone of theseFor a p-V plot, the slope of an adiabatic curve = x × slope of isothermal at the same point. Here x is :
ARλ−1B1λCλD1λ−1Assertiobn : Ratio of isothermal bulk modulus and adiabatic bulk modulus for a monoatomic gas at a given pressure is 35.
This ratio is equal to γ=CpCv.AIf both Assertion and Reason are true and the Reason is correctn explanation of the Assertion.BIf both Assertion and Reason are true but Reason is not the correct explanation of Assertion.CIf Assertion is true, but the Reason is false.DIf Assertion is false but the Reason is true.
Graph the line passing through the point P and having slope m.
P=(2,4),m=−3
View SolutionConider a given sample of an ideal gas (CpCv=γ) having initial pressure (p0)andvolume(V0) . (a) The gas is isothermally taken to a pressure (P02) and from there adiabatically ta a pressure (p02)andomthereisothermally→apressure(p_0 / 4 ) . find the volume.
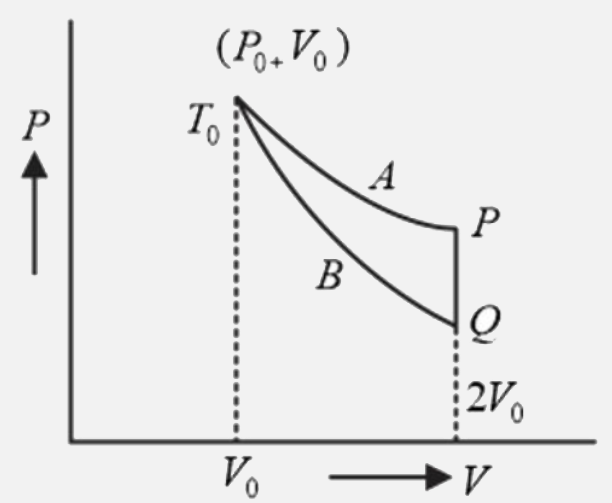
View SolutionAn ideal gas (1 mol ,monatomic) is in the initial state P (see diagram) on an isothermal curve A at a temperature T0 . It is brought under a constant volume (2V0) process to Q which lies on an adiabatic curve B intersecting the isothermal curve A at (P0,V0,T0) . The change in the internal energy of the gas (in terms of T0 ) during the process is (22/3=1.587)
View SolutionA gas may expand either adiabatically or isothermally. A number of p−V curves are drawn for the two processes over different ranges of pressure and volume, it will be found that
(i) Two adiabatic curves do not intersect
(ii) two isothermal curves do not intersect
(iii) an adiabatic curve and an isothermal curve may intersect.
(iv) the magnitude of the slope of an adiabatic curve is greater thanthe magnitude of the slope of an isothermal curve
View SolutionDuring an adiabatic process , the pressure p of a fixed mass of an ideal gas change by Δpand its volume V change by ΔV . If γ=Cp/Cv then ΔV/V is given by
View Solution
