Five particles undergo damped harmonic motion. Value for the spring constant k, the damping constantb, ad the mass m are given below. Which leads to the smallest rate of loss of mechanical energy?
k=100N/m, m=50g, b= 8g m/s
k= 150N/m, m=50g, b= 5gm/s
k=150N/m, m=10g,b= 8gm/s
k=200N/m, m=8g, b=6gm/s
The correct Answer is:B
Topper's Solved these Questions
- OSCILLATIONSBOOK - RESNICK AND HALLIDAYCHAPTER - OSCILLATIONSEXERCISE - Problems
39 Videos
- MOTION IN TWO AND THREE DIMENSIONSBOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PRACTICE QUESTIONS (MATRIX-MATCH)
10 Videos
- PHOTONS AND MATTER WAVESBOOK - RESNICK AND HALLIDAYCHAPTER - PHOTONS AND MATTER WAVESEXERCISE - PRACTICE QUESTIONS(Integer Type)
4 Videos
Similar Questions
Here are three sets of values for the spring constant, damping constant, and mass for the damped oscillator of Fig. 15.17. Rank the sets according to the time required for the mechanical energy to decrease to one-fourth of its initial value, greatest first.
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯Set 12k0 b0 m0Set 2 k0 6b0 4m0Set 33k0 3b0 m0–––––––––––––––––––––
View SolutionA particle of mass 200g executes a simple harmonic motion. The restoring force is provided by a spring of spring constant 80N/m. Find the time period.
View Solution
Knowledge Check
A particle undergoes damped harmonic motion. The spring constant is 100N/m, the damping constant is 8.0×10−3kgm/s, and the mass is 0.50kg. If the particle starts at its maximum displacement, x= 1.5m at time t=0, what is the particle's position at t= 5.0s?
A−1.5mB−0.73mC0mD0.75mWhich of the below figure (s) represent damped simple harmonic motion.
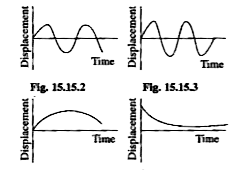
Select the correct answer using the codes given belowAFig. 1 aloneBFig. 2 aloneCFig. 4 aloneDFig. 3 and 4In damped oscillation mass is 1 kg and spring constant = 100 N/m, damping coefficient = 0.5 kg s–1. If the mass is displaced by 10 cm from its mean position that what will be the value of its mechanical energy after 4 seconds ?
A0.67 JB0.067 JC6.7 JD0.5 J
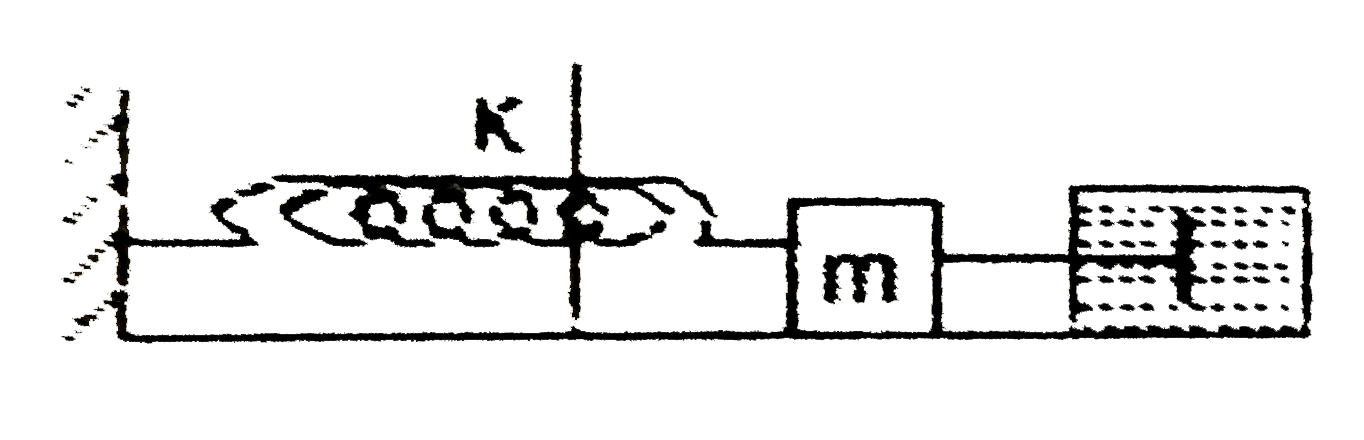
For a damped oscillator which follow the equation →F=−k→x−b→V
the mass of the block is 100 gm K=100N/M and the damping constant is 20 gm/sec. thhen find the time taken for its mechanical energy to drop to one-fourth of its initial value.
View SolutionDerive an experession for the period of oscillation of a mass attached to a spring executing simple harmonic motion. Given that the period of oscillation depends on the mass m and the force constant k, where k is the force required to stretch the spring by unit length?
View Solution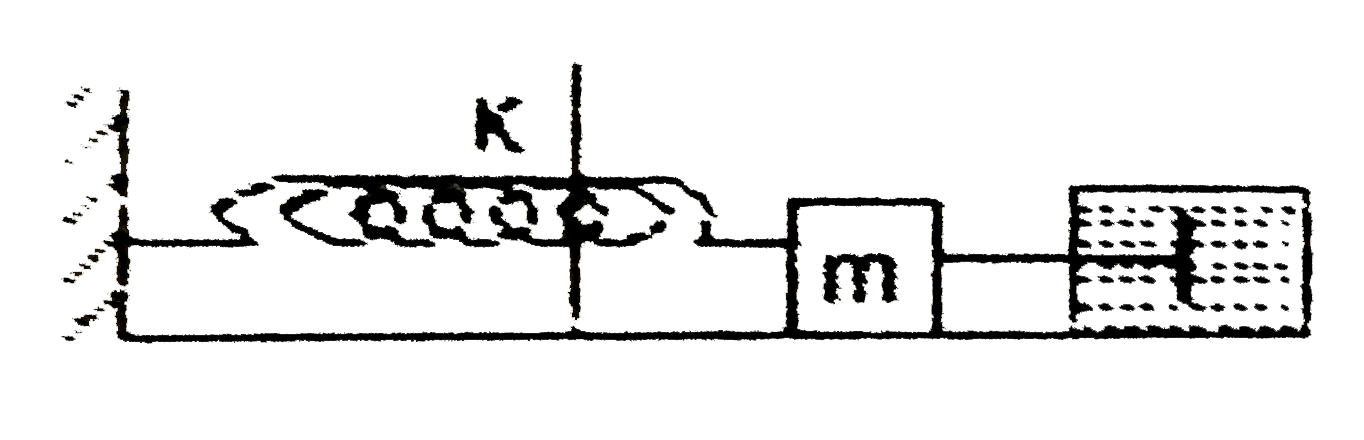
For a damped oscillator which follow the equation →F=−k→x−b→V
the mass of the block is 100 gm K=100N/M and the damping constant is 20 gm/sec. thhen find the time taken for its mechanical energy to drop to one-fourth of its initial value.
View SolutionA particle of mass m is hanging vertically by an ideal spring of force constant K . If the mass is made to oscillate vertically, its total energy is
View SolutionA particle of mass m is hanging vertically by an ideal spring of force constant K . If the mass is made to oscillate vertically, its total energy is
View Solution
