Consider two concentric shells shown in Fig. 24-27, one of charge q and radius R and another outer shell of charge −q and radius 2R. Find the potential at the surface of the outer shell and at the center.

Remember that both the shells will contribute to the potential at any point. Center is a point which is inside both the shells. It can be said that net potential at any point is
V=V1+V2.
where V1andV2 are potential due to inner and outer shells, respectively.
Determining potential: The potential at the center due to the shell is given by Eq. 24-39.
V1=kqR,V2=−kq2R.
Therefore,
V=kqR+−kq2R=kq2R.
The surface of the outer shell is a point lying outside both the shells. So, at that point the potential will not be constant but will be given by the equation
V=kq2R+−kq2R=0.
Thus, we find that the potential everywhere out of the system of shells is zero. This can be explained on the basis of Gauss. law, the net charge in any Gaussian surface drawn outside the shells is zero. So, the electric field outside is zero. According to Eq. 24-19, the potential outside the shell is constant. But at an outside point, that is infinitely, the electric potential is already defined to be zero. So, the potential at all the points outside must be zero.
Topper's Solved these Questions
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - SAMPLE PROBLEM 24.14 Field and potential inside a charged spherical shell
1 Videos
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - SAMPLE PROBLEM 24.15 Point charge projected from a large distance towards center of fixed charged ring
1 Videos
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - SAMPLE PROBLEM 24.12 Potential due to infinite sheet of charge
1 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Questions (Integer Type )
3 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PRACTICE QUESTIONS (Integer Type)
14 Videos
Similar Questions
Consider two concentric spherical metal shells of radii r1 and r2(r2>r1). If the outer shell has a charge q and the inner one is grounded, then the charge on the inner shell is
View SolutionThree concentric conducting sphereical shells carry charges +4Q on the inner shell −2Q on the middle shel and +6Q on the outer shell. The charge on the inner surface of the outer shell is
View Solution
Knowledge Check
Consider two concentric spherical metal shells of radii r1 and r2(r2>r1). If the outer shell has a charge q and the inner one is grounded, then the charge on the inner shell is
A−r2r1qBzeroC−r1r2qD−qThree concentric conducting spherical shells carry charges +4Q on the inner shell −2Q on the middle shell and +6Q on the outer shell. The charge on the inner surface of the outer shell is
A0B4QC−QD−2QThree concentric conducting spherical shells carry charges as : +4Q on the inner shell, −2Q on the middle shell and −5Q on the outer shell. The charge on the inner surface of the outer shell is
A0B4QC−QD−2Q
In (Fig. 3.73), if the inner shell has charge +q0 and the outer shell is earthed, then
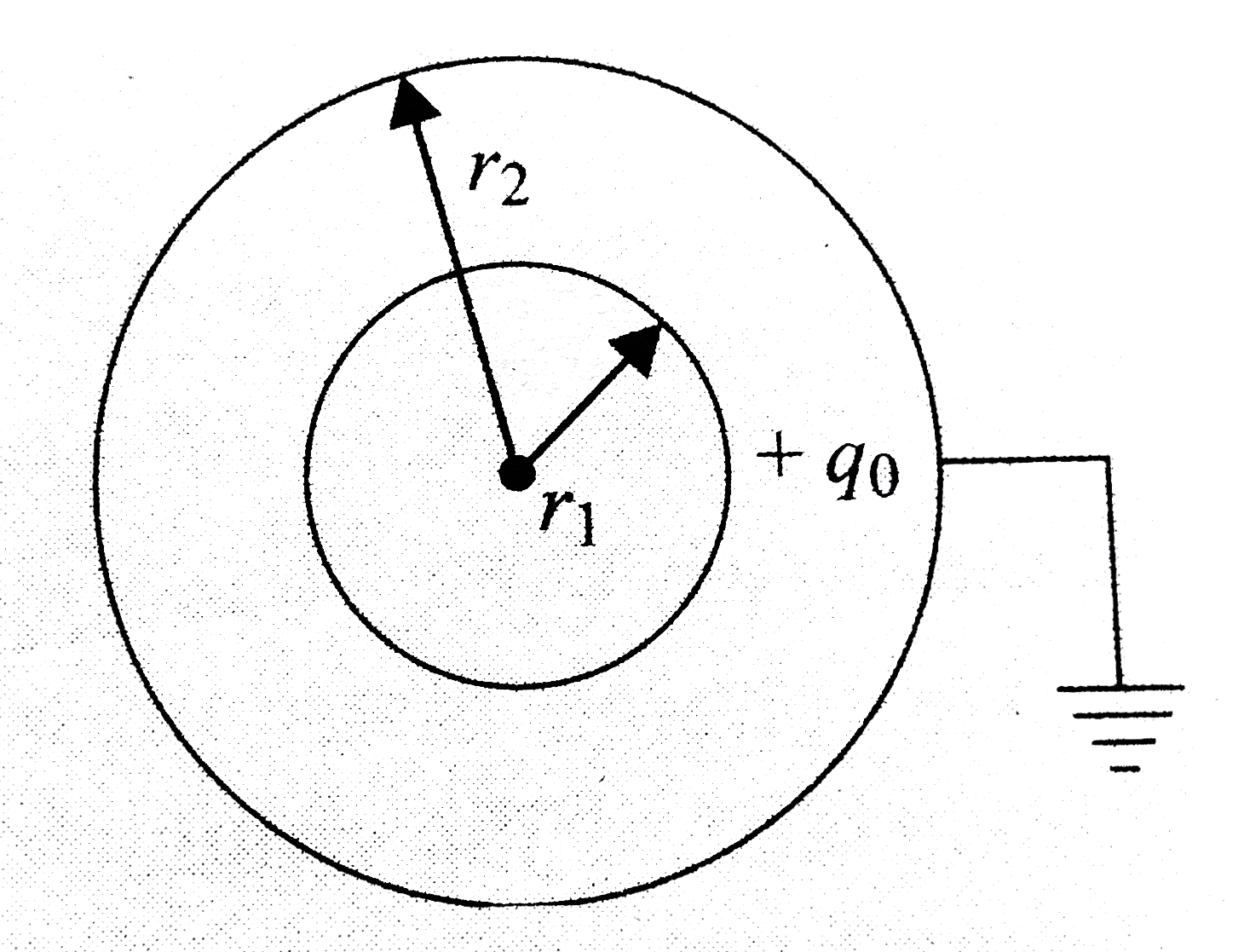 .
.
i. determine the charge on the outer shell, and
ii. find the potential of the inner shell.
View SolutionIn (Fig. 3.73), if the inner shell has charge +q0 and the outer shell is earthed, then
 .
.
i. determine the charge on the outer shell, and
ii. find the potential of the inner shell.
View SolutionConsider two concentric metalic shell's of radii R and 2R. The inner shell is having charge Q and outer shell is uncharged. If they are connected with a conducting wire. Then,
View SolutionConsider two concentric metalic shell's of radii R and 2R. The inner shell is having charge Q and outer shell is uncharged. If they are connected with a conducting wire. Then,
View SolutionThree concentric conducting spherical shells carry charges as : +4Q on the inner shell, −2Q on the middle shell and −5Q on the outer shell. The charge on the inner surface of the outer shell is
View Solution
