Consider two concentric metalic shell's of radii R and 2R. The inner shell is having charge Q and outer shell is uncharged. If they are connected with a conducting wire. Then,
Q amount of change will flow from inner to outer shell
Q/e number of electrons will flow from outer to inner shell, where 'e' is the charge on an electron
KQ24R amount of heat is produced in the process
KQ22R amount of heat is produced in the process
The correct Answer is:A, B, C
To solve the problem step by step, we will analyze the situation of two concentric metallic shells with given charges and radii.
Step 1: Understand the Setup
We have two concentric metallic shells:
- Inner shell with radius R and charge Q.
- Outer shell with radius 2R and initially uncharged (charge = 0).
Step 2: Connect the Shells with a Wire
When the two shells are connected by a conducting wire, they will reach the same electric potential because conductors in electrostatic equilibrium must have the same potential.
Step 3: Calculate the Potential of Each Shell
The potential V at the surface of a charged spherical shell is given by:
V=kQr
where k is Coulomb's constant, Q is the charge, and r is the radius.
- For the inner shell (radius R):
Vinner=kQR
- For the outer shell (radius 2R):
Vouter=kQouter2R
where Qouter is the charge on the outer shell after they are connected.
Step 4: Set the Potentials Equal
Since both shells are at the same potential after connection:
kQR=kQouter2R
Step 5: Simplify the Equation
Cancelling k and R from both sides:
Q=Qouter2
Thus, we can express Qouter in terms of Q:
Qouter=2Q
Step 6: Charge Conservation
Since the total charge must be conserved, the charge on the outer shell after connection must equal the initial charge on the inner shell:
Qouter+Qinner=Q+0
Substituting Qouter=2Q and Qinner=0:
2Q+0=Q
This indicates that the charge Q flows from the inner shell to the outer shell.
Step 7: Calculate the Number of Electrons Transferred
The number of electrons n that flow from the outer shell to the inner shell can be calculated using:
Q=n⋅e
where e is the charge of an electron. Rearranging gives:
n=Qe
Step 8: Calculate the Change in Potential Energy
The initial potential energy Ui of the system (with charge Q on the inner shell) is given by:
Ui=kQ22R
The final potential energy Uf (with charge 2Q on the outer shell) is:
Uf=k(2Q)22(2R)=2kQ22R=kQ2R
The change in potential energy ΔU is:
ΔU=Uf−Ui=kQ2R−kQ22R=kQ22R
Step 9: Determine the Heat Produced
The energy lost in the process will be dissipated as heat, which is equal to the change in potential energy:
Heat produced=−ΔU=−kQ22R
Summary of Results
1. Charge Q flows from the inner shell to the outer shell.
2. The number of electrons that flow from the outer shell to the inner shell is Qe.
3. The amount of heat produced in the process is −kQ22R.
We have two concentric metallic shells:
- Inner shell with radius R and charge Q.
- Outer shell with radius 2R and initially uncharged (charge = 0).
When the two shells are connected by a conducting wire, they will reach the same electric potential because conductors in electrostatic equilibrium must have the same potential.
The potential V at the surface of a charged spherical shell is given by:
V=kQr
where k is Coulomb's constant, Q is the charge, and r is the radius.
Vinner=kQR
Vouter=kQouter2R
where Qouter is the charge on the outer shell after they are connected.
Since both shells are at the same potential after connection:
kQR=kQouter2R
Cancelling k and R from both sides:
Q=Qouter2
Thus, we can express Qouter in terms of Q:
Qouter=2Q
Since the total charge must be conserved, the charge on the outer shell after connection must equal the initial charge on the inner shell:
Qouter+Qinner=Q+0
Substituting Qouter=2Q and Qinner=0:
2Q+0=Q
This indicates that the charge Q flows from the inner shell to the outer shell.
The number of electrons n that flow from the outer shell to the inner shell can be calculated using:
Q=n⋅e
where e is the charge of an electron. Rearranging gives:
n=Qe
The initial potential energy Ui of the system (with charge Q on the inner shell) is given by:
Ui=kQ22R
The final potential energy Uf (with charge 2Q on the outer shell) is:
Uf=k(2Q)22(2R)=2kQ22R=kQ2R
The change in potential energy ΔU is:
ΔU=Uf−Ui=kQ2R−kQ22R=kQ22R
The energy lost in the process will be dissipated as heat, which is equal to the change in potential energy:
Heat produced=−ΔU=−kQ22R
1. Charge Q flows from the inner shell to the outer shell.
2. The number of electrons that flow from the outer shell to the inner shell is Qe.
3. The amount of heat produced in the process is −kQ22R.
Similar Questions
there are two concentric metallic shells of radii R and 2R, if outer shell is given charge q1 , then charge on surface of inner shell will be
View SolutionTwo concentric conducting shells of radii R and 2R are having charges Q and -2Q respectively.In a region r
View SolutionIn the figure two concentric conducting shells of radius R & 2R are shown. The inner shell is charged with Q and the shell is unchanged. The amount of energy dissipated when the shells are connected by a conducting wire is:

View SolutionConsider two concentric spherical metal shells of radii 'a' and b>a .The outer shell has charge Q but the inner shell has no charge.Now the inner shell is grounded ,This means that the inner shell will come at zero potential and that electric fields lines leave the outer shell and end on the inner shell.(a) Find the charge on the inner shell
Find the potential on outer sphere.
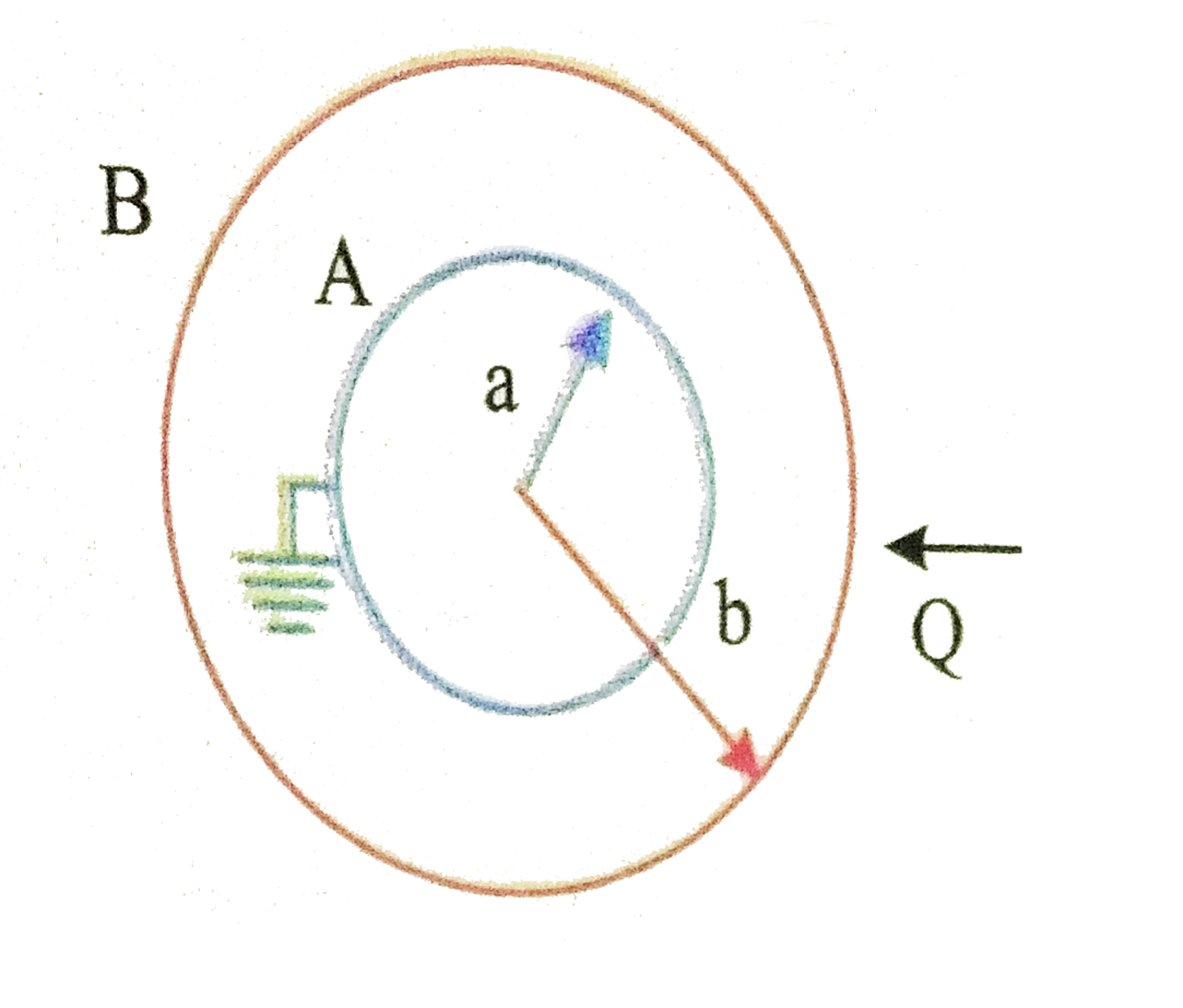
View SolutionTwo concentric spherical conducting shells of radii r and R (r<R) carry charges q and Q respectively . The two shells are now connected by a conducting wire. The final charge on the inner shell is
View SolutionTwo concentric spherical conducting shells of radii R and 2R are carrying charges q and 2q, respectively. Both are now connected by a conducting wire. Find the change in electric potential (inV) on the outer shell.
View SolutionConsider two concentric spherical metal shells of radii r1 and r2(r2>r1). If the outer shell has a charge q and the inner one is grounded, then the charge on the inner shell is
View SolutionFigure shows two conducting thin concentric shells of radii r and 3r. The outer shell carries charge q and the inner shell is neutral . The amount of charge that flows from the inner shell to the earth after the key K is closed is equal to (1/n)th of the charge on the outer shell. What is the value of n ?
 .
.
View SolutionThere are two concentric metal shells of radii r1 and r2(>r1). If initially, the outer shell has a charge q and the inner shell is having zero charge and then inner shell is grounded. Find :
(i) Charge on the inner surface of outer shell.
(ii) Final charges on each sphere.
(iii) Charge flown through wire in the ground.
View SolutionThere are two concentric conducting spherical shells of radii 10cm and 20cm. The outer shell is charged to a potential of 50esu while inner shell is earthed. The charge on outer shell is
View Solution
