A rifle that shoots bullets at 460 m/s is to be aimed at a traget 45.7 m away. If the center of the target is level with the rifle, how high above the target must the rifle barrel be pointed so that the bullet hits dead center?
To solve the problem of how high above the target a rifle must be aimed to hit the center of the target, we will use the principles of projectile motion. Here’s a step-by-step solution:
Step 1: Understand the Problem
The rifle shoots bullets at a speed of 460 m/s, and the target is 45.7 m away. The bullet will follow a parabolic trajectory due to gravity, so we need to find the angle at which to aim the rifle to ensure the bullet hits the target.
Step 2: Use the Range Formula
The range R of a projectile is given by the formula:
R=u2sin(2θ)g
Where:
- R is the range (45.7 m)
- u is the initial velocity (460 m/s)
- g is the acceleration due to gravity (approximately 10 m/s²)
- θ is the angle of projection
Step 3: Rearranging the Formula
We can rearrange the formula to find sin(2θ):
sin(2θ)=Rgu2
Substituting the known values:
sin(2θ)=45.7×104602
Step 4: Calculate sin(2θ)
Calculating the right side:
sin(2θ)=457211600≈0.00216
Step 5: Find 2θ
Now, we find 2θ by taking the inverse sine:
2θ=sin−1(0.00216)
Calculating this gives:
2θ≈0.00216 radians
Step 6: Find θ
Now, divide by 2 to find θ:
θ≈0.00108 radians
Step 7: Calculate the Height
Using the small angle approximation, where tan(θ)≈sin(θ)≈θ for small angles, we can find the height h using:
tan(θ)=hd
Where d is the horizontal distance (45.7 m). Thus:
h=d⋅tan(θ)≈d⋅θ
Substituting the values:
h=45.7⋅0.00108≈0.0494 m≈4.94 cm
Final Answer
The rifle must be aimed approximately 4.94 cm above the center of the target.
---
The rifle shoots bullets at a speed of 460 m/s, and the target is 45.7 m away. The bullet will follow a parabolic trajectory due to gravity, so we need to find the angle at which to aim the rifle to ensure the bullet hits the target.
The range R of a projectile is given by the formula:
R=u2sin(2θ)g
Where:
- R is the range (45.7 m)
- u is the initial velocity (460 m/s)
- g is the acceleration due to gravity (approximately 10 m/s²)
- θ is the angle of projection
We can rearrange the formula to find sin(2θ):
sin(2θ)=Rgu2
Substituting the known values:
sin(2θ)=45.7×104602
Calculating the right side:
sin(2θ)=457211600≈0.00216
Now, we find 2θ by taking the inverse sine:
2θ=sin−1(0.00216)
Calculating this gives:
2θ≈0.00216 radians
Now, divide by 2 to find θ:
θ≈0.00108 radians
Using the small angle approximation, where tan(θ)≈sin(θ)≈θ for small angles, we can find the height h using:
tan(θ)=hd
Where d is the horizontal distance (45.7 m). Thus:
h=d⋅tan(θ)≈d⋅θ
Substituting the values:
h=45.7⋅0.00108≈0.0494 m≈4.94 cm
The rifle must be aimed approximately 4.94 cm above the center of the target.
Topper's Solved these Questions
- MOTION IN TWO AND THREE DIMENSIONSBOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PRACTICE QUESTIONS (SINGLE CORRECT CHOICE TYPE)
49 Videos
- MOTION IN TWO AND THREE DIMENSIONSBOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)
3 Videos
- MOTION IN TWO AND THREE DIMENSIONSBOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - CHECKPOINT
3 Videos
- MOTION ALONG A STRAIGHT LINEBOOK - RESNICK AND HALLIDAYCHAPTER - MOTION ALONG A STRAIGHT LINEEXERCISE - PRACTICE QUESTIONS ( Integer Type )
3 Videos
- OSCILLATIONSBOOK - RESNICK AND HALLIDAYCHAPTER - OSCILLATIONSEXERCISE - Practice Questions
57 Videos
Similar Questions
A bullet with muzzvelocity 100 m/s is to be shot at a target 30 m away in the horizontal line .How high above the target must the renaimed so that the bullet will hit the target?
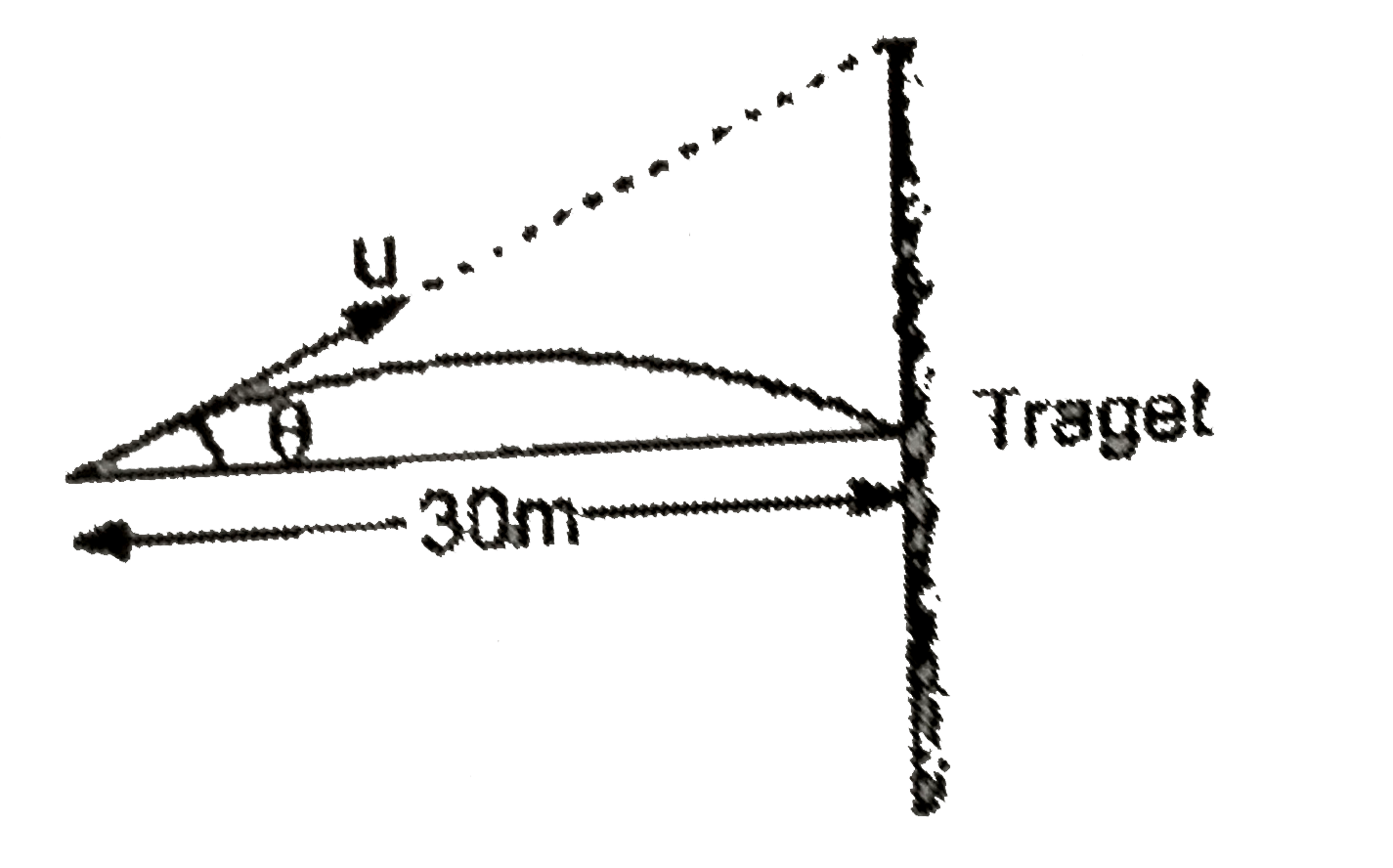
View SolutionA rifle with a muzzle velocity of 100m/s shoots a bullet at small target 30m away in the same horizontal line. How high above the traget must the gun be aimed so that the bullet will hit the target. (Hint: use small angle approximation.)
View Solution
Knowledge Check
A bullet with muzzle velocity 100ms−1 is to be shot at a target 30m away in the same horizontal line. How high above the target must the rifle be aimed so that the bullet will hit the target ?
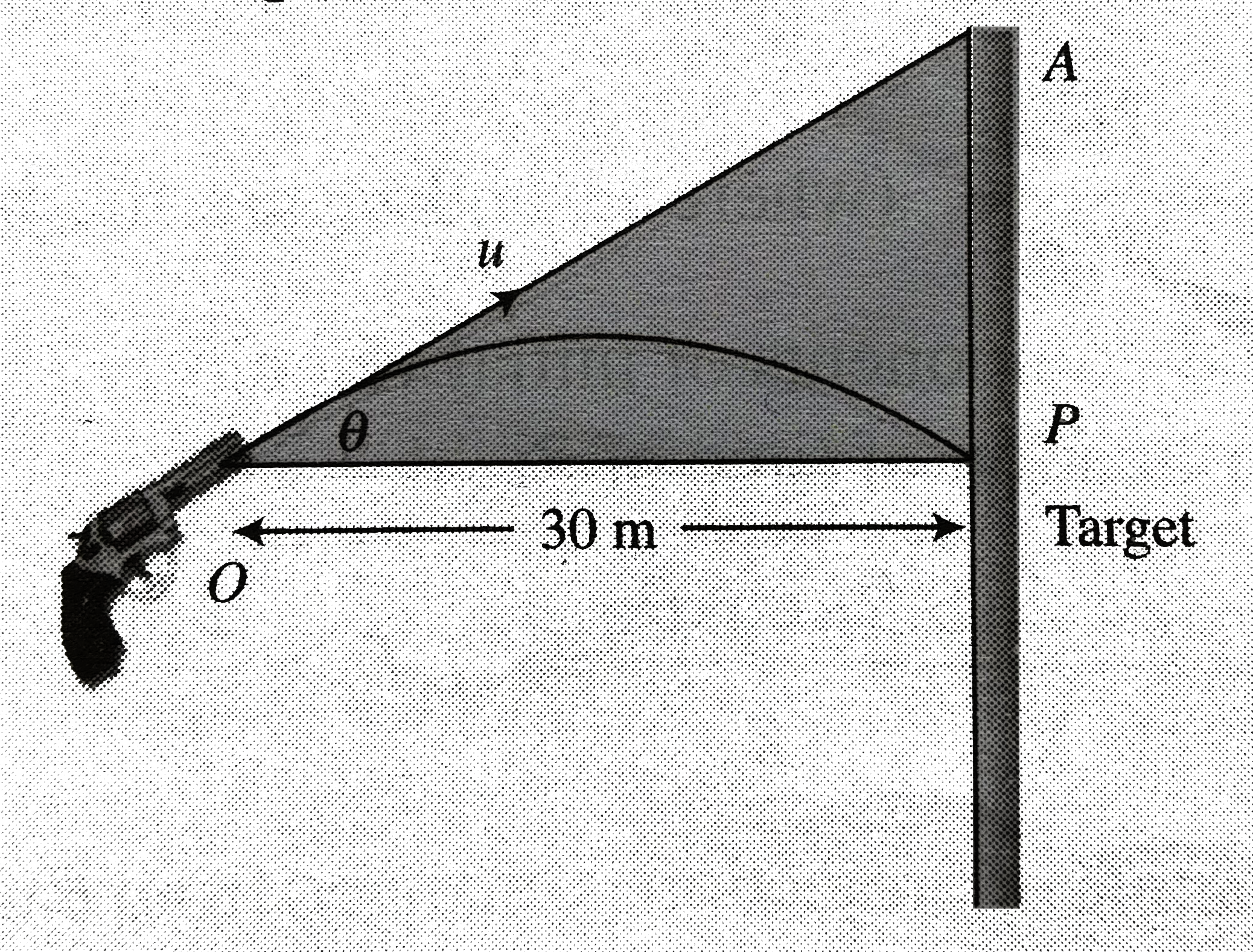 .A0B0.45cmC45cmD90cm
.A0B0.45cmC45cmD90cmA rifle shoots a bullet with a muzzle velocity of 400ms−1 at a small target 400m away. The height above the target at which the bullet must be aimed to hit the target is (g=10ms−2).
A1 mB5 mC10 mD0.5 mThe initial speed of a bullet fired from a rifle is 630 m/s. . The rifle is fired at the centre of a target 700 m away at the same level as the target. How far above the centre of target, the rifle must be aimed in order to hit the target?
A6.1 mB1.0 mC9.8 mD4.2m
A ball with projection velocity 10m/s is to hit a target 6m away in the same horizontal line. How high above the target must the ball be aimed so that the ball will hit the target ? (tan18.5∘=1/3,tan71.5∘=3)
View SolutionA bullet fired from a rifle travels at an average speed of 2520 km/hr. It hits the target after 0.2 seconds. How far (in m) is the target from the rifle ?
View SolutionA rifle is used to shoot twice at a target, using identical cartridges. The first time, the rifle is aimed parallel to the ground and directly at the center of the bull's-eye. The bullet strikes the target at a distance of HA below the center, however. The second time, the rifle is similarly aimed, but from twice the distance from the target. This time the bullet strikes the target at a distance of HB below the center. Find the ratio HB/HA.
View SolutionA bullet fired from a rifle travels at an average speed of 2520 km/hr. It hits the target after 0.2 seconds. How far (in metre) is the target from the rifle?
View SolutionA bullet of mass 10 g is fired with a velocity of 100 m/s from a rifle of mass 2.5 kg, the recoil velocity of the rifle is
View Solution
