An overweight acrobat, weighing in at 115 kg, wants to perform a single hand stand. He tries to cheat by resting one foot against a smooth frictionless vertical wall. The horizontal force there is 130 N. What is the magnitude of the force exerted by the floor on his hand? Answer in N.
1134
1257
997
1119
The correct Answer is:A
To solve the problem, we need to analyze the forces acting on the acrobat when he is in a handstand position with one foot against a frictionless vertical wall.
1. Identify the Weight of the Acrobat:
The weight (W) of the acrobat can be calculated using the formula:
W=m⋅g
where m=115kg (mass of the acrobat) and g=9.8m/s2 (acceleration due to gravity).
W=115kg⋅9.8m/s2=1127N
2. Identify the Horizontal Force:
The horizontal force (F_H) exerted by the wall is given as:
FH=130N
3. Set Up the Equilibrium Condition:
For the acrobat to be in equilibrium, the net force acting on him must be zero. The forces acting on him are:
- The weight (W) acting downwards.
- The normal reaction force (R_H) from the floor acting upwards.
- The horizontal force (F_H) from the wall acting horizontally.
The vertical forces must balance:
RH=W
4. Calculate the Resultant Force:
The resultant force (R) acting on the acrobat can be calculated using the Pythagorean theorem since the forces are perpendicular:
RH=√W2+F2H
Substituting the values:
RH=√(1127N)2+(130N)2
RH=√1270729+16900
RH=√1287629
RH≈1134N
5. Conclusion:
The magnitude of the force exerted by the floor on his hand is approximately:
RH≈1134N
Final Answer:
The magnitude of the force exerted by the floor on his hand is approximately 1134 N.
The weight (W) of the acrobat can be calculated using the formula:
W=m⋅g
where m=115kg (mass of the acrobat) and g=9.8m/s2 (acceleration due to gravity).
W=115kg⋅9.8m/s2=1127N
The horizontal force (F_H) exerted by the wall is given as:
FH=130N
For the acrobat to be in equilibrium, the net force acting on him must be zero. The forces acting on him are:
- The weight (W) acting downwards.
- The normal reaction force (R_H) from the floor acting upwards.
- The horizontal force (F_H) from the wall acting horizontally.
RH=W
The resultant force (R) acting on the acrobat can be calculated using the Pythagorean theorem since the forces are perpendicular:
RH=√W2+F2H
Substituting the values:
RH=√(1127N)2+(130N)2
RH=√1270729+16900
RH=√1287629
RH≈1134N
The magnitude of the force exerted by the floor on his hand is approximately:
RH≈1134N
The magnitude of the force exerted by the floor on his hand is approximately 1134 N.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DISHA PUBLICATIONCHAPTER - LAWS OF MOTIONEXERCISE - Exercise-1: Concept Builder (Topicwise) (Topic 5: Circular Motion and Banking of Road )
7 Videos
- JEE MAINS- 2019 (HELD ON :9TH APRIL 2019 (SHIFT-I))BOOK - DISHA PUBLICATIONCHAPTER - JEE MAINS- 2019 (HELD ON :9TH APRIL 2019 (SHIFT-I))EXERCISE - QUESTIONS
30 Videos
- MAGNETISM AND MATTERBOOK - DISHA PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - EXERCISE-2:CONCEPT APPLICATOR
31 Videos
Similar Questions
A uniform ladder of mass 10 kg leans against a smooth vertical wall making an angle of 530 with it. The other end rests on a rough horizontal floor. Find the normal force and the frictional force that the floor exerts on the ladder
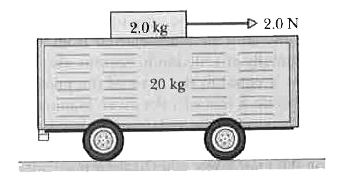
View SolutionIn Fig. 6-48, a 2.0 kg block lies on a 20 kg trolley that can roll across a floor on frictionless bearings. Between the block and the trolley, the coefficient of kinetic friction is 0.20 and the coefficient of static friction is 0.25. When a horizontal 2.0 N force is applied to the block, what are the magnitudes of (a) the frictional force between the block and the trolley and (b) the acceleration of the trolley?
View Solution
Knowledge Check
If a lodder weighing 250 N is placed against a smooth vertical wall having coefficient of friction between it and floor is 0.3 then what is the maximum force of friction available at the point of contact between the lodder and the floor?
A75NB50NC35ND25NIf a lodder weighing 250 N is placed against a smooth vertical wall having coefficient of friction between it and floor is 0.3 then what is the maximum force of friction available at the point of contact between the lodder and the floor?
A75NB50NC35ND25NA ladder wighing 300 N is placed against a smooth vertical wall having a coefficient of friction between it and the floor of 0.2. What is the maximum force of friction available at the point of contact between the ladder and the floor?
A40 NB50 NC60 ND70 N
A girl weighing 25 kg stands on the floor. She exerts a downward force of 250 N on the floor. What force does the floor exert on her ?
View SolutionA uniform cubical crate is 0.850 m on each side and weighs 500 N. It rests on a floor with one edge against a very small, fixed obstruction. At what least height above the floor must a horizontal force of magnitude 400 N be applied to the crate to tip it?
View SolutionEnd A of the bar AB in figure rests on a frictionless horizontal surface and end B is hinged. A horizontal force →F of magnitude 120N is exerted on end A. You can ignore the weight of the bar. What is the net force exerted by the bar on the hinge at B?

View SolutionThe foot of a uniform ladder is on a rough horizontal ground and the top rests against a smooth vertical wall. The weight of the ladder is 400 units. A man weighing 800 units stands on the ladder one quarter of its length from the bottom. If the inclination of the ladder to the horizontal is 30∘, the reaction at the wall is
View SolutionA weightless ladder 6m long rests against a frictionless wall at an angle of 60∘ from the horizontal. A 60kg man standing on it is 2m from the top of the ladder. A horizontal force is applied at the lower end to keep it from slipping. The magnitude of the force is
View Solution
