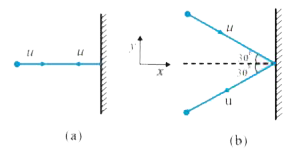
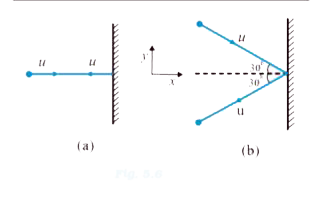
Two identical billiard balls strike a rigid wall with the same speed but at different angles, and get reflected without any change in speed, as shown in Fig. 5.6. What is (i) the direction of the force on the wall due to each ball? (ii) the ratio of the magnitudes of impulses imparted to the balls by the wall ?
 Solution in Telugu
Solution in Telugu
An instinctive answer to (i) might be that the force on the wall in case (a) is normal to the wall, while that in case (b) is inclined at 30° to the normal. This answer is wrong. The force on the wall is normal to the wall in both cases. How to find the force on the wall? The trick is to consider the force (or impulse) on the ball due to the wall using the second law, and then use the third law to answer (i). Let u be the speed of each ball before and after collision with the wall, and m the mass of each ball. Choose the x and y axes as shown in the figure, and consider the change in momentum of the ball in each case :
Case (a)
(px)initial = mu (py)initial=0
(px)final = -mu (py)final=0
Impulse is the change in momentum vector. Therefore ,
x-component of impulse = – 2 m u
y-component of impulse = 0
Impulse and force are in the same direction. Clearly, from above, the force on the ball due to the wall is normal to the wall, along the negative x-direction. Using Newton’s third law of motion, the force on the wall due to the ball is normal to the wall along the positive x-direction. The magnitude of force cannot be ascertained since the small time taken for the collision has not been specified in the problem.
case (b)
(px)initial= mu cos 30∘,(py)initial= - mu sin 30∘
(px)final = - mu cos 30∘,(py)final= - mu sin 30∘
Note while px changes sign after collision , py does not. therefore
x - component of impulse = - mu cos30∘
y = component impulse = 0
The direction of impulse (and force) is the same as in (a) and is normal to the wall along the negative x direction. As before, using Newton’s third law, the force on the wall due to the ball is normal to the wall along the positive x direction. The ratio of the magnitudes of the impulses imparted to the balls in (a) and (b) is
2mu/(2mu cos 30∘) = 2√3=1.2
Topper's Solved these Questions
- BOOK - NCERT TELUGUCHAPTER - LAW OF MOTIONEXERCISE - EXERCISE23videos
LAW OF MOTION
- BOOK - NCERT TELUGUCHAPTER - LAW OF MOTIONEXERCISE - EXERCISE (ADDITIONAL EXERCISE)17videos
LAW OF MOTION
- BOOK - NCERT TELUGUCHAPTER - KINETIC THEORYEXERCISE - ADDITIONAL EXERCISE3videos
KINETIC THEORY
- BOOK - NCERT TELUGUCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - EXERCISE45videos
MECHANICAL PROPERTIES OF FLUIDS
Similar Questions
Give the magnitude and direction of the net force acting on (a) a drop of rain falling down with a constant speed, (b) a cork of mass 10 g floating on water, (c) a kite skillfully held stationary in the sky, (d) a car moving with a constant velocity of 30 km/h on a rough road, (e) a high-speed electron in space far from all material objects, and free of electric and magnetic fields.
07:11View SolutionFigure shows the position-time graph of a body of mass 0.04 kg. Suggest a suitable physical context for this motion. What is the time between two consecutive impulses received by the body ? What is the magnitude of each impulse ?
 09:49View Solution
09:49View SolutionA metre long narrow bore held horizontally (and closed at one end) contains a 76 cm long mercury thread, which traps a 15 cm column of air. What happens if the tube is held vertically with the open end at the bottom ?
View SolutionExplain why
The blood pressure in humans is greater at the feet than at the brain.05:35View SolutionAs shown in fig. two identical balls strike a rigid wall with equal speeds but a different angles of incidence. They are reflected back without any lose in speed.


(a) Determine the direction of force exerted by each ball on the wall.
(b) Determine the ratio of impulse imparted by the two balls on the wall in both cases.08:58View SolutionTwo identical billiard balls striks a rigid wall with the same speed but at different angles , and get reflected without any change in speed , as shown in Fig . What is (i) the direction of the force on the wall due to each ball ? (ii) the ratio of the magnitudes of impulses imparted to the balls by the wall ?
03:50View SolutionA rigid ball of mass m strikes a rigid wall at 60^(@) and gets reflected without loss of speed as shown in the figure. The value of impulse imparted by the wll on the ball will be
05:00View Solutionदो सर्वसम बिलियर्ड गैंडे किसी दृढ़ दीवार से समान चाल से तुरंत भिन्न कोणों पर टकराती है तथा नीच दर्शये चित्र 5.6 की भांति चाल में बिना क्षय हुए परावर्तित हो जाती है । (i) प्रत्येक गेंद के कारण दीवार पर बल की दिशा क्या है ? तथा (ii) दीवार द्वारा दोनों गेंदोंपर लगे आवेगों का अनुपात क्या है?
02:15View Solutionदो सर्वसम बिलियर्ड गेंदें किसी दृढ़ दीवार से समान चाल से , परंतु भिन्न कोनों पर टकराती है तथा नीचे दर्शाए चित्र की भाँति चाल में बिना क्षय हुए परावर्तित हो जाती है ।
(i) प्रत्येक गेंद के कारण दीवार पर बल की दिशा क्या है ? तथा (ii) दीवार द्वारा दोनों गेंदों पर लगे आवेगों का अनुपात क्या है ?04:31View SolutionA ball of mass m strikes a rigid wall with speed v and gets reflected without any loss of speed, as shown in the figure. (a) What is the magnitude of the impulse imparted to the ball by the wall? (b) What is the direction of the force on the wall due to the ball?
04:11View SolutionTwo identical billiard balls strike a rigid wall with the same speed but at different angles, and get reflected without any change in speed, as shown in Fig. 5.6. What is (i) the direction of the force on the wall due to each ball? (ii) the ratio of the magnitudes of impulses imparted to the balls by the wall ?
 05:29View Solution
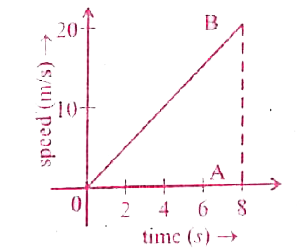
05:29View SolutionThe speed of a car as a function of time as shown figure. Find the acceleration and distance travelled by the car in 8 seconds .
 03:24View Solution
03:24View SolutionTwo identical billiard balls striks a rigid wall with the same speed but at different angles , and get reflected without any change in speed , as shown in Fig . What is (i) the direction of the force on the wall due to each ball ? (ii) the ratio of the magnitudes of impulses imparted to the balls by the wall ?
05:57View SolutionA rigid ball of mass m strikes a rigid wall at 60° and gets reflected without loss of speed as shown in the fiugre below. The value impulse imparted by the wall on the ball will be:
03:57View SolutionTwo identical billiard balls strike a rigid wall with the same speed but at different angles, and get reflected without any change in speed, as shown in figure. What is (i) the direction of the force on the wall due to each ball? (ii) the ratio of the magnitudes of impulses imparted to the balls by the wall ?
06:49View Solution

