Three deminsions. Three point particles are fixed in place in a xyz coordinate system. Particle A, at the origin, has mass mA. Particle B, at xyz coordinates (2.00d,1.00d. 200), has mass 2.00mA, and particle C, at coordinates (-1.00d,2.00d,-3.00d), has mass 3.00mA. A fourth particle D,with mass 4.00mA,is to be placed near the other particles. In terms of distance d, at what (a)x, (b)y, and (c)z coordinate should D be placed so that the net gravitational force on A from B, C, and D is zero ?
(a)−1.88d,(b)−3.90d,(c)0.489d. In this way, we are able to deduce that (x,y,z) = (1.88d,3.90d, 0.489d).
Topper's Solved these Questions
- GRAVITATIONBOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (SINGLE CORRECT CHOICE TYPE)
16 Videos
- GRAVITATIONBOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)
7 Videos
- GRAVITATIONBOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - CHECKPOINT
5 Videos
- GEOMETRICAL OPTICS : REFRACTIONBOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Practice questions(Integers)
4 Videos
- HEAT-MEASUREMENT AND TRANSFERBOOK - RESNICK AND HALLIDAYCHAPTER - HEAT-MEASUREMENT AND TRANSFEREXERCISE - PRACTICE QUESTIONS( INTEGER TYPE)
5 Videos
Similar Questions
What must be the momentum of a particle with mass m so that the total energy of the particle is 4.00 times its rest energy?
View SolutionIn Fig. 22-19, particle 1 of charge q1=5.00q and a particle 2 of charge q2+2.00q are fixed on the x-axis.
As a multiple of distance L, at what coordinate on the axis is the net electric field of the particles zero?
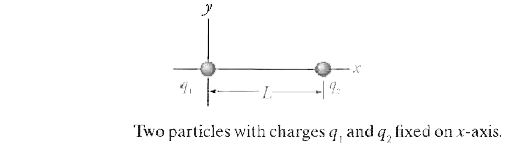
View Solution
Knowledge Check
The point equidistant from the O(0,0,0),A(a,0,0),B(0,b,0) and C(0,0,c) has the coordinates
A(a,b,c)B(a/2,b/2,c/2)C(a/3,b/3,c/3)D(a/4,b/4,c/4)A very large number of particle of same mass m are kept at horizontal distance of 1m,2m,4m,8m and so on from (0,0) point. The total gravitational at this point (0,0) is .
A−8GB−3GmC−4GmD−2GmConsider a system of two particles having masses m1 and m2. If the particle of mass m1 is pushed towards the centre of mass of particles through a distance d, by what distance would the particle of mass m2 move so as to keep the mass centre of particles at the original position?
Am1m1+m2dBm1m2dCdDm2m1d
Consider a system of two particles having masses m1 and m2. If the particle of mass m1 is pushed towards the centre of mass of particles through a distance d, by what distance would the particle of mass m2 move so as to keep the mass centre of particles at the original position?
View SolutionConsider a sytem of two particles having masses m1 and m2. If the particle of mass m1 is pushed towards the centre of mass of particles through a distance d, by what distance would the particle of mass m2 move so as to keep the mass centre of particles at the original position?
View SolutionIn Fig., particle 1 of charge +q and particle 2 of charge +9.00 q are held at separation L = 8.00 cm on an x axis. If particle 3 of charge q3 is to be located such that the three particles remain in place when released, what must be the (a) x and (b) y coordinates of particle 3, and (c ) the ratio q3/q ?
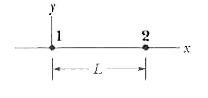
View SolutionA force (2.00ˆi−4.00ˆj+2.00ˆk)N acts on a particle located at (3.00ˆi+2.00ˆj−4.00ˆk)m. What is the magnitude of the torque on the particle as measured about the origin?
View SolutionThe coordinates of centre of mass of three particles of masses 1 kg, 2 kg, 3 kg are (2m, 2m, 2m). Where should a fourth particle of mass 4 kg be placed so that the coordinates of centre of mass are (4m, 4m, 4m)?
View Solution
