The locus of the point of intersection of the tangents to the circle $x^{2} + y^{2} = a^{2}$ at points whose parametric angles differ by $\frac{π}{3}$ .

Video Solution

Text Solution

Verified by Experts

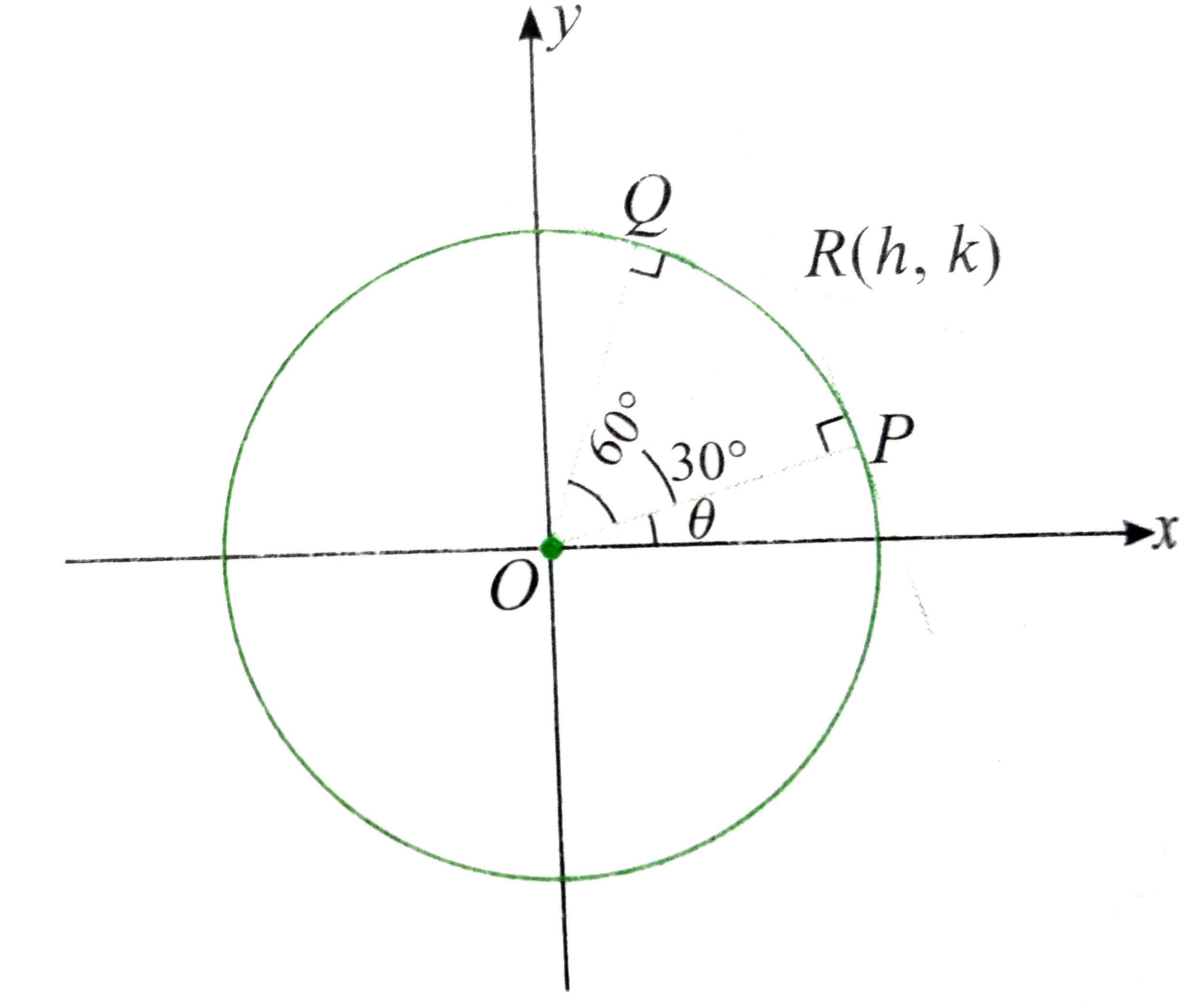
Let the points on the circle whose parametrci angles differ by $60^{\circ}$ be P (a cos $θ$ ,a sin $θ$ ) and $Q (a cos (θ + 60^{\circ})$ , $a sin (θ + 60^{\circ})$ . Tangents at points P and Q intersect at R(h,k).
In the figure, $∠ P O Q = 60^{\circ}$ and $∠ P O R = 30^{\circ}$ .
In triangle OPR,
$O P = O R {cos 30}^{\circ}$
$∴ a = \sqrt{h^{2} + k^{2}} \frac{\sqrt{3}}{2}$
Hence, locus of R is $3 (x^{2} + y^{2}) = 4$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The locus of the point of intersection of tangents to the circle $x^{2} + y^{2} = a^{2}$ at the points whose parametric angles differ by $π / 3$ is
A $x^{2} + y^{2} = a^{2}$
B $x^{2} + y^{2} = 4 a^{2}$
C $x^{2} + y^{2} = 4 a^{2} / 3$
D $x^{2} + y^{2} = 9$
Question 2 - Select One
The locus of the point of intersection of the tangents to the circle $x = a cos θ, y = a sin θ$ at the points, whose parametric angles differ by $\frac{π}{3}$ is
AStraight line
BEllipse
CCircle of radius 2a
DCircle of radius $\frac{2 a}{\sqrt{3}}$
Question 3 - Select One
Locus of the point of intersection of perpendicular tangents to the circle $x^{2} + y^{2} = 16$ is
A $x^{2} + y^{2} = 8$
B $x^{2} + y^{2} = 32$
C $x^{2} + y^{2} = 64$
D $x^{2} + y^{2} = 16$

CENGAGE-CIRCLE -JEE Advanced (Single Correct Answer Type)

Home
Ask Doubt
Profile