A circle x2+y2=a2 meets the x-axis at A(-a,0) and B(a,0). P(α) and Q(β) are two points on the circle so that α−β=2γ, where γ is a constant. Find the locus of the point of intersection of AP and BQ.
Let
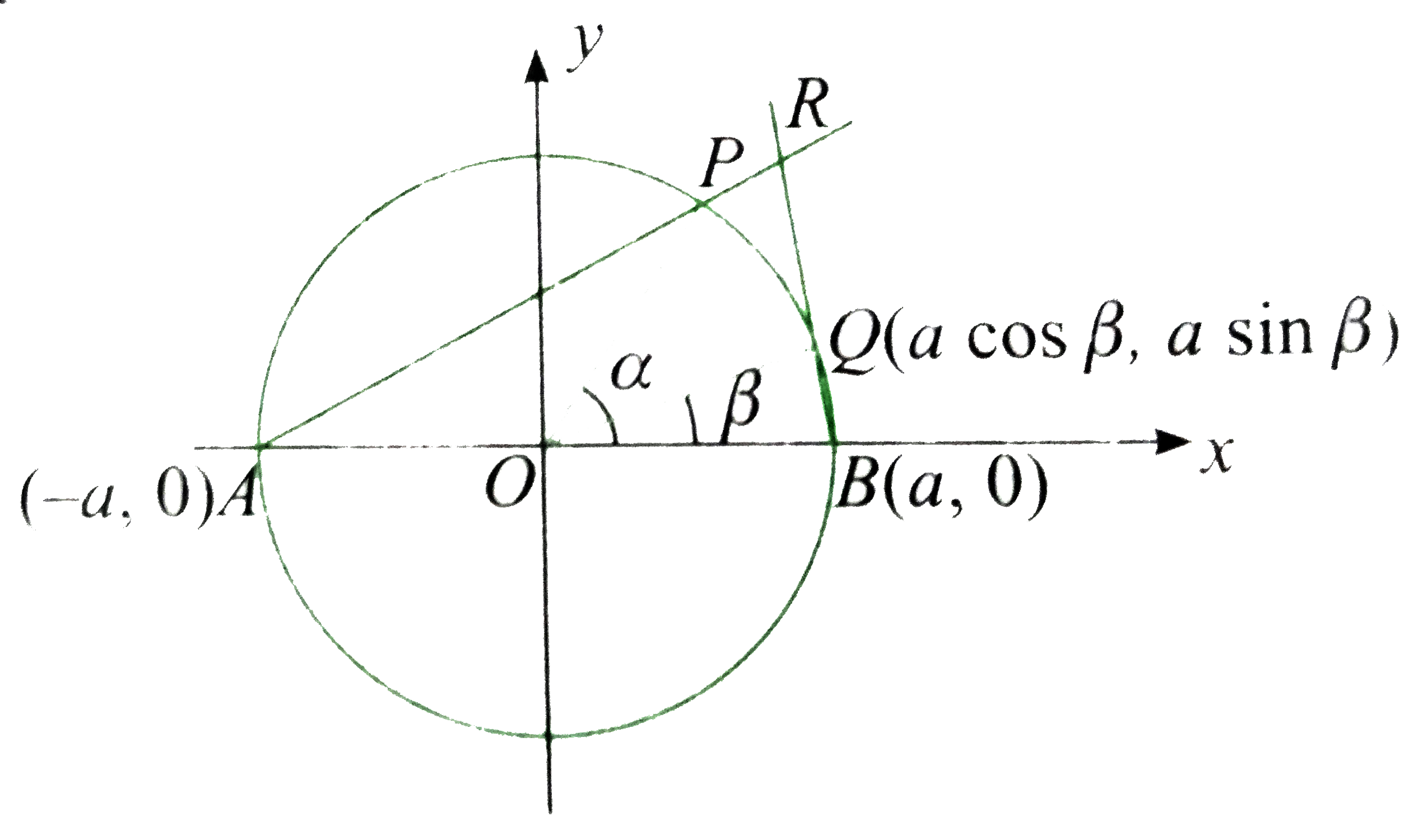
The coordinates of A are ( -a,0) and that of P are (acosα,asinα).
Therefore, the equation of AP is
y=asinαa(cosα+1)(x+a)
or y=tan.α2(x+a) (1)
Similarly, the equationof BQ is
y=asinβa(cosβ−1)(x−a)
or y=−cot.β2(x−a) (2)
We now eliminate α and β from (1) and (2). Therefore,
tan.α2=ya+x,tan.β2=a−xy
Now, α−β=2γ⇒γ=α2−β2
∴tanγ=tan.α2−tan.β21+tan.α2tan.β2=ya+x−a−xy1+ya+x.a−xy
or tanγ=y2−(a2−x2)(a+x)y+(a−x)y
=x2+y2−a22ay
or x2+y2−2aytanγ=a2
Topper's Solved these Questions
Similar Questions
A circle with center at the origin and radius equal to a meets the axis of x at AandB.P(α) and Q(β) are two points on the circle so that α−β=2y , where γ is a constant. Find the locus of the point of intersection of AP and BQ.
View SolutionIf alpha beta gamma are the roots of x3+x2−5x−1=0 then α+β+γ is equal to
View Solution
Knowledge Check
If the eccentric angles of two points P and Q on the ellipse x2a2+y2b2 are α,β such that α+β=π2, then the locus of the point of intersection of the normals at P and Q is
Aax+by=0Bax-by=0Cx+y=0Dx+y=a+bIf α,β,γ are the roots of x3+2x2−3x−1=0, then α−2+β−2+γ−2 is equal to
A12B13C14D15If α,β,γ are the parameters of points A,B,C on the circle x2+y2=a2 and if the triangle ABC is equilateral ,then
A∑cosα=0B∑sinα=0C∑tanα=0D∑cotα=0
If alpha beta gamma are roots of x3+x2−5x−1=0 then α+β+γ
is equal to
View SolutionI If a point (α,β) lies on the circle x2+y2=1 then the locus of the point (3α.+2,β), is
View SolutionIf α and β are roots of x2−3x+p=0 and γ and δ are the roots of x2−6x+q=0 and α,β,γ,δ are in G.P. then find the ratio of (2p+q):(2p−q)
View SolutionThe circle x2+y2=4 ,cuts the line joining the points A(1,0),&B(3,4) in two points P& Q.Let BPPA=α>0&BQQA=β>0 .Then α+β
View SolutionIf α,β,γ are the roots of x3−px2+qx−r=0then(α+β)(β+γ)(γ+α)=
View Solution
