Question

(a) Explain the principle of working of a potentiometer.
(b) In a potentiometer, a standard source of emf 5 V and negligible internal resistance maintains a steady current through the potentiometer wire of length wire of length 10 m. Two primary cells of emf $E_1\text{and}{E}_2$ are joined together in a series with (i) same polarity and (ii) opposite polarity. The combination is connected to the potentiometer circuit in each case. The balancing length of the wire in the two cases are found to be 700 cm and 100 m , respectively . Find the values of emf of the two cells.

Explanation

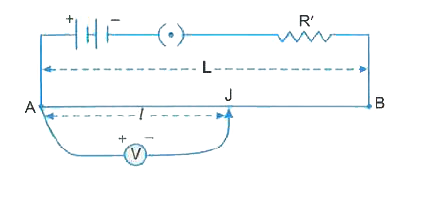
(a) Let a battery of emf `epsilon_0` us connected across a potentiometer wire of length L and resistance R and an external series resistance R..
Then constant current flowing through the potentiometer wire is
`I=(epsilon_0)/((R+R.))`
`:.` Fall in potential along the potentiometer wire `= IR = (epsilon_0R)/((R+R.)L)` ,
and the fall in potential per (i.e., the potential gradient) `k= (epsilon_(0)R)/((R+R^(.)L))` which is a constant.
Thus, potential drop across a length l of potentiometer wire V = kl , which is the basic, working principle of the potentiometer .
Here emf of driver cell E = 5 V, length of of potentiometer wire L = 10 m = 100 cm
`:.` Potential gradient k `E/L=(5V)/(100 cm ) = 0.005V cm ^(-1)`
(i) When cells of emf `E_1 and E_2` are joined together in series with same polarity then balancing length `l_1 = 700 cm ` and we have
`E_1 +E_2 =krho_(1) = 0.005xx700= 3.5 " "...(i)`
(ii) When the same cells are joined in series with opposite polarity , the balancing length `l_2=100 cm` and we have
`E_1 -E_(2)=krho_(2)=0.005xx100=0.5 " "...(ii)`
From (i) and (ii) , on solving , we get `E_1 = 2.0 V and E_2 = 1.5 V`