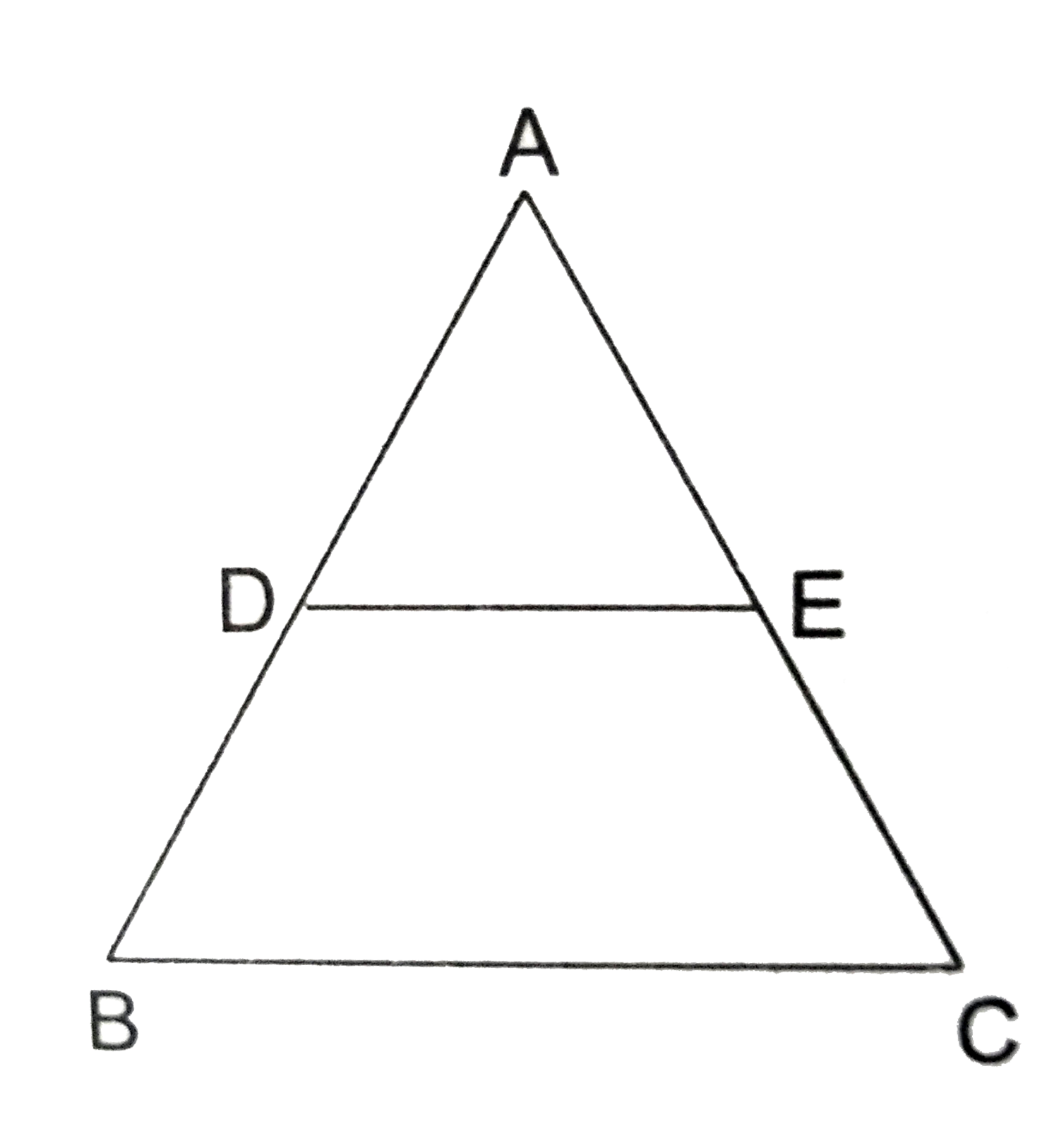
The line drawn from the midpoint of one side of a triangle parallel to another side bisects the third side.
GIVEN AΔ in which D is the midpoint of AB and DE∣∣BC, meeting AC at E.
TO PROVE AE+EC.
PROOF Since DE∣∣BC, by Thale's theorem, we have
AEEC=ADDB=1[∴AD=DB(given)]
⇒AEEC=1⇒AE=EC
Topper's Solved these Questions
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLE
4 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - EXERCISE 7 A
1 Videos
- T-RATIOS OF SOME PARTICULAR ANGLESBOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 11
27 Videos
- TRIGONOMETRIC IDENTITIESBOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself
20 Videos
Similar Questions
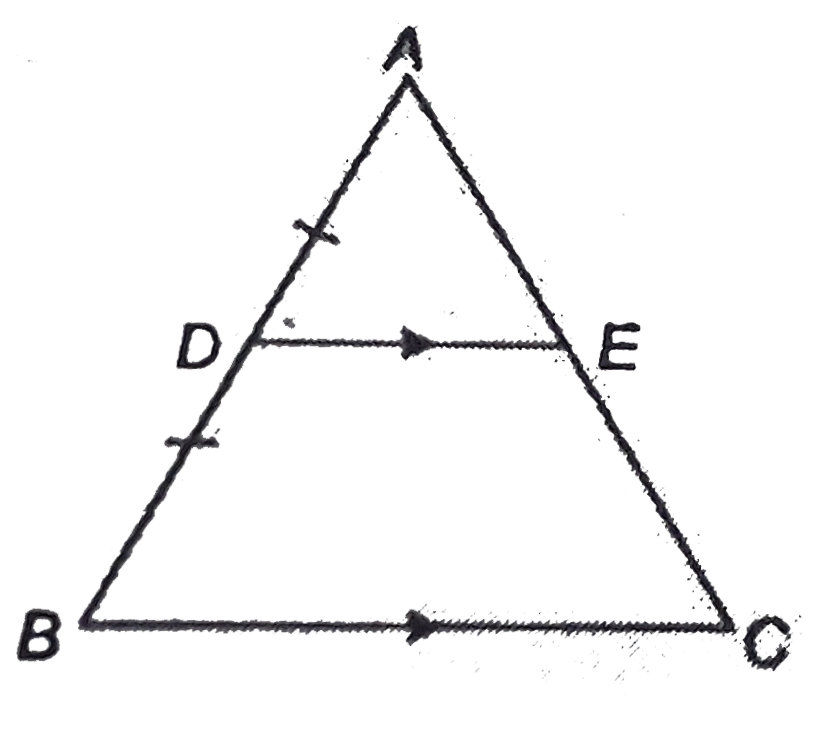
Using Theorem 6.1, prove that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side. (Recall that you have proved it in Class IX).
View SolutionProve using similar triangles, that a line drawn through the mid-point of one side of a triangle parallel to another side, bisects the third side.
View Solution
Knowledge Check
The line segment joining the midpoints of any two sides of a triangle is parallel to the third side and equal to half of it.
In the given figure, the side AC of ΔABC is produced to E such that CE = FAC. If D is the midpoint of BC and ED produced meets AB at F and CP, DQ are drawn parallel to BA, then FD=
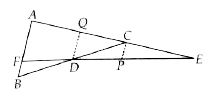 A12FEB13EFC2FEDFE
A12FEB13EFC2FEDFE
Theorem 8.10 : The line drawn through the mid-point of one side of a triangle, parallel to another side bisects the third side.
View SolutionThe line drawn through the mid-point of one side of a triangle, parallel to another side, intersects the third side at its mid-point.
View SolutionThe line drawn through the mid-point of one side of a triangle is parallel to another side and intersects the third side at its mid-point.
View SolutionThe line joining the mid-points of two sides of a triangle is parallel to the third side.
View Solutionprove that the line segment joining the mid-points of two sides of a triangle is parallel to the third side.
View SolutionProve that the line segment joining the mid points of two sides of a triangle is parallel to its third side.
View SolutionProve that the line joining the middle points of the two sides of a triangle is parallel to the third side.
View Solution
