What is the area of the triangle bounded by the side x=0,y=0 and x+y=2 ?
A
1 square unit
B
2 square unit
C
4 square unit
D
8 square unit
Video Solution
Text Solution
The correct Answer is:B
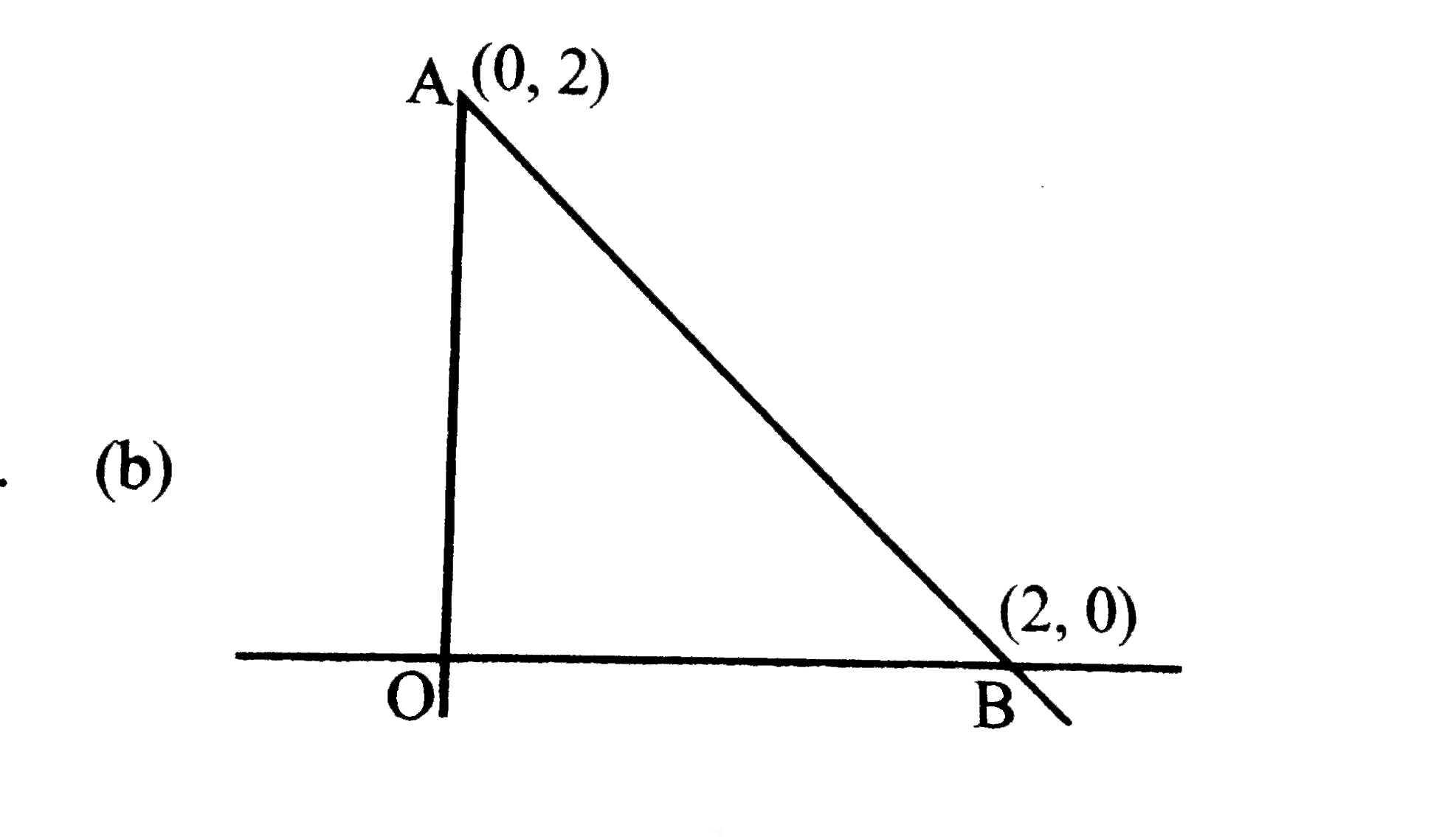
Area of ΔOAB=12×OB×OA
=12×2×2=2 square units.
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
What is the area of the triangle formed by the lines y−x=0,y+x=0,x=c ?
Ac/2Bc2C2c2Dc2/2What is the area of the region bounded by the lines y=x.y=0 and x=4?
A4 sq. unitsB8 sq. unitsC12 sq. unitsD16 sq. unitsWhat is the area of the region bounded by the lines y=x,y=0 and x=4 ?
A4 square unitsB8 square unitsC12 square unitsD16 square units
Find the area of the triangle formed theby lines y−x=0,x+y=0andx−k=0.
View SolutionArea of triangle formed by straight lines x=0,x+2y=0andy=1
View SolutionWhat is the area bounded by the lines x=0,y=0 and x+y+2=0?
View SolutionWhat is the area bounded by the lines x=0,y=0 and x+y+2=0 ?
View SolutionThe area of the figure bounded by y=ex−1,y=0,x=0andx=2 is
View Solution
