What is the ratio in which the point C(−27,−207) divides the line joining the points A(−2,−2) and B(2,−4) ?
A
1:3
B
3:4
C
1:2
D
2:3
Video Solution
Text Solution
The correct Answer is:B
C=(2K−2K+1,−4K+2K+1)
So, 2K−2K+1=−27
C(−27,−207)
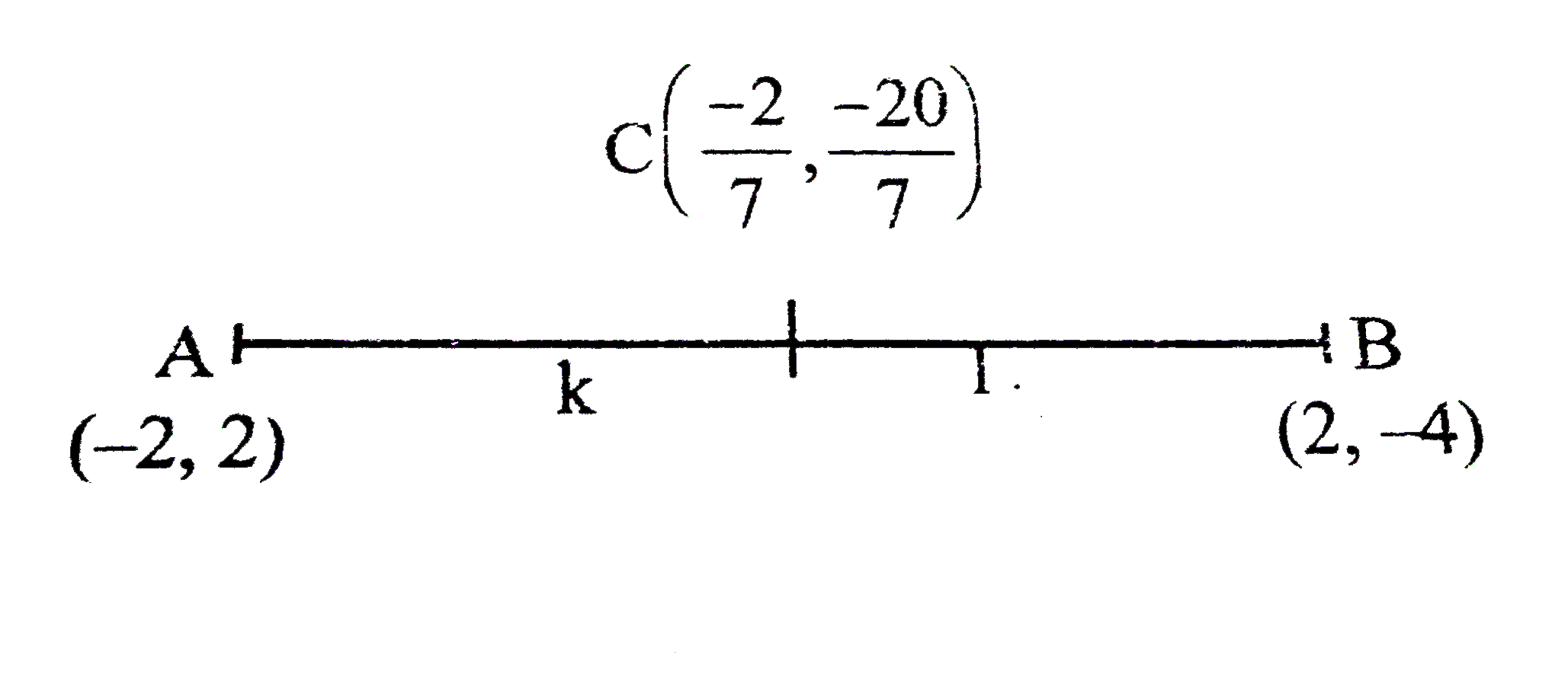
implies (cancel(2)(K-1))/(K+1)=cancel(-2)/7
⇒7K−7=−K−1
⇒8K=6⇒K=34
∴K:1=34:1=3:4
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The ratio in which the point, P(-3,x) divide the line segment joining the points A(-5,-4) and B(-2,3) is :
A3:2B4:7C2:1D5:3The ratio in which the point (5, 4) divides the line joining points (2, 1) and (7,6 ) is…..
A3:2B2:3C1:3D2:3The ratio by which the line 2x + 5y -7=0 divides the straight line joining the points (-4,7) and (6,-5) is
A1:4B1:2C1:1D2:3
Find the ratio in which the point (2, y) divides the line segment joining the points A (-2, 2) and B (3, 7). Also find the value of y
View SolutionWhat is the ratio in which the point P(−25,6) divides the line joining of A (-4, 3) and B (2, 8)?
View SolutionFind the ratio in which the point (2, y) divides the line segment joining the points A(−2, 2) and B(3, 7) . Also, find the value of y .
View SolutionThe ratio in which the plane x−2y+3z=17 divides the line joining the points (2,−4,7)and(3,−5,8) is
View SolutionFind the ratio in which the line x + 3y -14 = 0 divides the line segment joining the points A (-2, 4) and B(3. 7).
View Solution
