Consider the collision depicted in Fig. 6.10. to be between two billiard balls with equal masses . The first ball is called the cue while the second ball is called the target. The billiard player wants to 'sink' the target ball in a corner pocket, which is at an angle . Assume that the collision is elastic and that friction and rotational motion are not important. Obtain .
From momentum conservation, since the masses are equal
or,
Since the collision is elastic and it follows from conservation of kinetic energy that
(6.33)
Comparing Eqs. (6.32) and (6.33), we get
or,
Thus, .
This proves the following result : when two equal masses undergo a glancing elastic collision with one of them at rest, after the collision, they will move at right angles to each other.
Topper's Solved these Questions
Similar Questions
Consider the collision depicted in Figure, to be between two billiard balls with equal masses m1=m2. The first ball is called the cue and the second ball is called the target. The billiard player wants to sink the target ball in a corner pocket, which is at an angle θ2=ϕ=37∘. Assume that the collision is elastic and that friction and rotational motion are not important. Obtain θ1=θ .
View SolutionA billiard table whose length and width are as shown in the figure. A ball is placed at point A. at what angle θ the ball be projected so that after colliding with two walls, the ball will fall in the pocket B? assume that all collisions are perfectly elastic: (neglect friction)
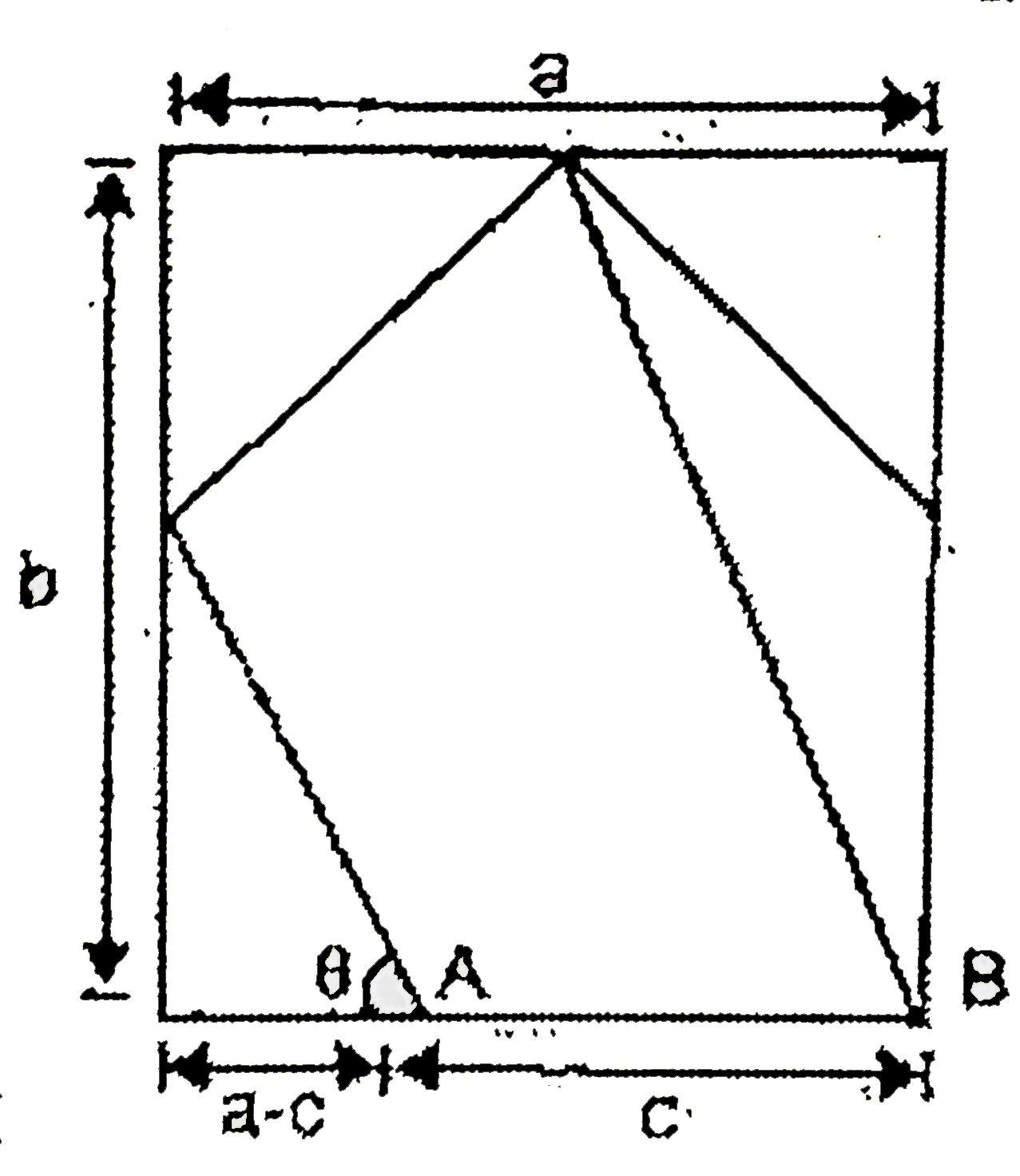 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A billiards player hits a stationary ball by an identical ball to pocket the target ball in a corner pocket that is at an angle of 35∘ with respect to the direction of motion of the first ball. Assuming the collision as elastic and that friction and rotational motion are not important, the angle made by the target ball with repect to the incoming ball is
A35∘B50∘C55∘D60∘ - Question 2 - Select One
A spherical ball of mass m1 collides head on with another ball of mass m2 at rest . The collision is elastic . The fraction of kinetic energy lost by m1 is :
A4m1m2(m1+m2)2Bm1m1+m2Cm2m1+m2Dm1m2(m1+m2)2 - Question 3 - Select One
A spherical ball of mass m1 colliddes head on with another ball of mass m2 at rest . The collision is elastic . The fraction of kintic energy lost by m1 is :
A4m1m2(m1+m2)2Bm1m1+m2Cm2m1+m2Dm1m2(m1+m2)2
A particle of mass m is projected towards a wall such that the angle of incidence is θ and the speed just before collision is u. Assuming that the wall is smooth and the collision is elastic, show that the ball rebounds at same angle.
View SolutionA ball of mass m moving with velocity u collides head-on which the second ball of mass m at rest. If the coefficient of restitution is e and velocity of first ball after collision is v1 and velocity of second ball after collision is v2 then
View SolutionA ball of mass m moving with velocity v collides head-on which the second ball of mass m at rest. I the coefficient of restitution is e and velocity of first ball after collision is v1 and velocity of second ball after collision is v2 then
View SolutionWhich one of the following statement does not hold good when two balls of masses m1 and m2 undergo elastic collision
View SolutionA ball of mass m moving with velocity v collides head-on which the second ball of mass m at rest. I the coefficient of restitution is e and velocity of first ball after collision is v1 and velocity of second ball after collision is v2 then
View Solution

