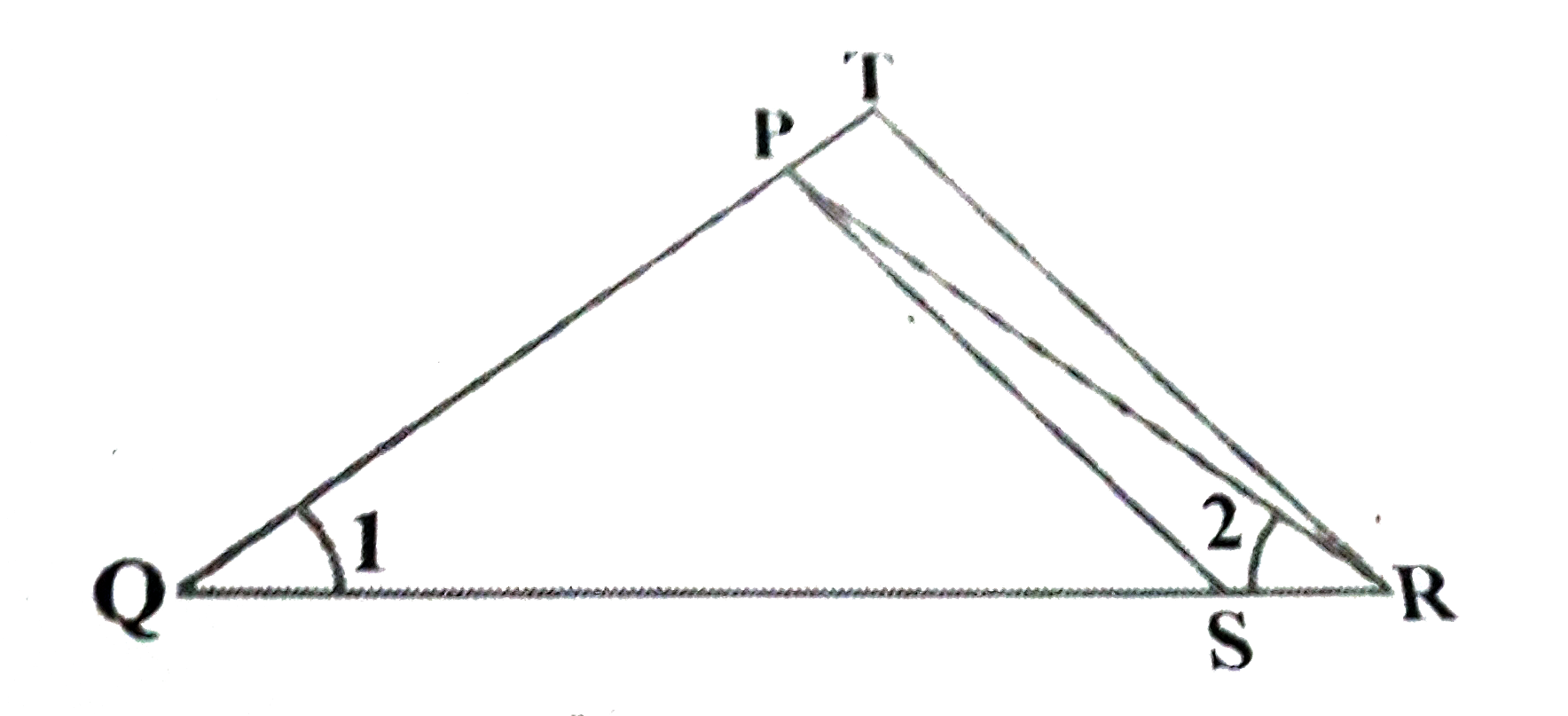
S and T are points on sides PR and QR of ΔPQR such that ∠P=∠RTS. Show that ΔRPQ~ΔRTS.
To prove that triangle ΔRPQ is similar to triangle ΔRTS, we will use the Angle-Angle (AA) similarity criterion. Here are the steps to follow:
Step 1: Identify the Given Information
We are given that:
- S and T are points on sides PR and QR of triangle PQR.
- ∠P=∠RTS.
Step 2: Identify the Common Angle
Observe that both triangles ΔRPQ and ΔRTS share a common angle:
- ∠R is common to both triangles.
Step 3: Apply the Given Information
From the given information, we have:
- ∠P=∠RTS (from the problem statement).
- ∠R=∠R (common angle).
Step 4: Establish Angle Relationships
We now have two angles in triangle ΔRPQ that are equal to two angles in triangle ΔRTS:
- ∠P=∠RTS
- ∠R=∠R
Step 5: Use the Angle-Angle (AA) Criterion
Since two angles of triangle ΔRPQ are equal to two angles of triangle ΔRTS, we can conclude by the Angle-Angle (AA) similarity criterion that:
ΔRPQ∼ΔRTS
Conclusion
Thus, we have shown that triangle ΔRPQ is similar to triangle ΔRTS.
---
We are given that:
- S and T are points on sides PR and QR of triangle PQR.
- ∠P=∠RTS.
Observe that both triangles ΔRPQ and ΔRTS share a common angle:
- ∠R is common to both triangles.
From the given information, we have:
- ∠P=∠RTS (from the problem statement).
- ∠R=∠R (common angle).
We now have two angles in triangle ΔRPQ that are equal to two angles in triangle ΔRTS:
- ∠P=∠RTS
- ∠R=∠R
Since two angles of triangle ΔRPQ are equal to two angles of triangle ΔRTS, we can conclude by the Angle-Angle (AA) similarity criterion that:
ΔRPQ∼ΔRTS
Thus, we have shown that triangle ΔRPQ is similar to triangle ΔRTS.
Similar Questions
S and T are points on sides PR and QR of ΔPQR such that ∠P=∠RTS Show that ΔRPQ~ΔRTS
View SolutionIn figure QRQS=QTPRand ∠1=∠2. Show that ΔPQS~ΔTQR.
View SolutionIn Figure, S and T are points on the sides PQ and PR respectively of △PQR such that PT=2cm , TR=4cm and ST is parallel to QR . Find the ratio of the areas of △PST and △PQR .
View SolutionIn Fig. 4.99, if ∠P=∠RTS , prove that RPQ~RTS . (FIGURE)
View SolutionIn Figure, T is a point on side QR of PQR and S is a point such that RT=ST. Prove That : PQ+PR>QS
View SolutionIn figure Cm and RN are respectively the medians of ΔABCand ΔPQR. If ΔABC~ΔPQR, prove that: (i) ΔAMC~ΔPNR (ii) CMRN=ABPQ (ii) ΔCMB~ΔRNQ
View SolutionIn Figure, PQRS is a quadrilateral and T and U are respectively points on PS and RS such that PQ=RQ, ∠PQT=∠RQU and ∠TQS=∠UQS. Prove that QT=QU.
View SolutionIn figure, PQ=PR . Show that PS>PQ.
View SolutionProve that the figure formed by joining the mid-points of the pair of consecutive sides of a quadrilateral is a parallelogram. OR ABCD is a parallelogram in which P,Q,R and S are mid-points of the sides AB,BC,CD and DA respectively. AC is a diagonal. Show that : PQ∣∣AC and PQ=12AC SR∣∣AC and SR=12AC PQ=SR (iv) PQRS is a parallelogram.
View SolutionIn PQR , S is any point on the side QR . Show that PQ+QR+RP>2PS
View Solution
