Suppose you drop a die at random on the rectangular region shown in Figure. What is the probability that it will land inside the circle with diameter 1m?
To find the probability that a die will land inside a circle with a diameter of 1 meter when dropped randomly on a rectangular region, we can follow these steps:
Step 1: Identify the dimensions of the circle and rectangle
- The diameter of the circle is given as 1 meter.
- Therefore, the radius r of the circle is:
r=12 meters
- The dimensions of the rectangle are given as length l=3 meters and breadth b=2 meters.
Step 2: Calculate the area of the circle
- The area Ac of a circle is calculated using the formula:
Ac=πr2
- Substituting the radius:
Ac=π(12)2=π⋅14=π4 square meters
Step 3: Calculate the area of the rectangle
- The area Ar of the rectangle is calculated using the formula:
Ar=length×breadth=l⋅b
- Substituting the dimensions:
Ar=3×2=6 square meters
Step 4: Calculate the probability
- The probability P that the die will land inside the circle is given by the ratio of the area of the circle to the area of the rectangle:
P=AcAr=π46
- Simplifying this gives:
P=π4×6=π24
Final Answer
The probability that the die will land inside the circle is:
P=π24
- The diameter of the circle is given as 1 meter.
- Therefore, the radius r of the circle is:
r=12 meters
- The dimensions of the rectangle are given as length l=3 meters and breadth b=2 meters.
- The area Ac of a circle is calculated using the formula:
Ac=πr2
- Substituting the radius:
Ac=π(12)2=π⋅14=π4 square meters
- The area Ar of the rectangle is calculated using the formula:
Ar=length×breadth=l⋅b
- Substituting the dimensions:
Ar=3×2=6 square meters
- The probability P that the die will land inside the circle is given by the ratio of the area of the circle to the area of the rectangle:
P=AcAr=π46
- Simplifying this gives:
P=π4×6=π24
The probability that the die will land inside the circle is:
P=π24
Similar Questions
Suppose you drop a tie at random on the rectangular region shown in Fig. 13.14. What is the probability that it will land inside the circle with diameter 1 m? (FIGURE)
View SolutionA missing helicopter is reported to have crashed somewhere in the rectangular region shown in Fig. 15.2. What is the probability that it crashed inside the lake shown in the figure?
View Solution
Knowledge Check
In the figure, what is the average of the five angles shown inside the circle?
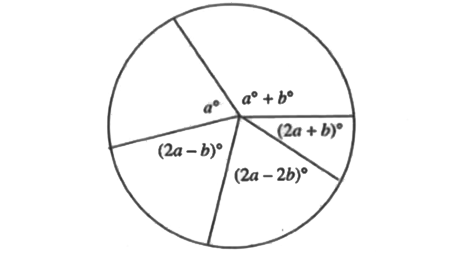 A36B45C60D72
A36B45C60D72A and B are centers of two circles that touch each other externally, as shown in the figure.What is the area of the circle whose diameter is AB?
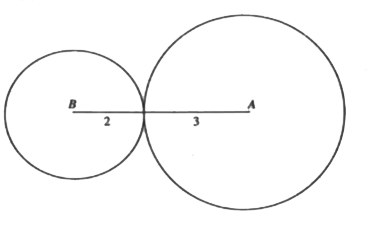 A4πB25π/4C9πD16π
A4πB25π/4C9πD16π
A missing helicopter is reported to have crashed somewhere in the rectangular region in Fig. 13.5. What is the probability that it is crashed inside the lake shown in the figure? (FIGURE)
View SolutionIn figure, a dart is thrown and lands in the interior of the circle. What is the probability that the dart will land in the shaded region?
View SolutionIn the Figure, a square dart board is shown. The length of a side of the larger square is 1.5 times the length of a side of the smaller square. If a dart is thrown and lands on the larger square. What is the probability that it will land in the interior of the smaller square?
View SolutionA circle with diameter 20 cm is drawn somewhere on a rectangular piece of paper with length 40 cm and width 30 cm. This paper is kept horizontal on table top and a dice, very small in size, is dropped on the rectangular paper without seeing towards it. If the dice falls and lands on the paper only, find the probability that it will fall and land inside the circle.
View SolutionIn the Fig. 13.12, JKLM is a square with sides of length 6 units. Points A and B are the mid-points of sides KL and LM respectively. If a point is selected at random from the interior of the square. What is the probability that the point will be chosen from the interior of JAB ? (FIGURE)
View SolutionA target shown in Figure, consists of three concentric circles of radii 3,7 and 9cm respectively. A dart is thrown and lands on the target. What is probability that the dart will and on the shaded region?
View Solution
