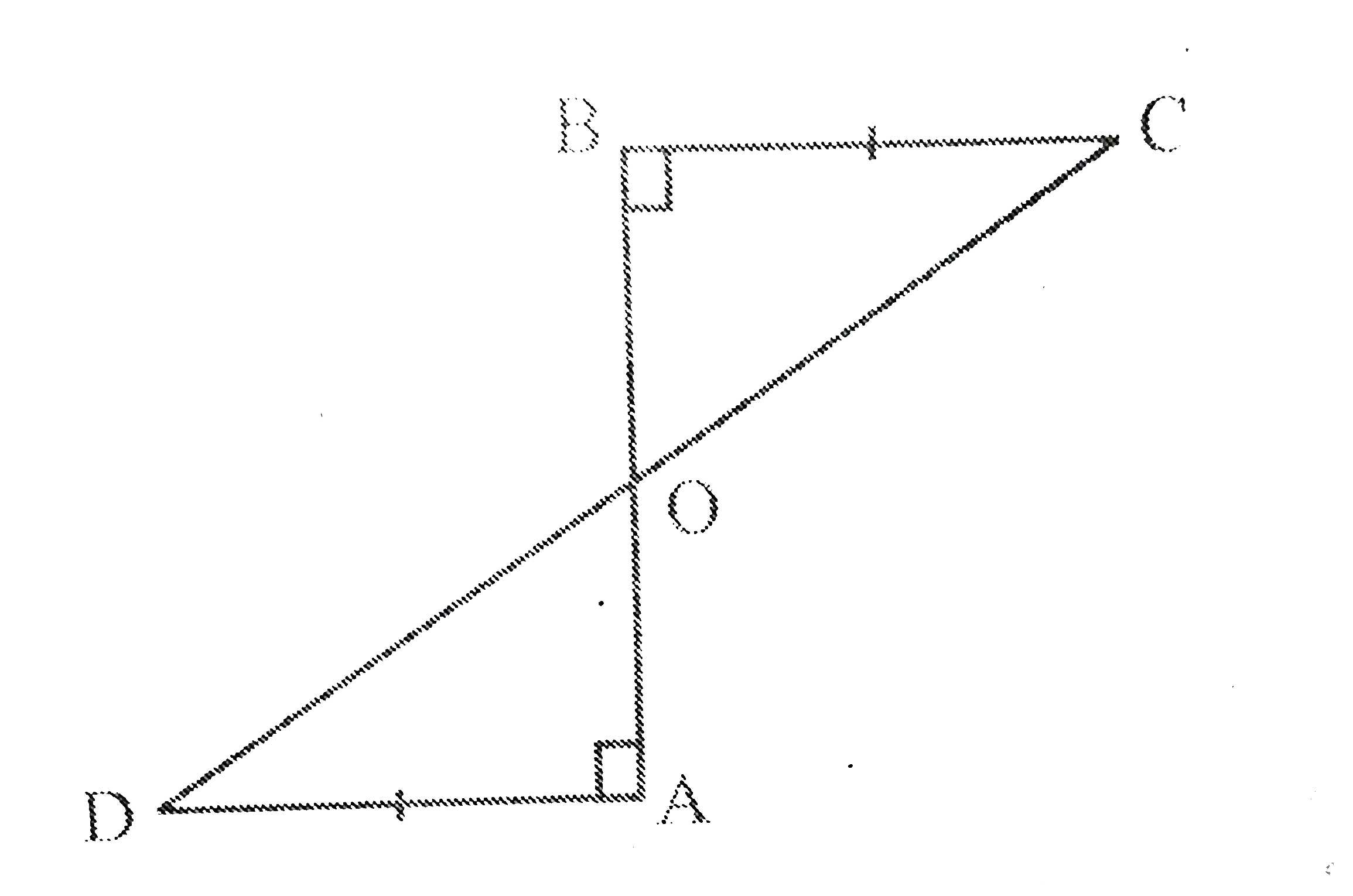
P is a point equidistant from two lines l and m intersecting at point A (see Fig. 7.38). Show that the line AP bisects the angle between them.
Perpendicular from P to l and m
PB=PC
∠PBA=∠PCA
In∠ABPand∠ACP
∠ABP=∠ACP
AP=AP
BP=CP
∠ABP≅∠ACP
=AP is angle bisector of ∠BAC
Topper's Solved these Questions
- TRIANGLESBOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - EXERCISE 7.5
4 Videos
- TRIANGLESBOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - EXERCISE 7.2
8 Videos
- TRIANGLESBOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - EXERCISE 7.1
8 Videos
- SURFACE AREAS AND VOLUMESBOOK - NCERT ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - Exercise 13.9
3 Videos
Similar Questions
Knowledge Check
The two regression lines intersect at unique point, then it should be
A(→x,→y)B(0,0)C(−−x,−−y)DNone of these
Two parallel straight lines are inclined to the horizon at an angle ∝. A particle is projected from a point mid way between them so as to graze one of the lines and strikes the other at right angle. Show that if θ is the angle between the direction of projection and either of lines, then tanθ=(√2−1)cot∝.
View SolutionP is a point on either of the two lines y−√3|x|=2 at a distance 5 units from their point of intersection The coordinates of the foot of the perpendicular from P on the bisector of the angle between them are
View SolutionIf the two equal chords of a circle intersect :
(i) inside
(ii) on
(iii) outside
the circle, then show that the line segment joining the point of intersection to the centre of the circle will bisect the angle between the chords.
View SolutionTwo circles with centres A and B intersect each other at points P and Q. Prove that the centre-line AB bisects the common chord PQ perpendicularly.
View SolutionA point P (2, -1) is equidistant from the points (a,7) and (-3,a). Find a.
View SolutionIn triangle LMN, bisector of interior angles at L and N intersect each other at point A. Prove that :
AM bisects angle LMN.
View SolutionAB is a line segment. P and Q are points on opposite sides of AB such that each of them is equidistant from the points A and B (in figure). Show that the line PQ is perpendicular bisector of AB.
View Solution