A charge of 8 mC is located at the origin. Calculate the work done in taking a small charge of −2×10−9 from a point P(0, 0, 3cm) to a point Q (0, 4cm, 0) via a point R(0, 6cm, 9cm).
To calculate the work done in moving a charge of −2×10−9 C from point P(0, 0, 3 cm) to point Q(0, 4 cm, 0) via point R(0, 6 cm, 9 cm) in the electric field created by a charge of 8 mC located at the origin, we will follow these steps:
Step 1: Calculate the Electric Potential at Point P
The electric potential V due to a point charge Q at a distance r is given by the formula:
V=k⋅Qr
where k=9×109N m2/C2 (Coulomb's constant).
For point P:
- Charge Q=8mC=8×10−3C
- Distance OP=3cm=0.03m
Calculating VP:
VP=9×109⋅8×10−30.03=72×1060.03=24×108V
Step 2: Calculate the Electric Potential at Point Q
For point Q:
- Distance OQ=4cm=0.04m
Calculating VQ:
VQ=9×109⋅8×10−30.04=72×1060.04=18×108V
Step 3: Calculate the Change in Electric Potential
The change in electric potential when moving from P to Q is:
ΔV=VQ−VP=18×108−24×108=−6×108V
Step 4: Calculate the Work Done
The work done W in moving a charge q0 through a potential difference ΔV is given by:
W=q0⋅ΔV
where q0=−2×10−9C.
Calculating W:
W=(−2×10−9)⋅(−6×108)=1.2J
Final Answer
The work done in moving the charge from point P to point Q via point R is 1.2J.
---
The electric potential V due to a point charge Q at a distance r is given by the formula:
V=k⋅Qr
where k=9×109N m2/C2 (Coulomb's constant).
- Charge Q=8mC=8×10−3C
- Distance OP=3cm=0.03m
VP=9×109⋅8×10−30.03=72×1060.03=24×108V
For point Q:
- Distance OQ=4cm=0.04m
VQ=9×109⋅8×10−30.04=72×1060.04=18×108V
The change in electric potential when moving from P to Q is:
ΔV=VQ−VP=18×108−24×108=−6×108V
The work done W in moving a charge q0 through a potential difference ΔV is given by:
W=q0⋅ΔV
where q0=−2×10−9C.
W=(−2×10−9)⋅(−6×108)=1.2J
The work done in moving the charge from point P to point Q via point R is 1.2J.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - NCERT ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - Exercise
37 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise
15 Videos
- MAGNETISM AND MATTERBOOK - NCERT ENGLISHCHAPTER - MAGNETISM AND MATTEREXERCISE - Exercise
25 Videos
Similar Questions
As per this diagram a point charge +q is placed at the origin O. Work done in taking another point charge −Q from the point A(0,a) to another point B(a,0) along the staight path AB is:
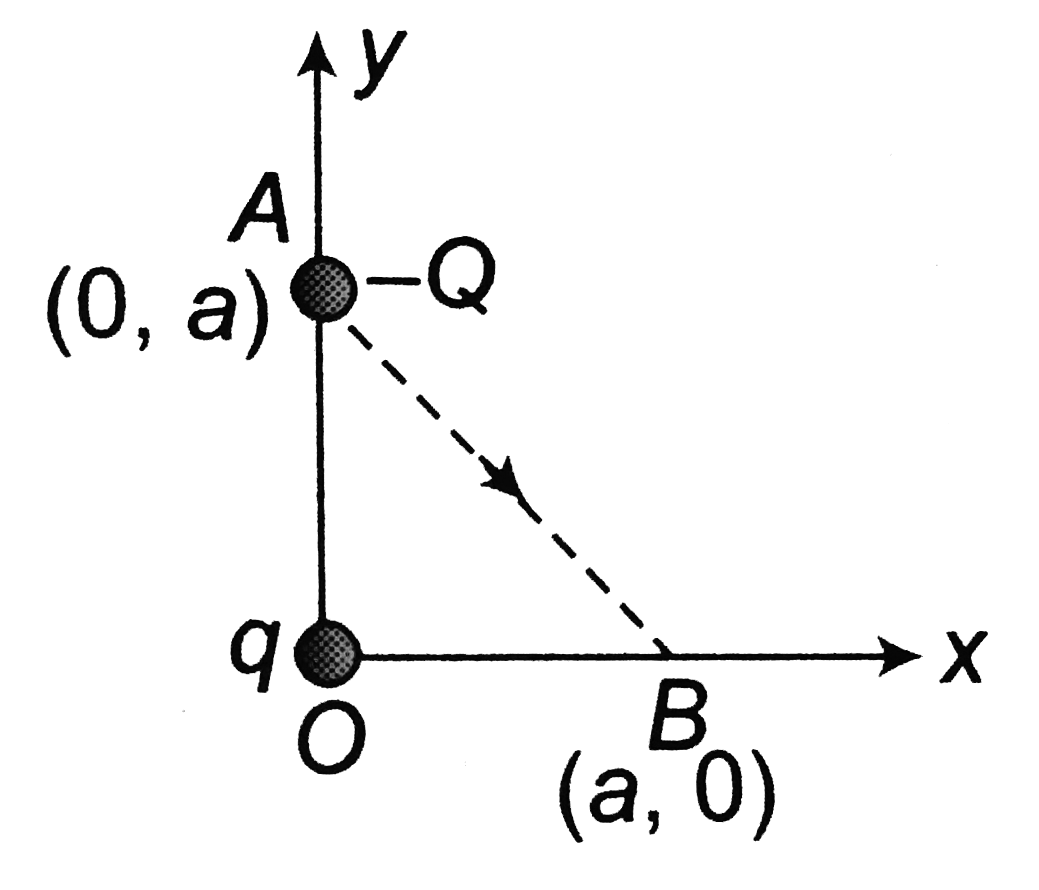
View SolutionAs per this diagram a point charge +q is placed at the origin O. Work done in taking another point charge −Q from the point A(0,a) to another point B(a,0) along the staight path AB is:

View Solution
Knowledge Check
In the question number 4, work done in bringing a charge of 4×10−9 C form infinity to that point is
A2.4×10−4JB1.8×10−4JC3.2×10−5JD4.1×10−5JThe potential at a point due to charge of 5×10−7C located 10 cm away is
A3.5×105VB3.5×104VC4.5×104VD4.5×105V
A point charge +q is placed at origin O. The work done in taking another point charge -q from the point A to point B along the circular arc as shown in the figure is
_PHY_E05_122_Q01.png) .
.
View Solution(a) Calculate the potential at a point P due to a charge of 4×10−7C located 9 cm away.
(b) Hence obtain the work done in bringing a charge of 2×10−9C from infinity to the point P. Does the answer depend on the path along which the charge is brought ?
View SolutionA point charge q1=−1.0μC is held stationary at the origin. A second point charge q2=+2.0μC moves from the point (1.0m,0,0) to (2.0m,0,0). How much work is done by the electric force on q2?
View SolutionA point charge q1=−5.8μC is held stationary at the origin. A second point charge q2=+4.3μC moves from the point (0.26m0,0) to (0.38m,0,0). How much work is done by the electric force on q2?
View Solution(i) Can two equaipotential surfaces intersect each other ? Give reasons
(ii) Two charges −qand+q are located at point A (0, 0 -a) and B(0, 0, +a) respectively. How much work is done in moving a test charge from point P(7,0,0) to Q(−3,0,0) ?
View Solution(i) Find potential at point A and B sue to the small charge - system fixed near origin. (Distance between the charges is negligible).
(ii) Find work done to bring a test charge q0 from point A to point B, slowly. All parameters are in S.I. units.
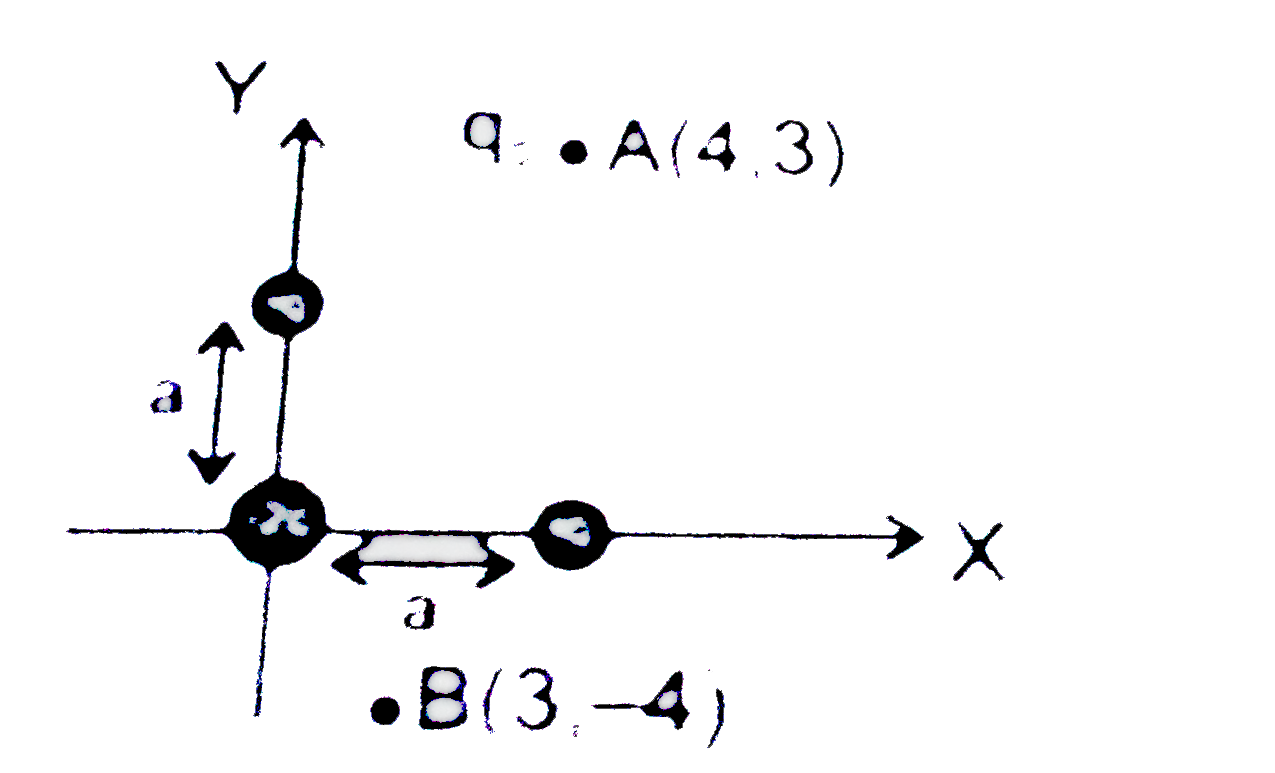
View Solution
