A straight wire carrying a current of 12A is bent into a semicircular arc of radius 2⋅0cm as shown in figure. Consider the magnetic field →B at the centre of arc.
(a) What is the magnetic field due to the staight segments?
(b) In what way the contribution to →B from the semicircle differs from that of a circular loop and in what way does it resemble?
(c) Would your answer be different if the wire were bent into a semicircle arc of the same radius but in the opposite way as shown in figure
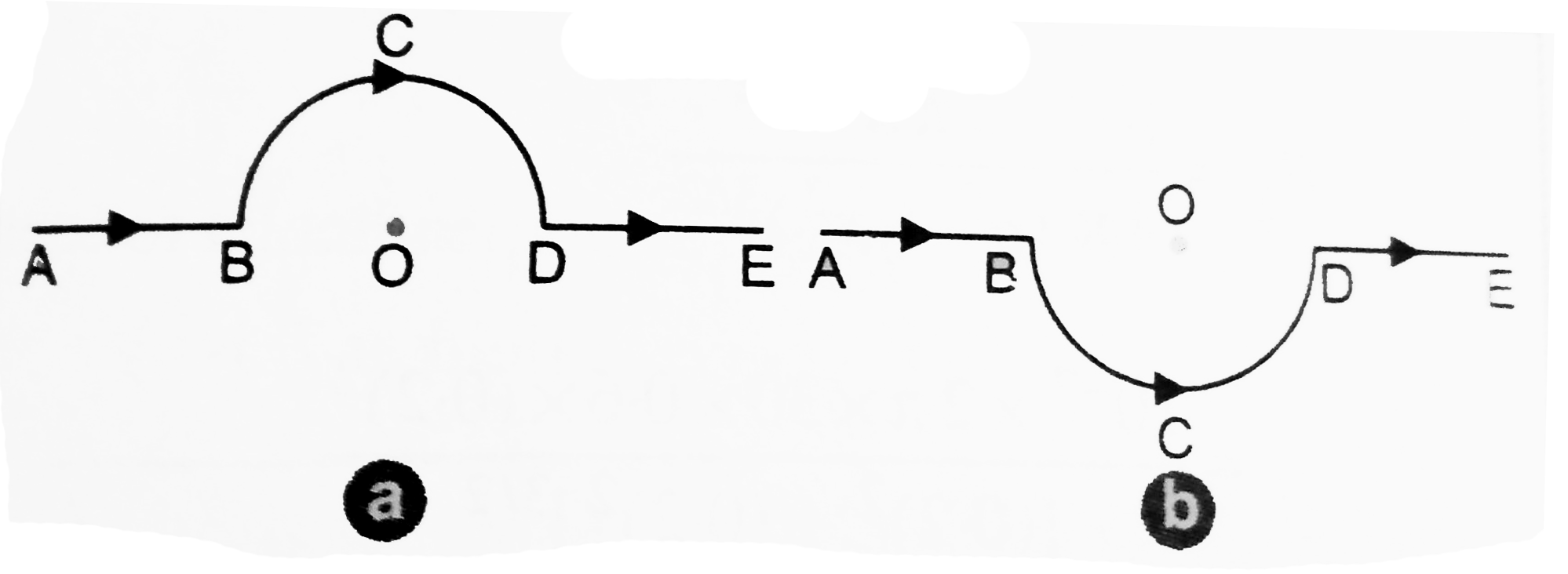
(a) dl and r for each element of the straight segments are parallel. Therefore, dl×r=0. Straight segments do not contribute to |B|.
(b) For all segments of the semicircular arc, dlx×r are all parallel to each other (into the plane of the paper). All such contributions add up in magnitude. Hence direction of B for a semicircular arc is given by the right-hand rule and magnitude is half that of a circular loop. Thus B is 1.9×10–4T normal to the plane of the paper going into it. (c) Same magnitude of B but opposite in direction to that in (b).
Topper's Solved these Questions
Similar Questions
A straight wire carrying a current of 13 A is bent into a semi-circular arc of radius 2 cm as shown in figure. The magnetic field is 1.5×10−4T at the centre of arc, then the magnetic field due to straight segment is

View SolutionA straight wire carrying a current of 13 A is bent into a semi-circular arc of radius 2 cm as shown in figure. The magnetic field is 1.5×10−4T at the centre of arc, then the magnetic field due to straight segment is

View Solution
Knowledge Check
A straight wire carrying a current 10A is bent into a semicircular arc of radius 5cm. The magnitude of magnetic field at the center is
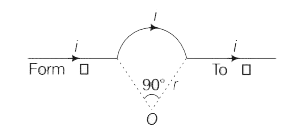
A1.5×10−5TB3.14×10−5TC6.28×10−5TD19.6×10−5TA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is
 Aμ0i4rBμ0i2rCμ0i2πr(π+1)Dμ0i2πr(π−1)
Aμ0i4rBμ0i2rCμ0i2πr(π+1)Dμ0i2πr(π−1)What is the magnetic field at the centre of arc in the figure below?
 Aμ04π⋅2ir[√2+π]Bμ04π⋅2ir[√2+π4]Cμ04π⋅ir[√2+π]Dμ04π⋅ir[√2+π4]
Aμ04π⋅2ir[√2+π]Bμ04π⋅2ir[√2+π4]Cμ04π⋅ir[√2+π]Dμ04π⋅ir[√2+π4]
A straight wire carrying a current of 5 A is bent into a semicircular arc of radius 2 cm as shown in the fig. Find the magnitude and direction of the magnetic field at the centre of the arc.

View SolutionA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is :

View SolutionMagnetic field at the centre of a circular loop & arc
View SolutionA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is

View SolutionA straight wire carrying a current of 12 A is bent into a semi-circular are of radius of2.0 cm as shown in the adjoining figure (a). Consider the magnetic field B at the centre of the arc. Would your answer be different if the wire were bent into a semi-circular arc of the same radius but in the opposite way a shown in figure (b)?

View Solution
