A bob of mass m is suspended by a light string of length L. It is imparted a horizontal velocity v0 at the lowest point A such that it completes a semi-circular trajectory in the vertical plane with the string becoming slack on reaching the topmost point C, figure, Obtain an expression for (i) v0 (ii) the speeds at points B and C, (ii) the ration of kinetic energies (KB/KC) at B and C.
Comment on the nature of the trajectory of the bob after it reahes the poing C.
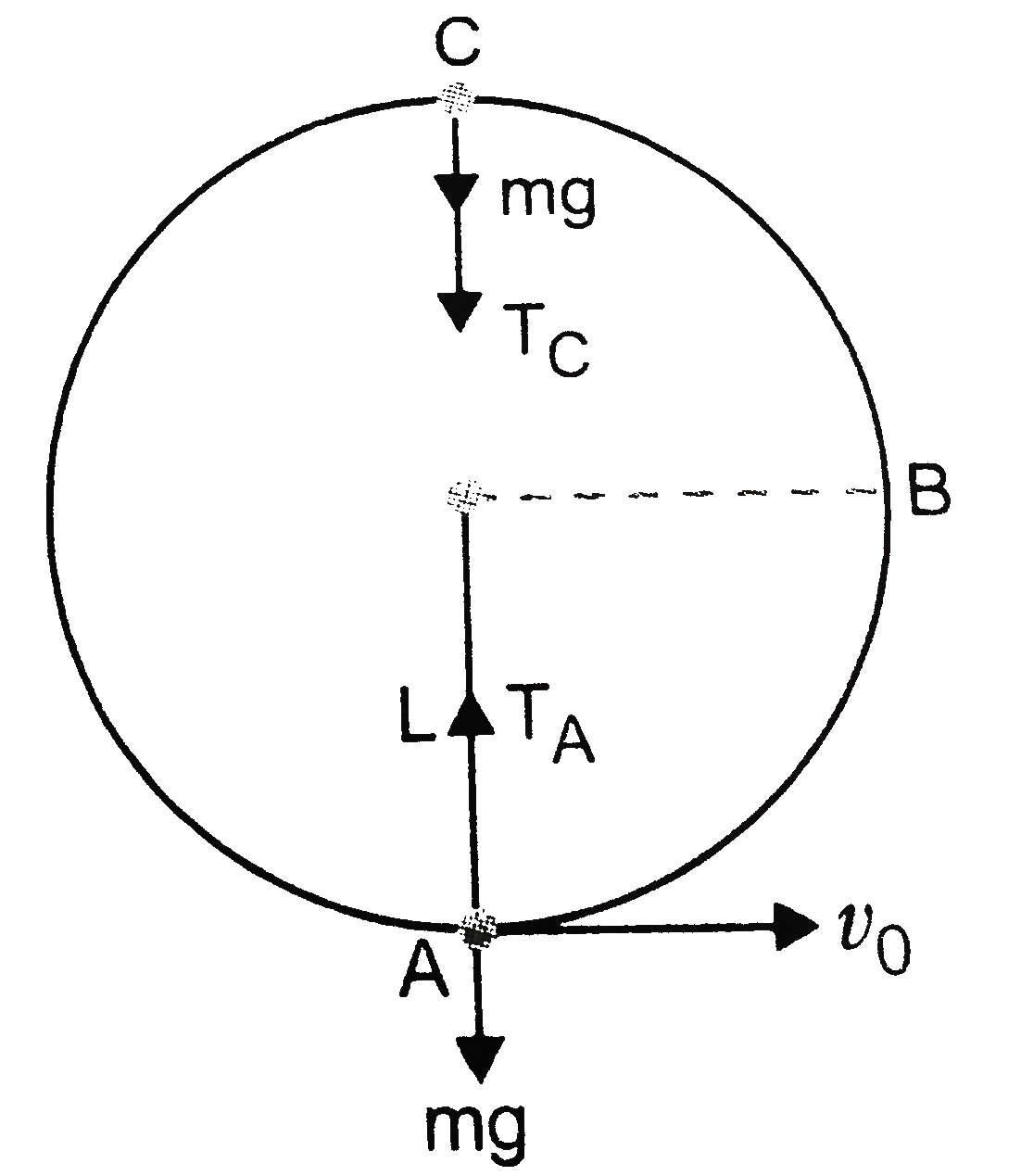
i) There are two external forces on the bob: gravity and the tension (T) in the string. The latter does no work since the displacement of the bob is always normal to the string. The potential energy of the bob is thus associated with the gravitational force only. The total mechanical energy E of the system is conserved. We take the potential energy of the system to be zero at the lowest point A. Thus, at A:
E=12mv20
TA−mg=mv20L [Newton's Second Law]
Where TA isthe tension in the string at A. At the highest point C, the string slackens, as the tension in the string (Tc) becomes zero.
Thus, at C
E=12mvc+2mgL
mg=mv2cL [Newton's Second law]
where vc is the speed at C.
E=5mgL
Equatting this to the energy at A
52mgL=m2v20
or , v0=√5gL
ii) It is clear from Eq. (6.14)
vC=√5gL
At B, the energy is
12mv2B+mgL
Equating this to the energy at A and employing the result from (i), namely v20=5gL
12mv2B+mgL=12mv20
52mgL
∴vB=√3gL
iii) The ratio of the kinetic energies at B and C is:
KBKC=12mv2B12mv2C = 31
At point C, the string becomes slack and the velocity of the bob is horizontal projection akin of a cliff. Otherwise the bob will continue on its circular path and complete the revolution.
Topper's Solved these Questions
Similar Questions
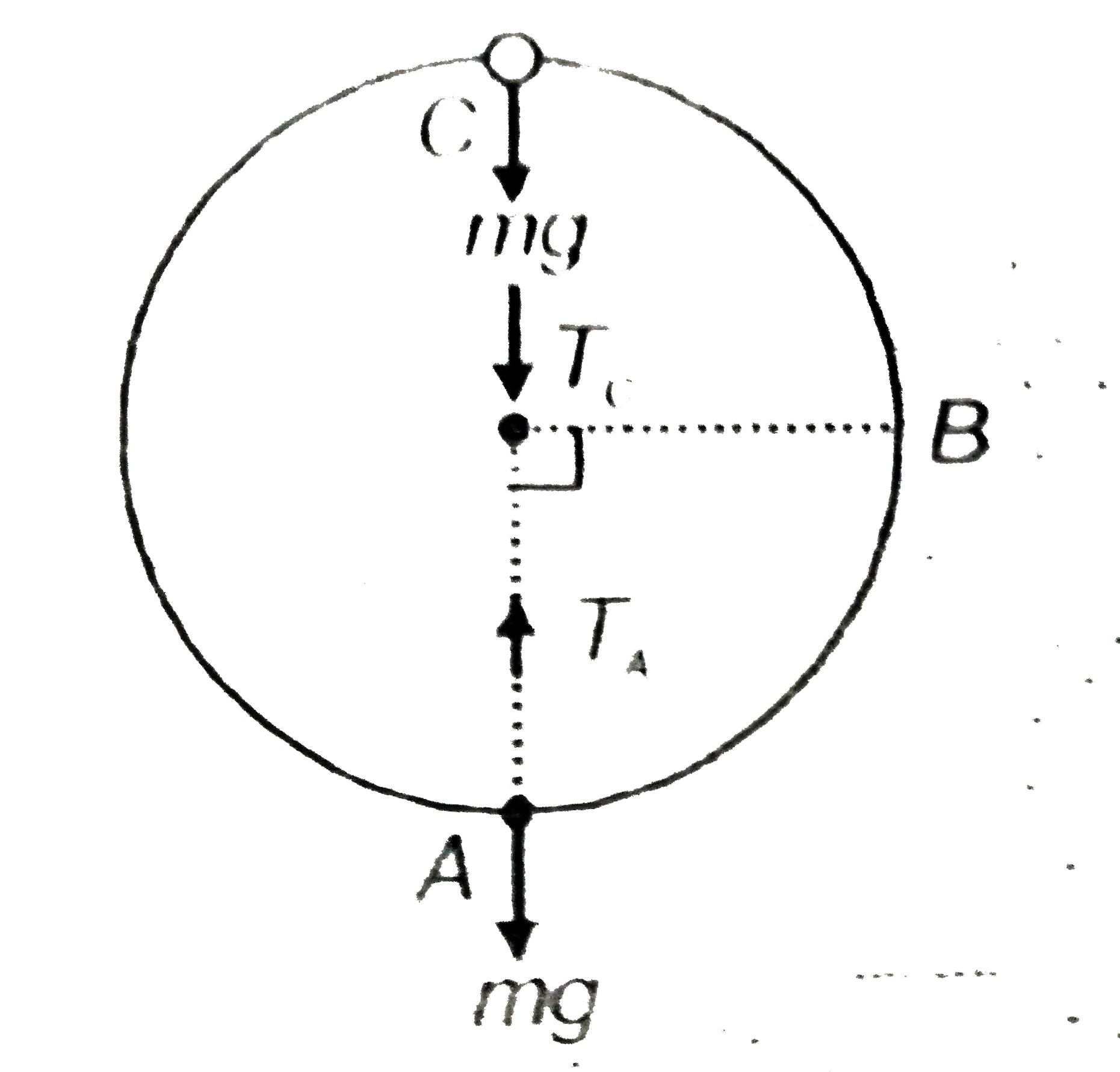
A bob of mass ma suspended by a massle3ss inextensible string is rotated in a vertical circle of radius r as shoen in the figure. Obtain an expenssion for speeds and kinetic energies at A, B and C, Comment on the nature of trajectory of the bob after it reaches the point C.
 View Solution
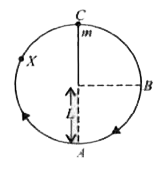
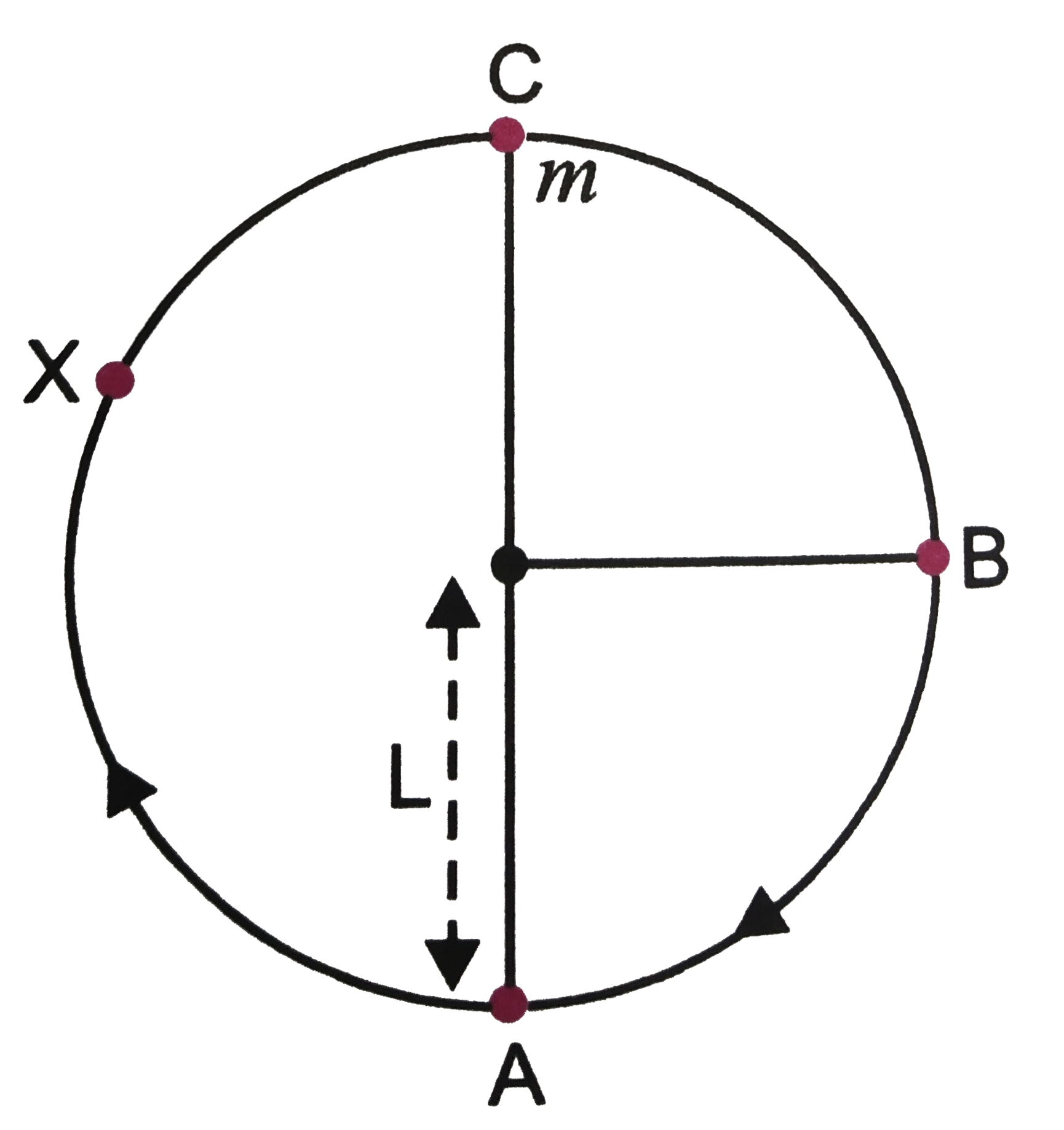
View SolutionA bob of mass m suspended by a light string of length L is whirled into a vertical circle as shown in figure. What will be X the trajectory of the particle if the string is cut at
(a) Point B
(b) Point C
(c) Point X
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
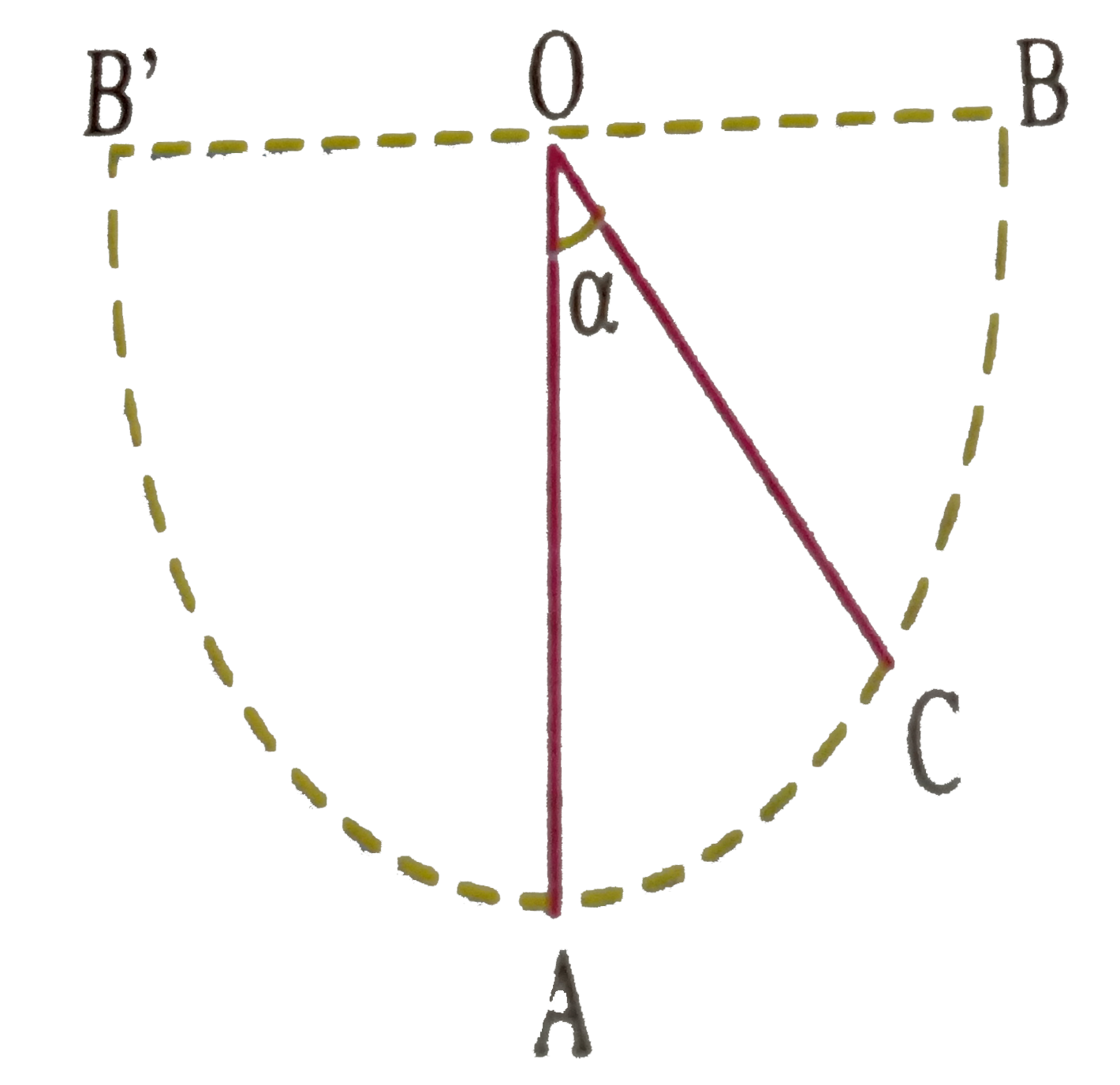
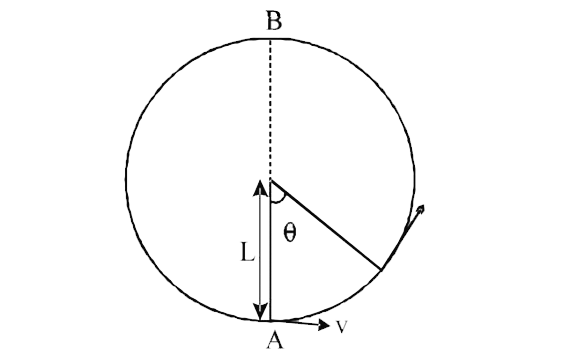
A bob of mas M is suspended by a massless string of length L. The horizontal velocity v at position A is just sufficient to make it reach the point B. The angle θ at which the speed of the bob is half of that at A, satisfies.
 .Aθ=π4Bπ4<θ<π2Cπ2<θ<3π4D3π4<θ<π
.Aθ=π4Bπ4<θ<π2Cπ2<θ<3π4D3π4<θ<π - Question 2 - Select One
A mass m is hanging by a string of length l. The velocity v0 which must be imparted to it to just reach the top is
A√3glB√4glC√5glD√6gl - Question 3 - Select One
The maximum velocity at the lowest point, so that the string just slack at the highest point in a vertical circle of radius l.
A√glB√3glC√5glD√7gl
A body is suspended by a string of length l is projected with horizontal velocity V such that it does not complete the motion in vertical circles.Then the height at which tension becomes is zero is given by?
View SolutionA bob of mass m suspended by a light string of length L is whirled into a vertical circle as shown in figure . What will be the trajectory of the particle if the string is cut at
(a) Point B ? (b) Point C? (c) Point X?
 View Solution
View SolutionA bob of mass m suspended by a light string of length L is whirled into a vertical circle as shown in figure . What will be the trajectory of the particle if the string is cut at
(a) Point B ? (b) Point C? (c) Point X?
 View Solution
View SolutionA bob of mass M is suspended by a massless string of length L. The horizontal velocity v at position A is just sufficient to make it reach the point B. The angle θ at which the speed of the bob is half of that at A, satisfies
 View Solution
View SolutionThe maximum velocity at the lowest point, so that the string just slack at the highest point in a vertical circle of radius l.
View Solution

