A body is moving undirectionally under the influence of a source of constatn power. It displacement in time t is proportional to (i) t1/2 (ii) t (iii) t3/2 (iv) t2
t1/2
t
t3/3
t2
To solve the problem step by step, we need to analyze the relationship between power, force, velocity, and displacement in the context of a body moving under constant power.
Step 1: Understanding the relationship between power, force, and velocity
Power (P) is defined as the product of force (F) and velocity (v):
P=F⋅v
Since the power is constant, we can denote it as P=K, where K is a constant.
Step 2: Relating force to mass and acceleration
Force can also be expressed in terms of mass (m) and acceleration (a):
F=m⋅a
And acceleration can be expressed as the derivative of velocity with respect to time:
a=dvdt
Thus, we can rewrite the power equation as:
K=m⋅v⋅dvdt
Step 3: Rearranging the equation
Rearranging the equation gives us:
m⋅v⋅dvdt=K
Now, we can separate variables:
m⋅vdv=Kdt
Step 4: Integrating both sides
Next, we integrate both sides. The left side will be integrated with respect to v from 0 to v, and the right side will be integrated with respect to t from 0 to t:
∫v0m⋅vdv=∫t0Kdt
The left side becomes:
m⋅v22∣∣∣v0=mv22
The right side becomes:
K⋅t∣∣∣t0=Kt
Thus, we have:
mv22=Kt
Step 5: Solving for velocity
From the equation above, we can solve for v2:
v2=2Ktm
Taking the square root gives us:
v=√2Km⋅t1/2
Let’s denote √2Km as a new constant k′:
v=k′⋅t1/2
Step 6: Relating velocity to displacement
Since velocity v is the derivative of displacement x with respect to time t:
v=dxdt
We can substitute our expression for v:
dxdt=k′⋅t1/2
Step 7: Integrating to find displacement
Now we integrate both sides to find x:
dx=k′⋅t1/2dt
Integrating gives:
∫x0dx=k′∫t0t1/2dt
The left side becomes:
x
The right side becomes:
k′⋅(t3/23/2)=2k′3t3/2
Thus, we have:
x=2k′3t3/2
Conclusion
From the above steps, we conclude that the displacement x is proportional to t3/2:
x∝t3/2
Final Answer
The displacement in time t is proportional to t3/2.
Power (P) is defined as the product of force (F) and velocity (v):
P=F⋅v
Since the power is constant, we can denote it as P=K, where K is a constant.
Force can also be expressed in terms of mass (m) and acceleration (a):
F=m⋅a
And acceleration can be expressed as the derivative of velocity with respect to time:
a=dvdt
Thus, we can rewrite the power equation as:
K=m⋅v⋅dvdt
Rearranging the equation gives us:
m⋅v⋅dvdt=K
Now, we can separate variables:
m⋅vdv=Kdt
Next, we integrate both sides. The left side will be integrated with respect to v from 0 to v, and the right side will be integrated with respect to t from 0 to t:
∫v0m⋅vdv=∫t0Kdt
The left side becomes:
m⋅v22∣∣∣v0=mv22
The right side becomes:
K⋅t∣∣∣t0=Kt
Thus, we have:
mv22=Kt
From the equation above, we can solve for v2:
v2=2Ktm
Taking the square root gives us:
v=√2Km⋅t1/2
Let’s denote √2Km as a new constant k′:
v=k′⋅t1/2
Since velocity v is the derivative of displacement x with respect to time t:
v=dxdt
We can substitute our expression for v:
dxdt=k′⋅t1/2
Now we integrate both sides to find x:
dx=k′⋅t1/2dt
Integrating gives:
∫x0dx=k′∫t0t1/2dt
The left side becomes:
x
The right side becomes:
k′⋅(t3/23/2)=2k′3t3/2
Thus, we have:
x=2k′3t3/2
From the above steps, we conclude that the displacement x is proportional to t3/2:
x∝t3/2
The displacement in time t is proportional to t3/2.
Topper's Solved these Questions
Similar Questions
A body is initially at rest. It undergoes one-dimensional motion with constant acceleration. The power delivered to it at time t is proportional to (i) t1/2 (ii) t (iii) t3/2 (iv) t2
View SolutionA body starts from rest and acquires velocity V in time T. The instantaneous power delivered to the body in time 't' proportional to
View Solution
Knowledge Check
A particle moves rectilinearly. Its displacement x at time t is given by x2=at2+b where a and b are constants. Its acceleration at time t is proportional to
A1x3B1x−1x2C−1x2D1x−t2x3
A body is moving with variable acceleartion (a) along a straight line. The average acceleration of body in time interval t1 to t2 is :-
View SolutionIdentify the numerical coefficients of terms (other than constants) in the following expressions : (i) 5−3t2 (ii) 1+t+t2+t3 (iii) x+2xy+3y (iv) 100m+100n
View SolutionRelation between displacement x and time t is x=2−5t+6t2, the initial acceleration will be :-
View SolutionThe displacement 'x' (in meter) of a particle of mass 'm' (in kg) moving in one dimension under the action of a force is released to time 't' (in sec) by t2=√x+2. The displacement of the particle when its velocity is zero will be.
View SolutionVelocity of a particle varies with time as v=4t. Calculate the displacement of particle between t=2 to t=4 sec.
View SolutionA body is moved along a straight line by a machine delivering a power proportional to time (P∝t). Then, match the following.
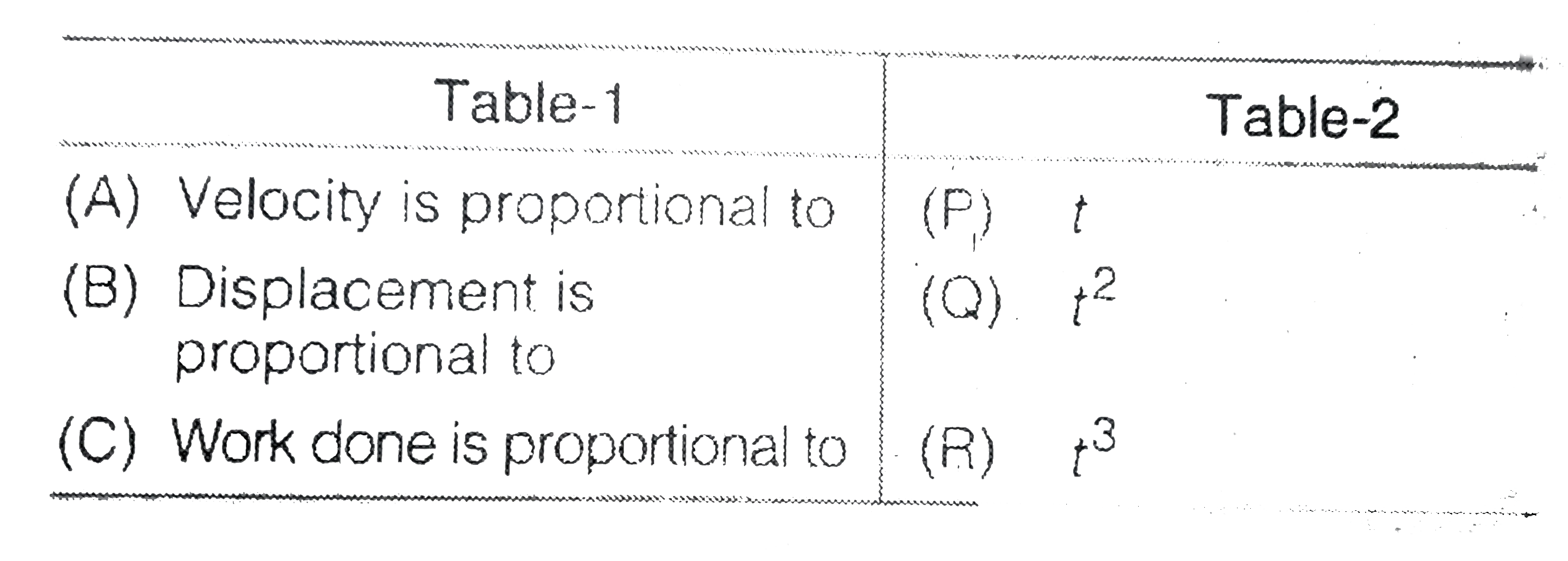
View SolutionA body is projected up with velocity u. It reaches a point in its path at times t1 and t2 seconds from the time of projection. Then (t1+t2) is
View Solution
