Width of a river is 60m . A swimmer wants to cross the river such that he reaches from A to B directly. Point B is 45m ahead of line AC (perpendicular to river) Assume speed of river and speed of swimmer as equal. Swimmer must try to swim at angle θ with line AC .If the value of θ (in degrees) is N2 .Find N
Step by step video & image solution for Width of a river is 60m . A swimmer wants to cross the river such that he reaches from A to B directly. Point B is 45m ahead of line AC (perpendicular to river) Assume speed of river and speed of swimmer as equal. Swimmer must try to swim at angle theta with line AC .If the value of theta (in degrees) is N^(2) .Find N by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
Velocity of man w.r.t river is equal to velocity at river value of θ for which man reaches directly at B
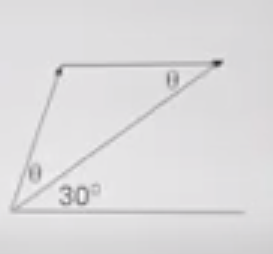 View Solution
View SolutionA swimmer wants to cross a river from point A to point B. Line AB makes an angle of 30∘ with the flow of river. Magnitude of velocity of the swimmer is same as that of the river. The angle θ with the line AB should be _________ ∘, so that the swimmer reaches point B.
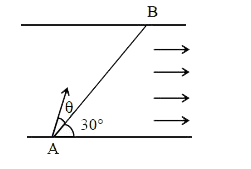 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
man wants to swim across a river of which 200 m along the shortest path . If the speed of river stream is 3kmh−1 and speed of swimmer in still water is 5kmh−1 , then the time of crossing the river is
A10 minB15 minC3 minD6 min - Question 2 - Select One
A swimmer wands to cross a river and reach point B directly from A. The speed of the wimmer in still river and that of river flow are same. The dotted line AN is normal to flow direction of river. For swimmer to reach point B, the angle his velocity relative to river will make with line AN is given by θ. then the value of θ is :
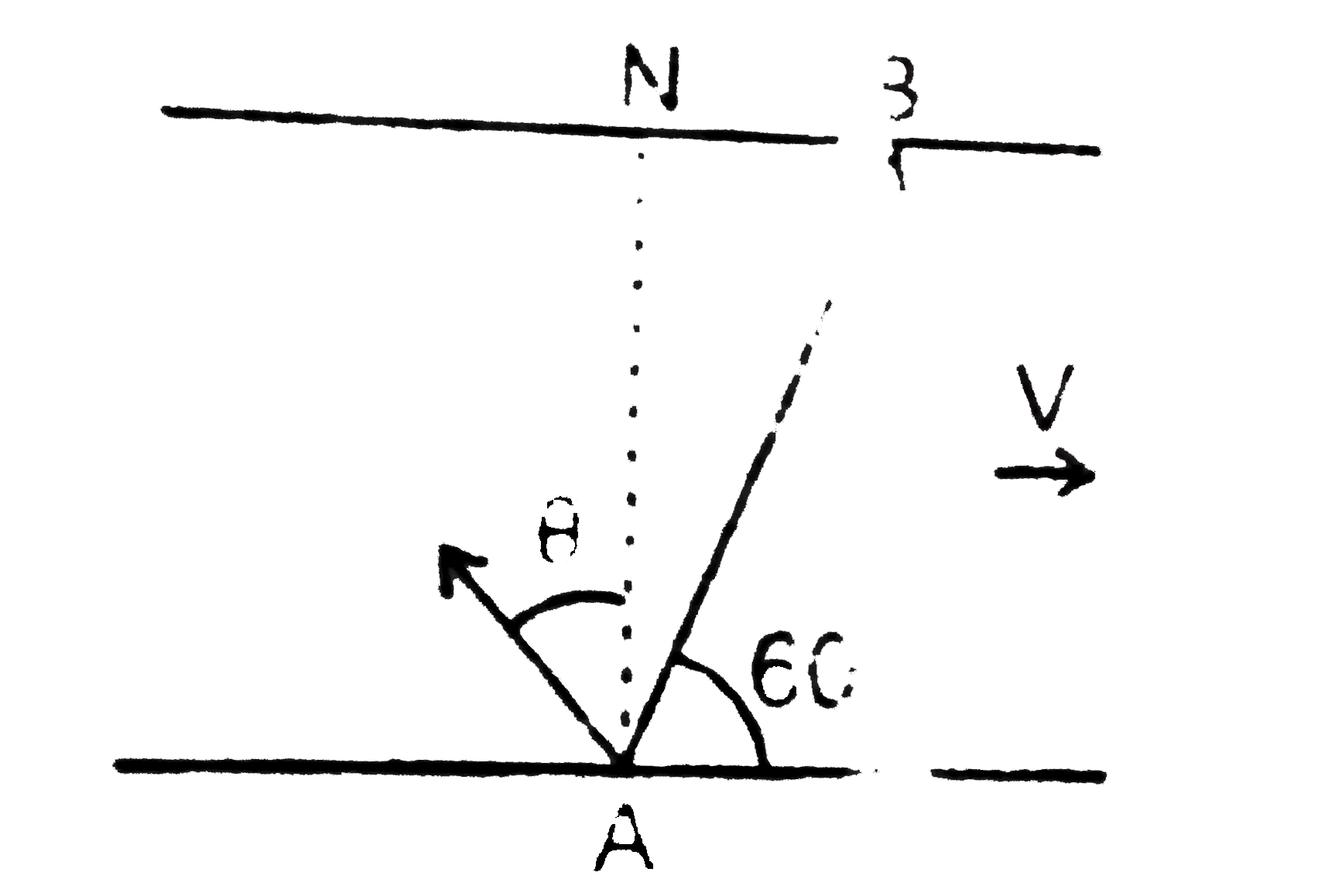 A60∘B30∘C45∘D0∘
A60∘B30∘C45∘D0∘ - Question 3 - Select One
A swimmer crosses the river along the line making an angle of 45∘ with the direction of flow. Velocity of the river is 5 m/s. Swimmer takes 6 seconds to cross the river of width 60 m. The velocity of the swimmer with respect to water will be :
A10m/sB12m/sC5√5m/sD10√2m/s
A swimmer wants to cross a 600 m wide river . If the speed of swimmer is 12 km/hr in still water and speed of river is 6km/hr , along what direction the swimmer must strike in order to cross the river straight ? Also, calculate his resultant velocity and time taken by him to do so .
View SolutionA swimmer wishes to cross a river 500m width flowing at a rate u. his speed w.r.t. still water is v. for this he makes an angle θ with the vertical as shown in the given figure then:
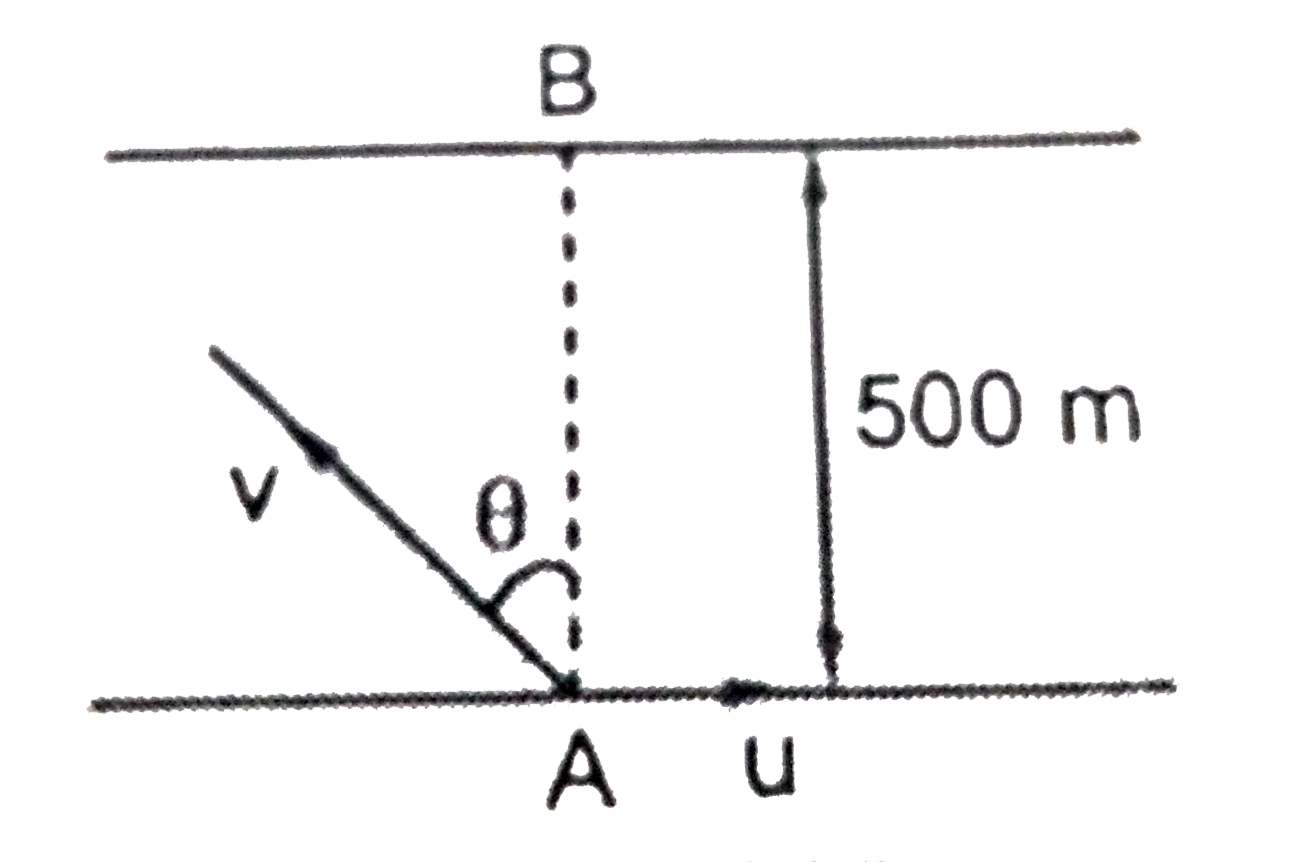 View Solution
View SolutionA swimmer wishes to cross a 500−m river flowing at 5kmh−1. His speed with respect to water is 3kmh−1. The shortest possible time to cross the river is.
View SolutionA man who can swim at a velocity v relative to water wants to cross a river of width b, flowing with a speed u.
View SolutionA swimmer can swim with 5ms−1 in still water. He wants to cross a river having width 200m heading perpendicular to the flow. Speed of flow is uniformly increasing from zero at side to 3ms−1 at mid from both side of the river. Then the time taken by the swimmer to cross the river will be
View Solution
