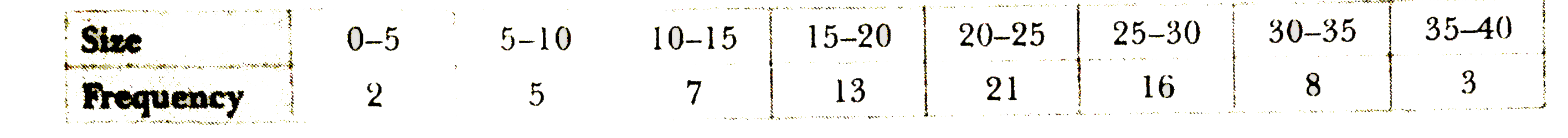Consider the two series of observations A and B as follows:
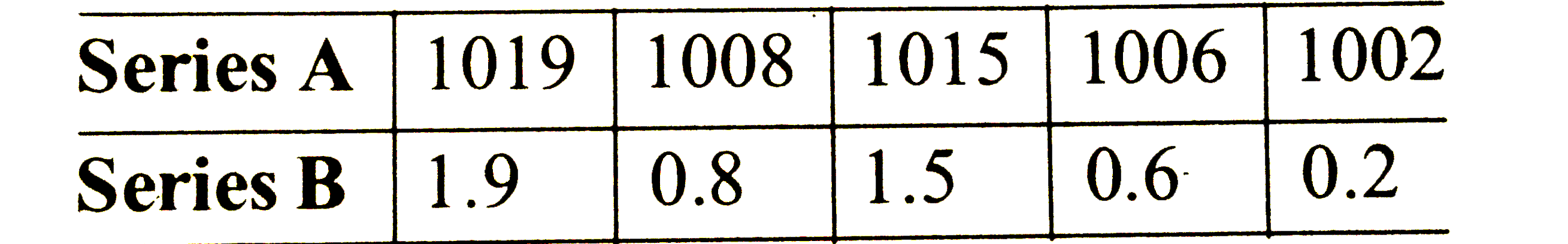
If the standard deviation of the Series A is √28, then what is the standard deviation of the Series B?
A
3.8
B
√0.38
C
0.38
D
√38
Text Solution
The correct Answer is:B
Standard deviation (series B) :
=
⎷15(1.92+0.82+1.52+0.62+0.22)+(1.9+0.8+1.5+0.6+0.25)2
=√6.95−1=√1.38−1
=√0.38
|
Topper's Solved these Questions
- SETS, RELATIONS, FUNCTIONS AND NUMBER SYSTEM BOOK - NDA PREVIOUS YEARSCHAPTER - SETS, RELATIONS, FUNCTIONS AND NUMBER SYSTEM EXERCISE - MCQ
271 Videos
- TRIGONOMETRY - RATIO & IDENTITY , TRIGONOMETRIC EQUATIONSBOOK - NDA PREVIOUS YEARSCHAPTER - TRIGONOMETRY - RATIO & IDENTITY , TRIGONOMETRIC EQUATIONSEXERCISE - MCQ
238 Videos
Similar Questions
Knowledge Check
Arithmetic mean of 10 observations is 60 and sum of squares of deviations from 50 is 5000, what is the standard deviation of the observation
A20B21C22.36D24.7In a series of 2n observations, half of them are equal to a2 and the remaining half are equal to −a2. If the standard deviation of the observation is 2, then |a| is equal to
A1nB√2C2D√2n
The standard deviation of the observations 22, 26, 28, 20, 24, 30 is
View SolutionConsider three observations a, b and c such that b=a+c. If the standard deviation of a+2, b+2, c+2 is d, then which of the following is true ?
View SolutionUsing step-deviation method, calculate standard deviation of the following series:
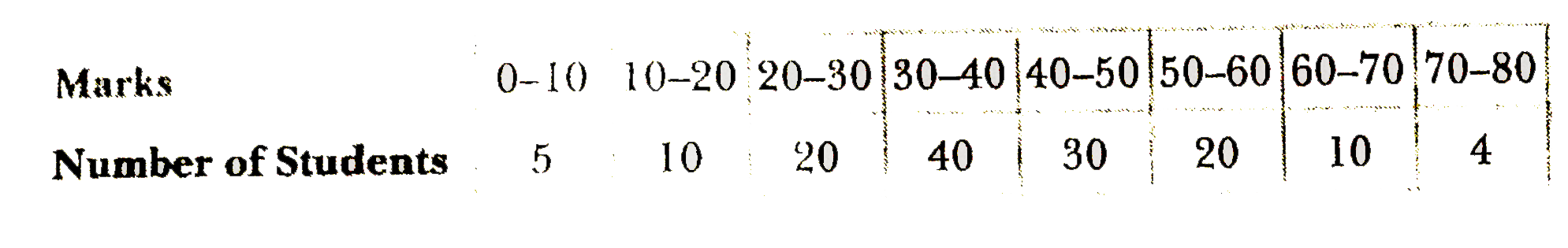
View SolutionUsing step-deviation method, calculate standard deviation of the following series:
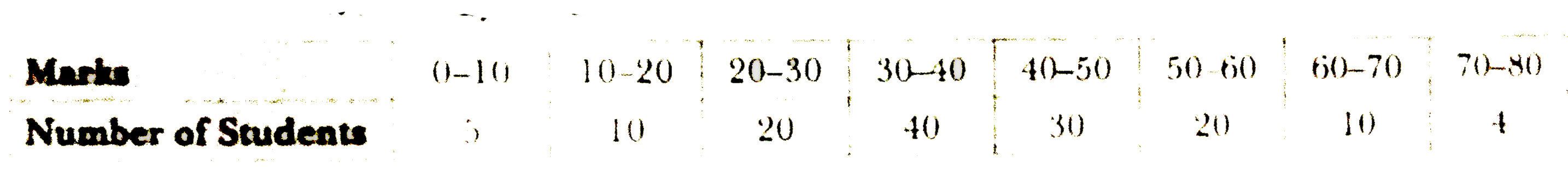
View SolutionCalculate the arithmetic average and the standard deviation of the following series :
View SolutionIn a series of 2n observations, half of them equals to a and remaining half equals to -a. If the standard deviation of the observations is 2, then find |a|.
View Solution