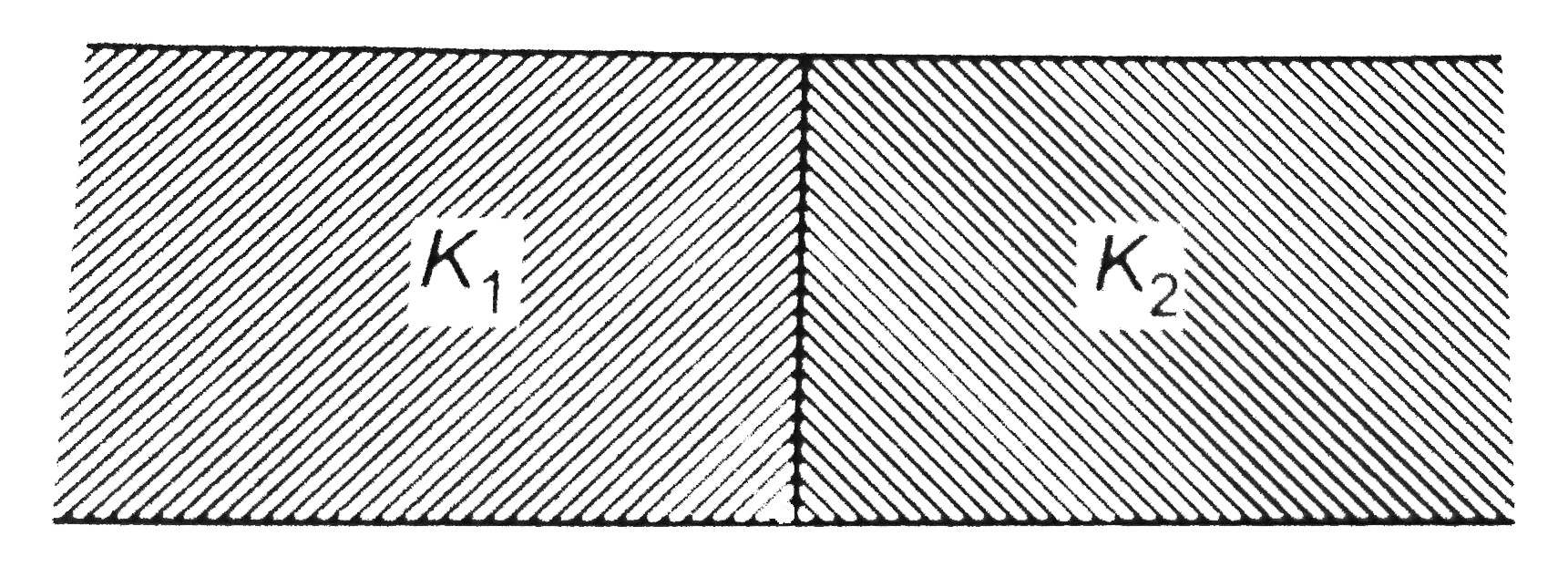
Two dielectric slabs of dielectric constants K1andK2 are filled in between the two plates, each of area A, of the parallel plate capacitor as shown in the figure. Find the net capacitance of the capacitor.
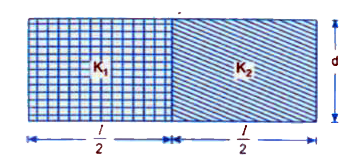
The arrangement is equivalent to two capacitors joined in parallel where area of plates of either capacitor is A2. Thus,
C1=K1ε0(A2)d=K1ε0A2d
and C2=K2ε0(A2)d=K2ε0A2d
∴ Net capacitance of the capacitor
C=C1+C2=K1ε0A2d+K2ε0A2d=ε0Ad(K1+K22).
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - U-LIKE SERIESCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - LONG ANSWER QUESTIONS-I
64 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - U-LIKE SERIESCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - LONG ANSWER QUESTIONS-II
13 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - U-LIKE SERIESCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - VERY SHORT ANSWER QUESTIONS
40 Videos
- ELECTROMAGNETICE INDUCTIONBOOK - U-LIKE SERIESCHAPTER - ELECTROMAGNETICE INDUCTIONEXERCISE - Self Assessment Test Section -D
1 Videos
- EXAMINATION PAPER 2020 (SOLVED)BOOK - U-LIKE SERIESCHAPTER - EXAMINATION PAPER 2020 (SOLVED)EXERCISE - SECTION D
6 Videos
Similar Questions
Two thin dielectric slabs of dielectric constants K1 and K2(K1<K2) are inserted between plates of a parallel plate capacitor, as shown in the figure. The variation of electric field E between the plates with distance d as measured from plate P is correctly shown by

View SolutionTwo dielectric slabs of constant K1 and K2 have been filled in between the plates of a capacitor as shown below. What will be the capacitance of the capacitor
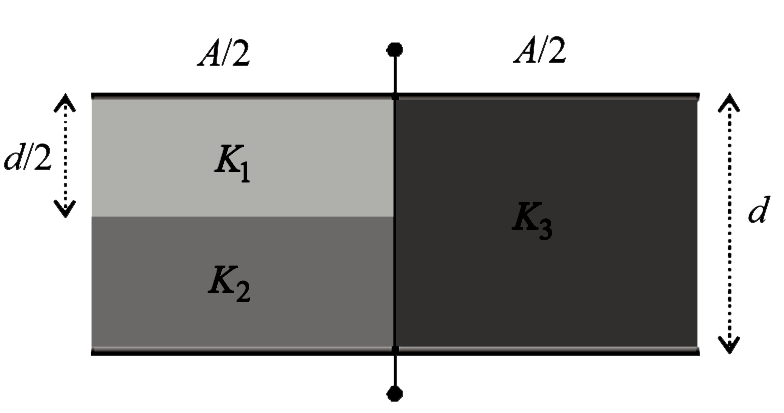
View Solution
Knowledge Check
Two thin dielectric slabs of dielectric constants K1 and K2(K1<K2) are inserted between plates of a parallel plate capacitor, as shown in the figure. The variation of electric field E between the plates with distance d as measured from plate P is correctly shown by
 A
A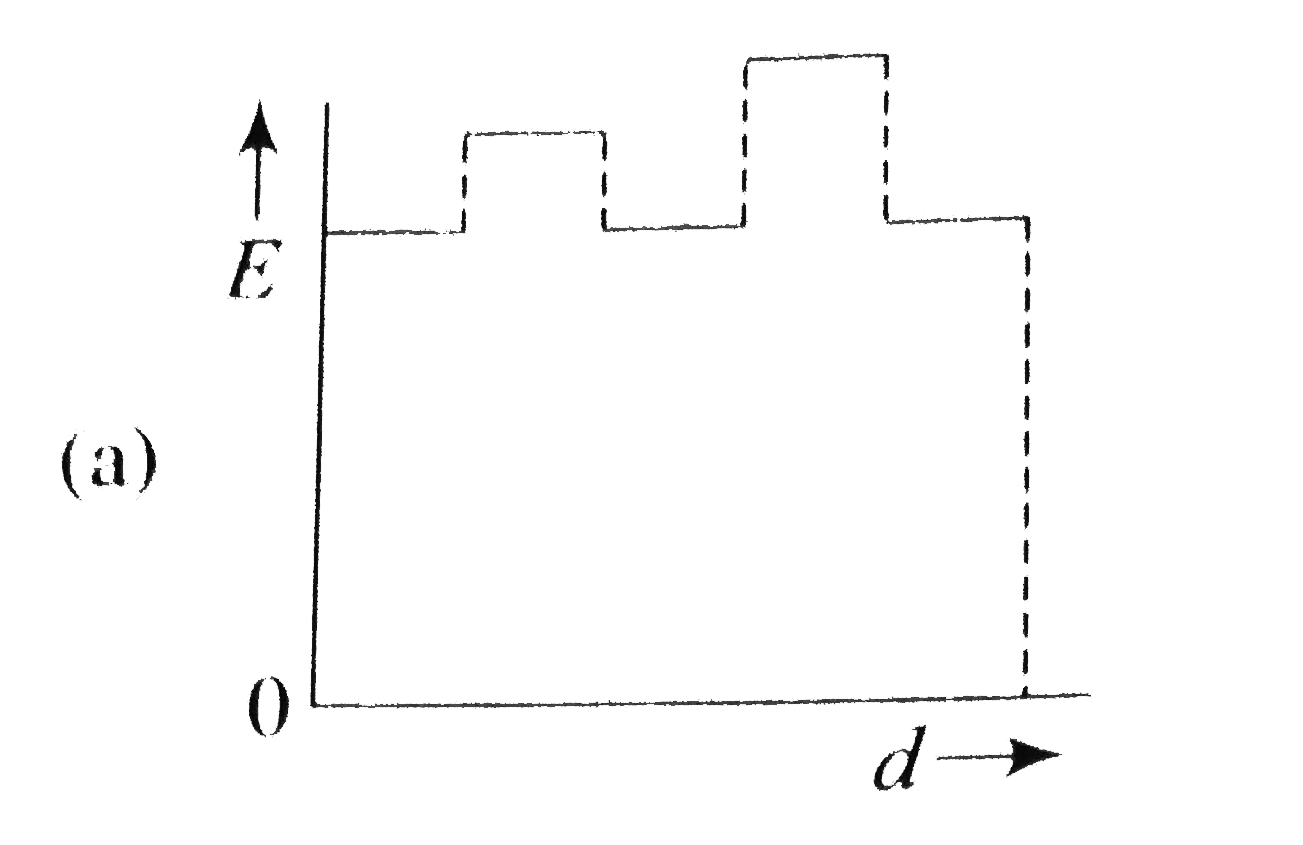 B
B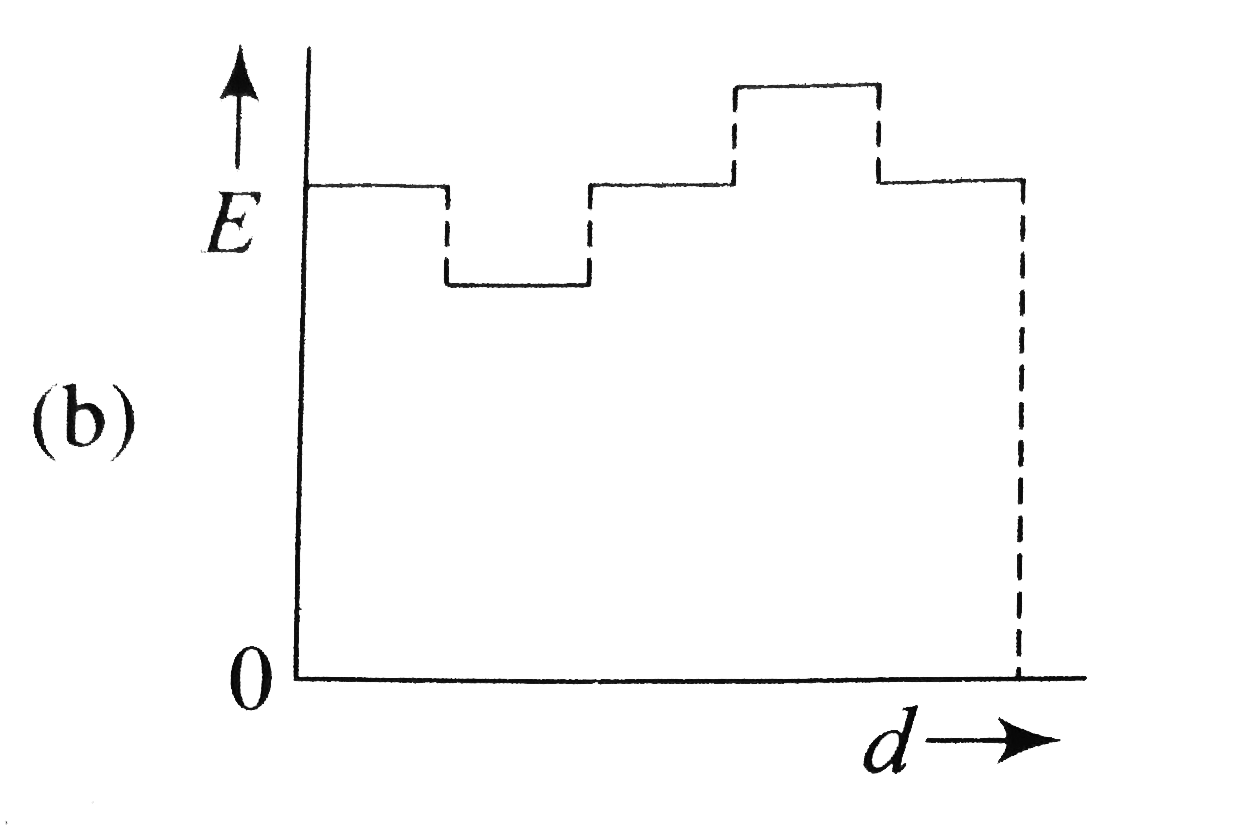 C
C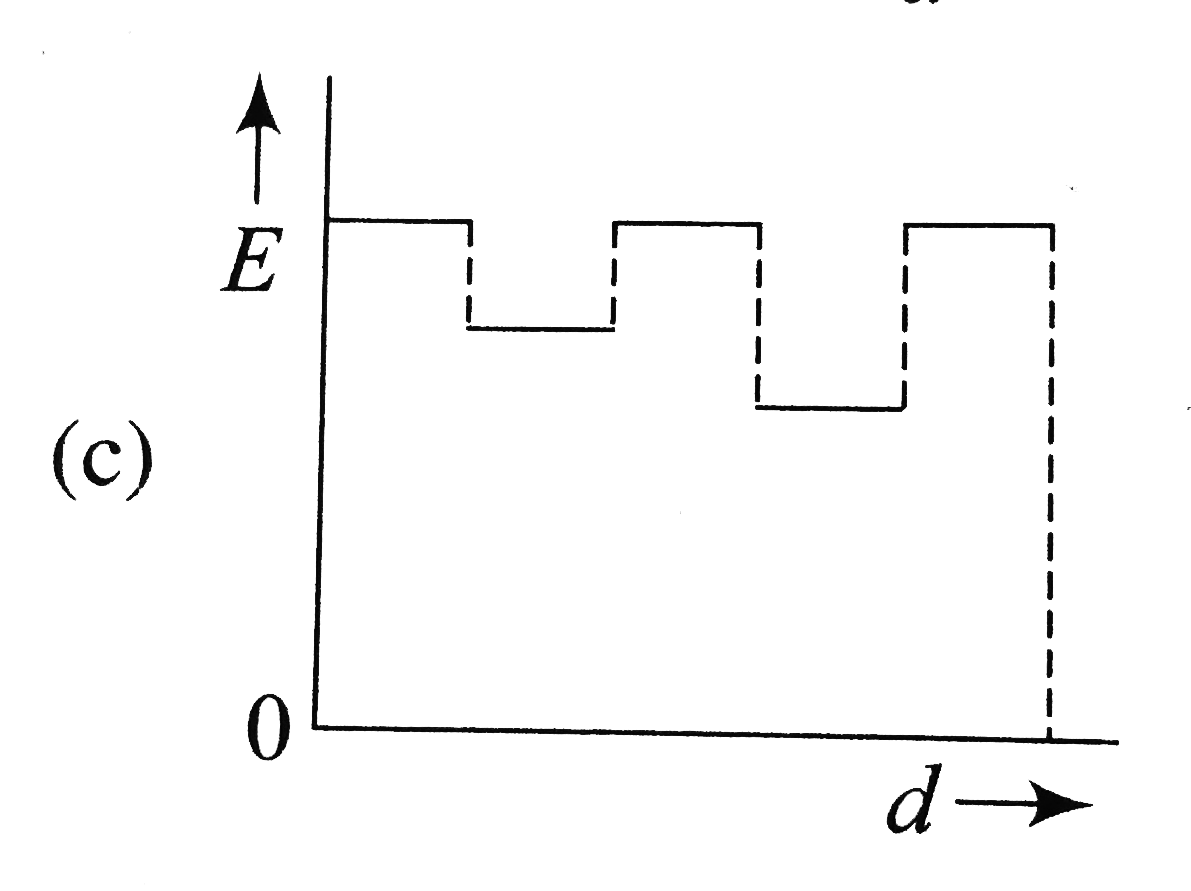 D
D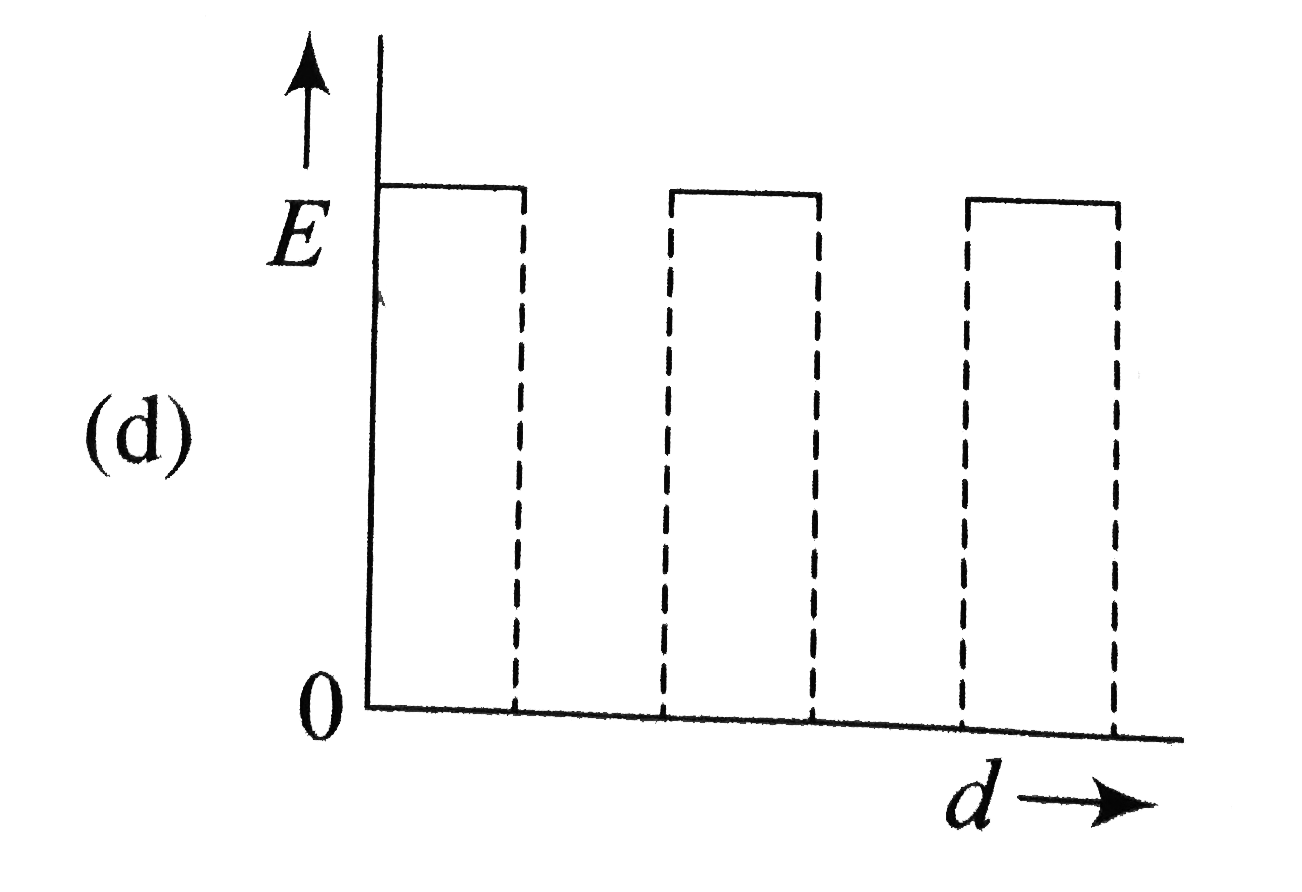
parallel plate capacitor has capacitance C when no dielectric between thw plates. Now a slab of dielectric constant K, having same thickness as the separation between the plates is introduced so as to fill one-fourth of the capacitor as shown in the figure. the new capacitance will be
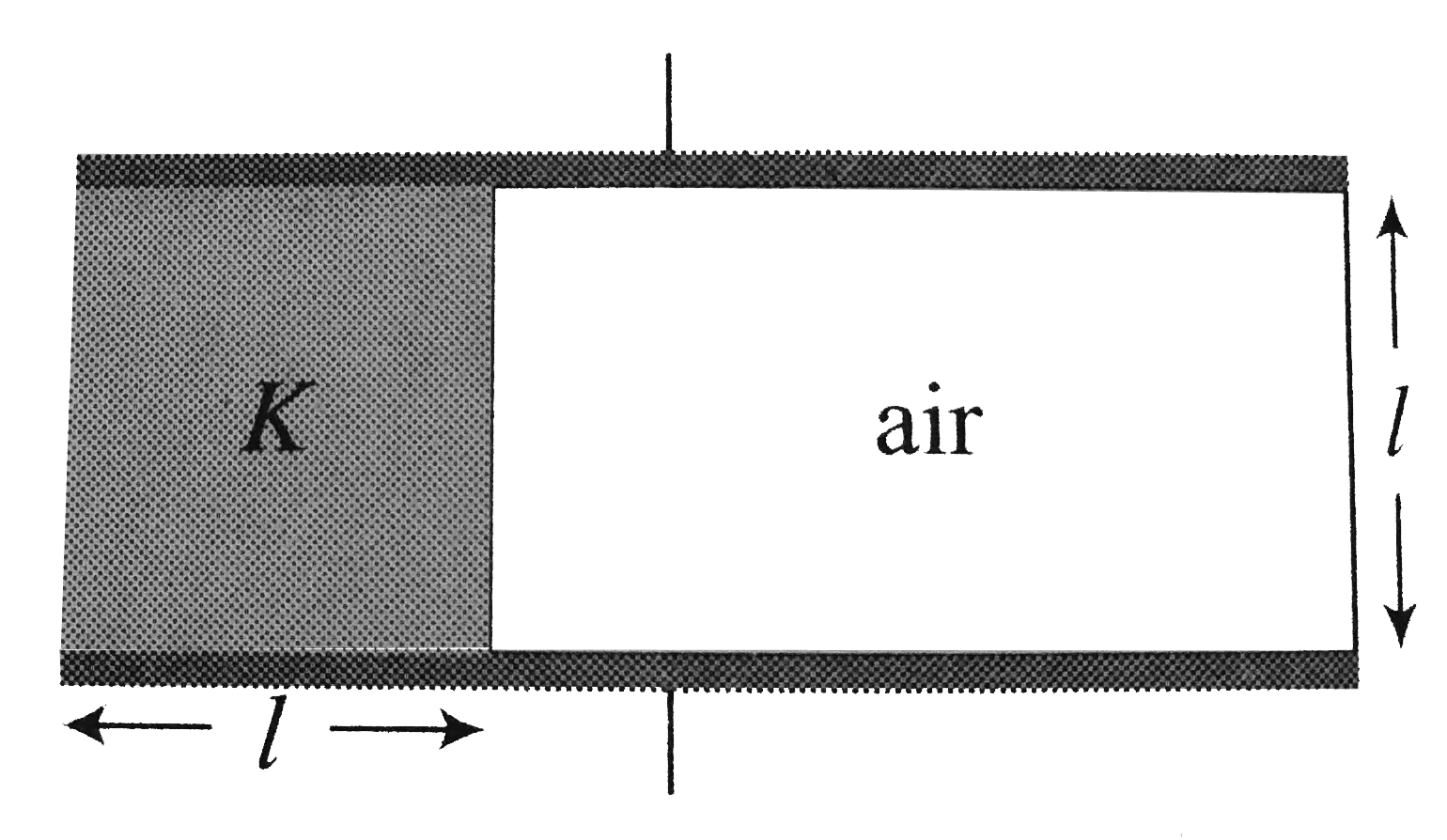 A(K+1)C4B(K+2)C4C(K+3)C4DKC4
A(K+1)C4B(K+2)C4C(K+3)C4DKC4Two material having the dielectric constans K1 and K2 are filled between two parallel plates of a capacitor.
where area of each plate is A and the distance between the plates is d.
The capacitor of the capacitor is:
 AAε0(K1×K2)d(K1+K2)BAε0(K1−K2)dCAε0(K1K2)(K1+K2)DAε0(K1+K2)d
AAε0(K1×K2)d(K1+K2)BAε0(K1−K2)dCAε0(K1K2)(K1+K2)DAε0(K1+K2)d
Find the capacitance between A and B if three dielectric slabs of dielectric constants K1areaA _(2)andthick≠ssd_(2))are∈sertedbetweentheplatesofaparal≤llate∩aci→rofplateareaA.(Giventˆthedistancebetweenthetwoplatesisd_(1)=d_(2)+d_(2).)
 .
.
View SolutionFind the capacitance between A and B if three dielectric slabs of dielectric constants K1areaA _(2)andthick≠ssd_(2))are∈sertedbetweentheplatesofaparal≤llate∩aci→rofplateareaA.(Giventˆthedistancebetweenthetwoplatesisd_(1)=d_(2)+d_(2).)
 .
.
View SolutionA parallel plate capacitor with air as the dielectric has capacitance C. A slab of dielectric constant K and having the same thickness as the separation between plates is introduced so as to fill one-fourth of the capacitor as shown in the figure. The new capacitance will be :
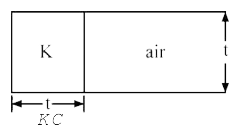
View SolutionA parallel plate capacitor with air as the dielectric has capacitance C. A slab of dielectric constant K and having the same thickness as the separation between plates is introduced so as to fill one-fourth of the capacitor as shown in the figure. The new capacitance will be :

View SolutionA dielectric slab is placed between the plates of a parallel plate capacitor. Its capacitance
View Solution
