Question

Obtain the expression of electric field at any point by continuous distribution of charge on a surface.

Explanation

Suppose, surface `DeltaS` is divided into small elements and `vecr` is the position vector on anyone element.

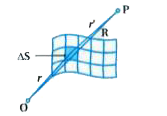
`sigma` is the surface charge density hence, charge on `DeltaS` surface element `DeltaQ = sigma.DeltaS, therefore sigma = (DeltaQ)/(DeltaS)`
Suppose a point P (inside or outside) the surface whose position vector is `vecR` and distance from `DeltaS` is r. and unit vector is r..
Electric field at P due to charge on a `sigma DeltaS`,
`DeltavecE = (ksigmaDeltaS)/(r.)^(2).hatr`
Total electric field at P by superposition principle,
`vecE = sum_(s) (ksigma.DeltaS)/(r.)^(2).hatr`
By integration method,
`vecE = int_(S)(ksigma.DeltaS)/(r.)^(2).hatr`