For a chemical reaction A→ Products, the rate of disappearance of A is given by:
dCAdt=K1CA1+K2CA at low CA, the reaction is of the _________order with rate constant_________(Assume K1,K2 are lesser than 1)Solution in Telugu
I,K1/K2
I,K1
II,K1/K2
II,K1/K1+K2
The correct Answer is:B
1st order Kt,xC0=(1−e−Kt),Ct=C0.e−Kt
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - AAKASH SERIESCHAPTER - CHEMICAL KINETICSEXERCISE - LEVEL-II(LECTURE SHEET EXERCISE-II LINKED COMPREHENSION TYPE QUESTIONS)
9 Videos
- CHEMICAL KINETICSBOOK - AAKASH SERIESCHAPTER - CHEMICAL KINETICSEXERCISE - LEVEL-II(LECTURE SHEET EXERCISE-III MATCH THE FOLLOWING QUESTIONS)
3 Videos
- CHEMICAL KINETICSBOOK - AAKASH SERIESCHAPTER - CHEMICAL KINETICSEXERCISE - LEVEL-1 (EXERCISE -II ORDER, MOLECULARITY, HALF LIFE:)
34 Videos
- CHEMICAL KINETCSBOOK - AAKASH SERIESCHAPTER - CHEMICAL KINETCSEXERCISE - EXERCISE - 3.2
45 Videos
- CHEMICAL THERMODYNAMICSBOOK - AAKASH SERIESCHAPTER - CHEMICAL THERMODYNAMICSEXERCISE - Additional Practice Exercise
54 Videos
Similar Questions
A certain endothermic reaction: A→ Product, ΔH=+ve proceeds ina sequence of three elementary steps with the rate constant K1,K2 and K3 and each one having energy of activation E1,E2 and E3 respectively at 25∘C. The observed rate constannt for the reaction is equal to K3√K1K2.
A1,A2 and A3 are Arrhenius parameters respectively.
The observed energy of activation for the reaction is
View SolutionMatch the following columns
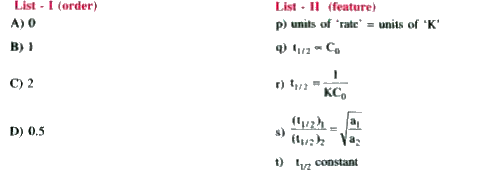
View SolutionSucrose decompose in acid solution into glucose and fructose according to the first order rate law, with t12=3.00 hours. What fraction of sample of sucrose remains after 8 hours ?
View SolutionDiscuss on the kinetic spontaneity of a chemical reaction. Is a slow reaction spontaneous? Why?
View SolutionWhich among the following is not an exact differential?
View SolutionTwo reaction : X→ Products and Y→ Products have rate constants k1 and k2 at temperature T and activation energies E1 and E2, respectively. If k1>k2 and E1<E2 (assuming that the Arrhenius factor is same for both the Products), then
(I) On increaisng the temperature, increase in k2 will be greater than increaisng in k1.
(II) On increaisng the temperature, increase in k1 will be greater than increase in k2.
(III) At higher temperature, k1 will be closer to k2.
(IV) At lower temperature, k1<k2
View SolutionFor a chemical reaction A rarr Products, the rate of disappearance of A is given by: -(dC_(A))/(dt)=(K_(1)C_(A))/(1+K_(2)C_(A)) At low C_(A) , the reaction is of the …. Order with rate constant …..: ("Assume" K_(1), K_(2) "are lesser than" 1)
View SolutionThe rate of reaction ((dx)/(dt)) varies with nature, physical state and concentration of reactants, temperature, exposure to light and catalyst, whereas rate constant (K) varies with temperature and catalyst only. The rate constant K is given as K=Ae^(-E_(a)//RT) where A is Arrhenius parameter or pre-exponential factor and E_(a) is energy of activation. The minimum energy required for a reaction is called threshold energy and the additional energy required by reactant molecules to attain threshold energy level is called energy of activation. For a chemical reaction: Ararr Product, the rate of disappearance of A is given by: (-dC_(A))/(dt)=K_(1) (C_(A))/(1+K_(2)C_(A)) . At low C_(A) , the order of reaction and rate constants are respectively:
View SolutionFor the consecutive unimolecular-type first-order reaction Ak1−−→Rk2−−→S, the concentration of component R, CR at any time t is given by -
CR=COAK1[e−k1t(k2−k1)+e−k2t(k1−k2)]
if CA=CAO,CR=CRO=0 at t=0
The time at which the maximum concentration of R occurs is -
View SolutionFor an elementary chemical reaction, A_(2) underset(k_(-1))overset(k_(1))(hArr) 2A , the expression for (d[A])/(dt) is
View SolutionConsider following two reactions
A→Product,d[A]dt=k1[A]00
B→Product,d[B]dt=k1[A]00
kaandk2 are expressed are expressed in term of molarity (mol L−1) and time (s−1) as :
View SolutionFor a reaction of reversible nature , net rate is (dxdt)=k1[A]2[B]1−k2[C], hence , given reactionis:
View SolutionFor a reaction of reversible nature, net rate is ((dx)/(dt))=k_(1)[A][B]-k_(2)[C] hence, given reaction is :
View SolutionIn the sequence of reaction
AK1−−→BK2−−→CKc−−→D,K3>K2>K1 then the rate determining step of the reaction is
View SolutionA chemical reaction was carried out at 300 K and 280 K. The rate constants were found to be k1 and k2 respectively . Then
View Solution
