Six point masses of mass m each are at the vertices of a regular hexagon of side l. Calculate the force on any of the masses.
To calculate the force on any of the masses located at the vertices of a regular hexagon with side length l, we will follow these steps:
Step 1: Identify the Masses and Their Arrangement
We have six point masses, each of mass m, positioned at the vertices of a regular hexagon. We will calculate the net gravitational force acting on one of these masses due to the other five masses.
Step 2: Calculate the Force Due to Adjacent Masses
The force between two point masses is given by Newton's law of gravitation:
F=Gm2r2
For the two adjacent masses, the distance r is equal to the side length l. Therefore, the force F1 exerted on our chosen mass by each adjacent mass is:
F1=Gm2l2
Step 3: Calculate the Force Due to Opposite Mass
Next, we consider the force exerted by the mass directly opposite to our chosen mass. The distance between these two masses is the length of the diagonal of the hexagon, which can be calculated as:
r=2l
Thus, the force F2 exerted by the opposite mass is:
F2=Gm2(2l)2=Gm24l2
Step 4: Resolve Forces into Components
The forces from the adjacent masses F1 act at an angle of 60∘ to the line connecting the center of the hexagon to the chosen mass. We can resolve these forces into their horizontal and vertical components.
The horizontal components of the forces from the two adjacent masses are:
F1x=F1cos(60∘)=Gm2l2⋅12=Gm22l2
Since there are two adjacent masses, the total horizontal component from both is:
F1x,total=2⋅F1x=2⋅Gm22l2=Gm2l2
The vertical components of the forces from the two adjacent masses are:
F1y=F1sin(60∘)=Gm2l2⋅√32=Gm2√32l2
Since both vertical components point in the same direction, the total vertical component from both is:
F1y,total=2⋅F1y=2⋅Gm2√32l2=Gm2√3l2
Step 5: Add the Force from the Opposite Mass
The force F2 from the mass directly opposite is purely horizontal and acts in the opposite direction:
F2x=−F2=−Gm24l2
The vertical component from the opposite mass is zero:
F2y=0
Step 6: Calculate the Net Force Components
Now we can sum the components to find the net force:
- Horizontal component:
Fnet,x=F1x,total+F2x=Gm2l2−Gm24l2=Gm2l2(1−14)=Gm2l2⋅34=3Gm24l2
- Vertical component:
Fnet,y=F1y,total=Gm2√3l2
Step 7: Calculate the Magnitude of the Net Force
The magnitude of the net force Fnet can be calculated using the Pythagorean theorem:
Fnet=√F2net,x+F2net,y
Substituting the values:
Fnet=
⎷(3Gm24l2)2+(Gm2√3l2)2
=√9G2m416l4+3G2m4l4=√9G2m4+48G2m416l4=√57G2m416l4=Gm2√574l2
Final Answer
The net gravitational force on any of the masses at the vertices of the hexagon is:
Fnet=Gm2√574l2
We have six point masses, each of mass m, positioned at the vertices of a regular hexagon. We will calculate the net gravitational force acting on one of these masses due to the other five masses.
The force between two point masses is given by Newton's law of gravitation:
F=Gm2r2
For the two adjacent masses, the distance r is equal to the side length l. Therefore, the force F1 exerted on our chosen mass by each adjacent mass is:
F1=Gm2l2
Next, we consider the force exerted by the mass directly opposite to our chosen mass. The distance between these two masses is the length of the diagonal of the hexagon, which can be calculated as:
r=2l
Thus, the force F2 exerted by the opposite mass is:
F2=Gm2(2l)2=Gm24l2
The forces from the adjacent masses F1 act at an angle of 60∘ to the line connecting the center of the hexagon to the chosen mass. We can resolve these forces into their horizontal and vertical components.
F1x=F1cos(60∘)=Gm2l2⋅12=Gm22l2
Since there are two adjacent masses, the total horizontal component from both is:
F1x,total=2⋅F1x=2⋅Gm22l2=Gm2l2
F1y=F1sin(60∘)=Gm2l2⋅√32=Gm2√32l2
Since both vertical components point in the same direction, the total vertical component from both is:
F1y,total=2⋅F1y=2⋅Gm2√32l2=Gm2√3l2
The force F2 from the mass directly opposite is purely horizontal and acts in the opposite direction:
F2x=−F2=−Gm24l2
The vertical component from the opposite mass is zero:
F2y=0
Now we can sum the components to find the net force:
- Horizontal component:
Fnet,x=F1x,total+F2x=Gm2l2−Gm24l2=Gm2l2(1−14)=Gm2l2⋅34=3Gm24l2
- Vertical component:
Fnet,y=F1y,total=Gm2√3l2
The magnitude of the net force Fnet can be calculated using the Pythagorean theorem:
Fnet=√F2net,x+F2net,y
Substituting the values:
Fnet=
⎷(3Gm24l2)2+(Gm2√3l2)2
=√9G2m416l4+3G2m4l4=√9G2m4+48G2m416l4=√57G2m416l4=Gm2√574l2
The net gravitational force on any of the masses at the vertices of the hexagon is:
Fnet=Gm2√574l2
Topper's Solved these Questions
Similar Questions
Three masses of 1 kg each are kept at the vertices of an equilateral triangle of side 3 m. What is the force acting on a point mass of 5 kg placed at its centroid ?
View SolutionFive point charge (+q each) are placed at the five vertices of a regular hexagon of side 2a.what is the magnitude of the net electric field at the centre of the hexazon?
View Solution
Knowledge Check
Six point charges are arrange at the vertices of a regular hexagon of side length a (shown in figure).
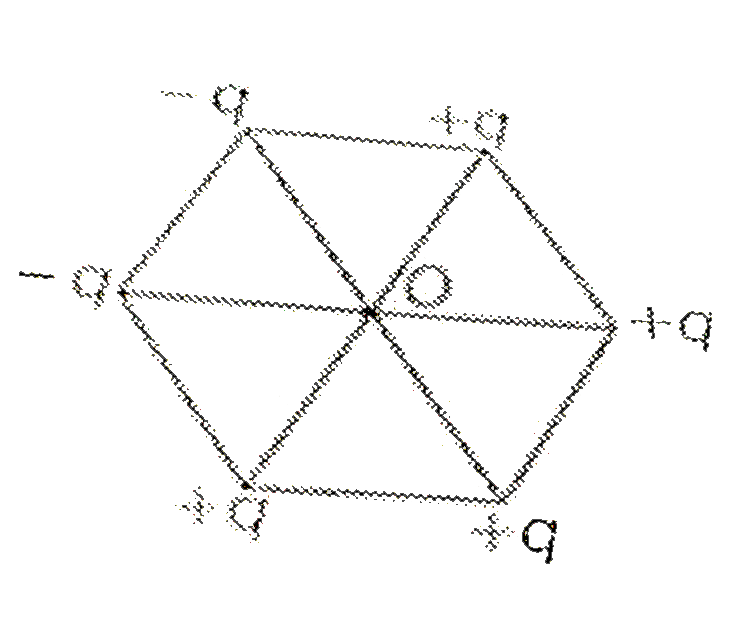
Find the magnitude of electric field at the centre of regular hexagon.A−qB−q6C+1.83qD−1.83q
Five point masses m each are kept at five vertices of a regular pentagon. Distance of center of pentagon from any one of the vertice is 'a'. Find gravitational potential and filed strength at center.
View SolutionThree equal masses of 1 kg each are placed at the vertices of an equilateral triangle of side 1 m, then the gravitational force on one of the masses due to other masses is (approx.)
View SolutionFour point masses easch of mass 'm' are placed at four vertieces A, B, C, nd D of a regular hexagon of side 'a' as shown in figure , Find gravitational potential and field strength at the centre O of the hexagon.
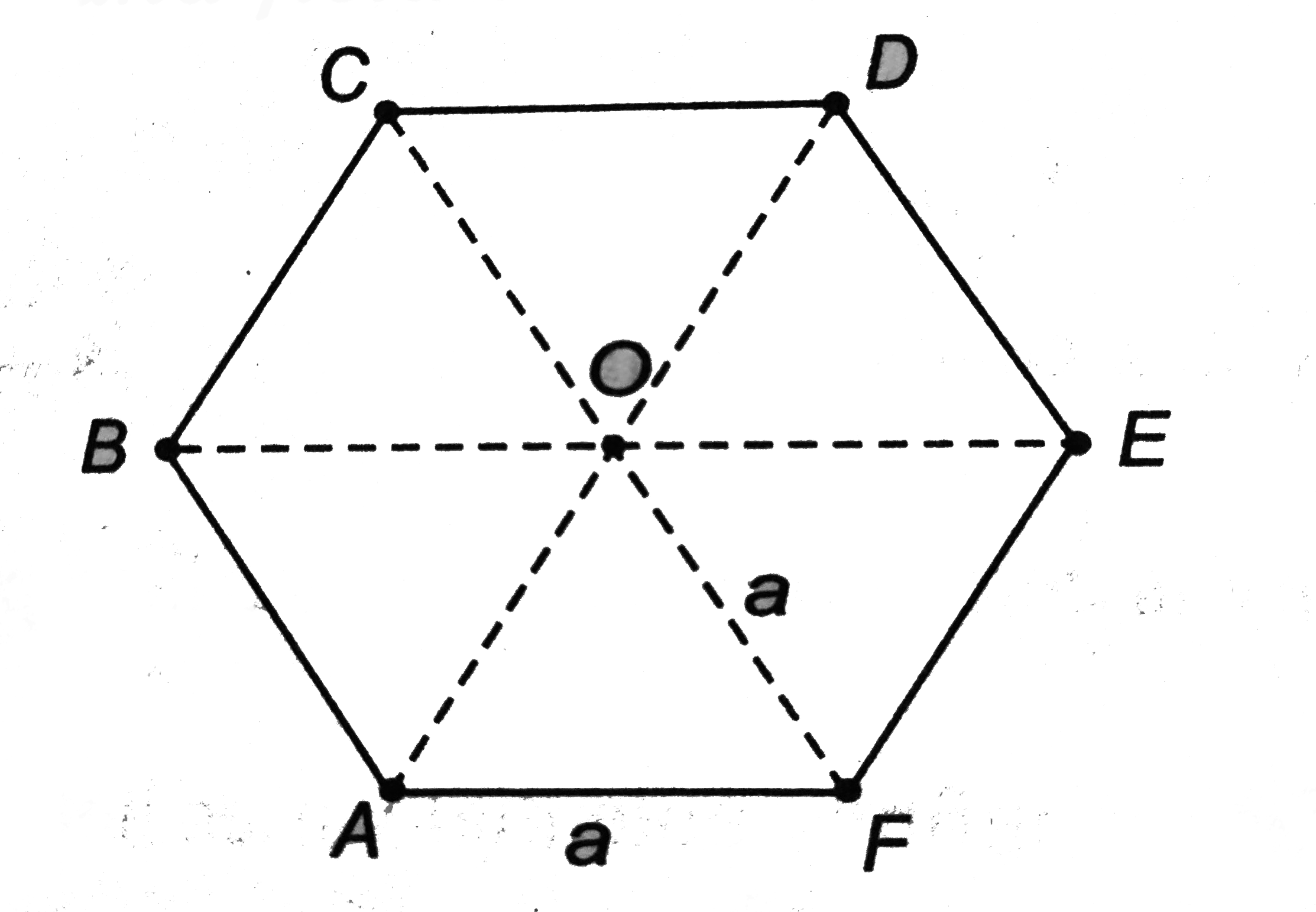 .
.
View SolutionThree identical point objects each of mass m are placed at the vertices of an equilateral triange of side l. What is the gravitational potential at the centre of the equilateral triangle due to the point masses?
View SolutionSix point charges are kept at the vertices of a regular hexagon of side L and centre O, as shown in the figure. Given that K=14πε0qL2, which of the following statements(s) is (are) correct?
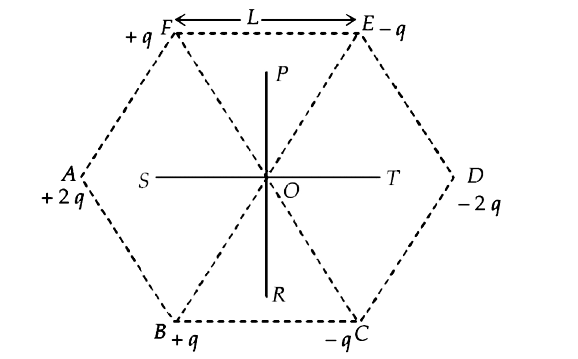
View SolutionSix point charges are placed at the vertices of a regular hexagon of side a as shown. If E represents electric field and V represents electric potential at O then,
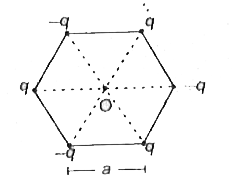
View SolutionThree equal masses of 1.5 kg each are fixed at the vertices of an equilateral triangle of side 1 m. What is the force acting on a particle of mass 1 kg placed at its centroid ?
View Solution
