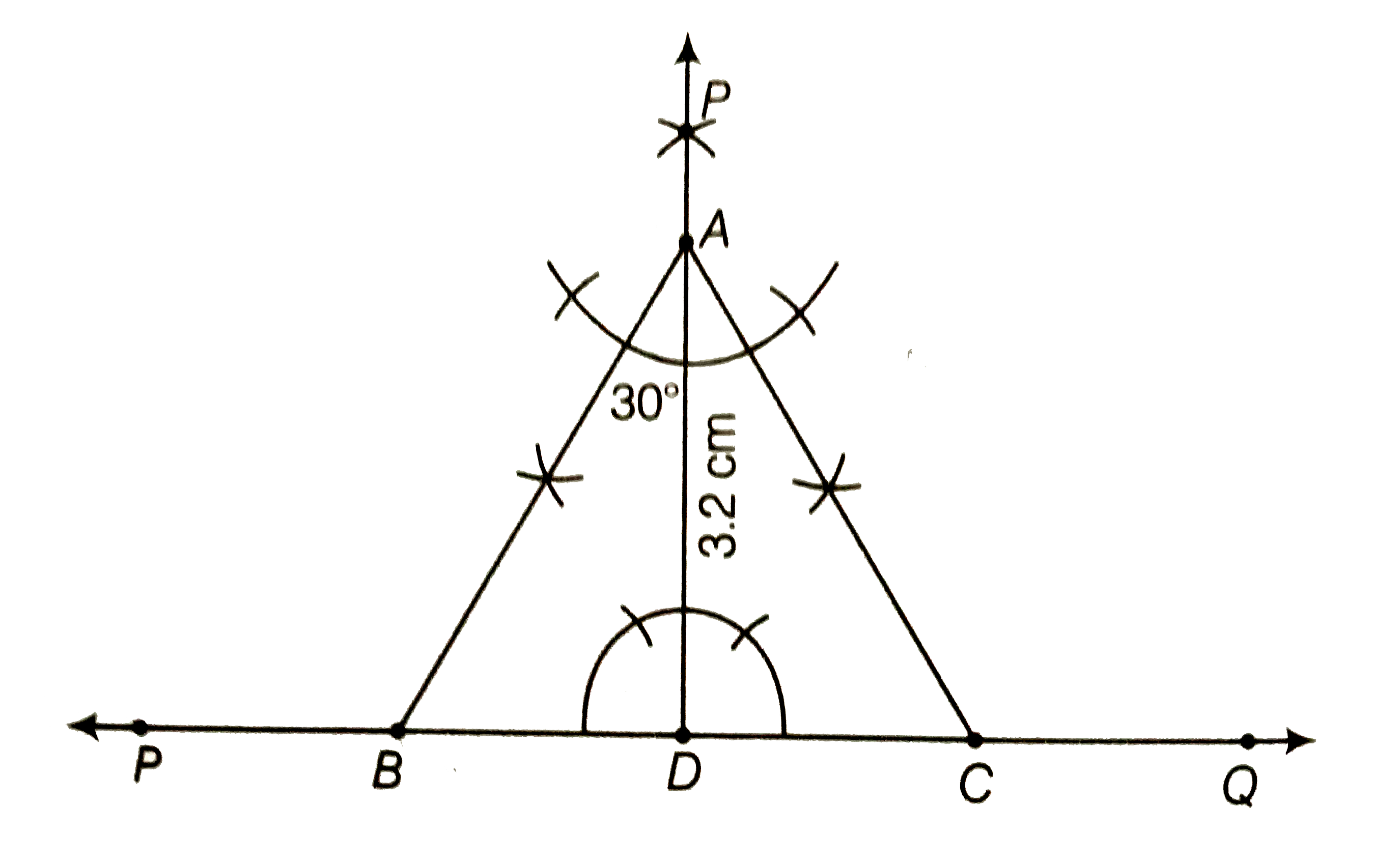
An equilateral triangle, if its altitude is $3.2 c m$

Text Solution

Verified by Experts

we know that , in an equilateral triangle all sides are equal and all angles are equal i.e., each angle is of $60^{\circ}$ .
Given , altitudeof an equilateral triangle say ABC is $3.2 c m$ . To construct the $Δ A B C$ use the following steps.
(i) Draw a line PQ.
(ii) Take a point D on PQ and draw a ray $D E ⊥ P Q$ .
(iii) Cut the line segment AD of length $3.2 c m$ from DE.
(iv) Make angles equal to $30^{\circ}$ at A on both sides of AD say $∠ C A D and ∠ B A D$ , where B and C lie on PQ.
(v) Cut the line segment DC from PQ such that DC = AD
(vi) Join AC
Thus , $Δ A B C$ is the required triangle.
Justification
Here, $∠ A = ∠ B A D + ∠ C A D$
= $30^{\circ} + 30^{\circ} = 60^{\circ}$
Also, $A D ⊥ B C$
$∴ ∠ A D B = 90^{\circ}$
In $Δ A B D ∠ B A D + ∠ D B A = 180^{\circ}$ [angle sum property]
$30^{\circ} + 90^{\circ} + ∠ D B A = 180^{\circ}$ [ $∠ B A D = 30^{\circ}$ , by construction]
$∠ D B A = 60^{\circ}$
Similary, $∠ D C A = 60^{\circ}$
Thus , $∠ A = ∠ B = ∠ C = 60^{\circ}$
Hence, $Δ A B C$ is an equilateral triangle.

Updated on:21/07/2023