In ΔABCandΔDEF, ∠B=∠E,∠F=∠C and AB=3DE. Then, the two triangles are
congruent but not similar
similar but not congruent
neither congruent nor similar
congruent as well as similar
The correct Answer is:B
To solve the problem, we need to analyze the given information about triangles ΔABC and ΔDEF.
1. Identify Given Information:
- ∠B=∠E
- ∠F=∠C
- AB=3×DE
2. Use Angle-Angle (AA) Similarity Criterion:
- Since two angles of triangle ΔABC are equal to two angles of triangle ΔDEF, we can conclude that the triangles are similar by the AA similarity criterion.
- Therefore, we can write:
ΔABC∼ΔDEF
3. Determine the Relationship of Sides:
- From the similarity of triangles, we know that the ratios of the corresponding sides are equal. Thus, we can express this as:
ABDE=BCEF=ACDF
- Given AB=3×DE, we can substitute this into our ratio:
3×DEDE=3
- This implies that the side AB is three times the side DE.
4. Conclusion about Congruence:
- For two triangles to be congruent, all corresponding sides must be equal. Here, we have established that AB is not equal to DE (since AB=3×DE).
- Therefore, while the triangles are similar, they are not congruent.
5. Final Statement:
- We conclude that ΔABC is similar to ΔDEF but not congruent.
Final Answer:
The two triangles are similar but not congruent.
---
- ∠B=∠E
- ∠F=∠C
- AB=3×DE
- Since two angles of triangle ΔABC are equal to two angles of triangle ΔDEF, we can conclude that the triangles are similar by the AA similarity criterion.
- Therefore, we can write:
ΔABC∼ΔDEF
- From the similarity of triangles, we know that the ratios of the corresponding sides are equal. Thus, we can express this as:
ABDE=BCEF=ACDF
- Given AB=3×DE, we can substitute this into our ratio:
3×DEDE=3
- This implies that the side AB is three times the side DE.
- For two triangles to be congruent, all corresponding sides must be equal. Here, we have established that AB is not equal to DE (since AB=3×DE).
- Therefore, while the triangles are similar, they are not congruent.
- We conclude that ΔABC is similar to ΔDEF but not congruent.
The two triangles are similar but not congruent.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - Very Short Answer Typw Questions12videos
TRIANGLES
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - Short Answer Type Questions15videos
TRIANGLES
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - LONG ANSWER TYPE QUESTIONS20videos
SURFACE AREAS AND VOLUMES
Similar Questions
In ΔABCandΔPQR , If AB=AC, ∠C=∠P and ∠B=∠Q, then the two triangles are
View SolutionIn ΔABCandΔPQR,∠A=∠Qand∠B=∠R. Which side of ΔPQR should be equal to side AB of ΔABC, so that the two triangle are congruent ? Give reason for your answer.
View SolutionIn a ΔABC,∠A=2∠B=3∠C, find each of the triangle.
View SolutionWhich of the following pairs of triangles are congruent ? Also state the condition of congruency in each case :
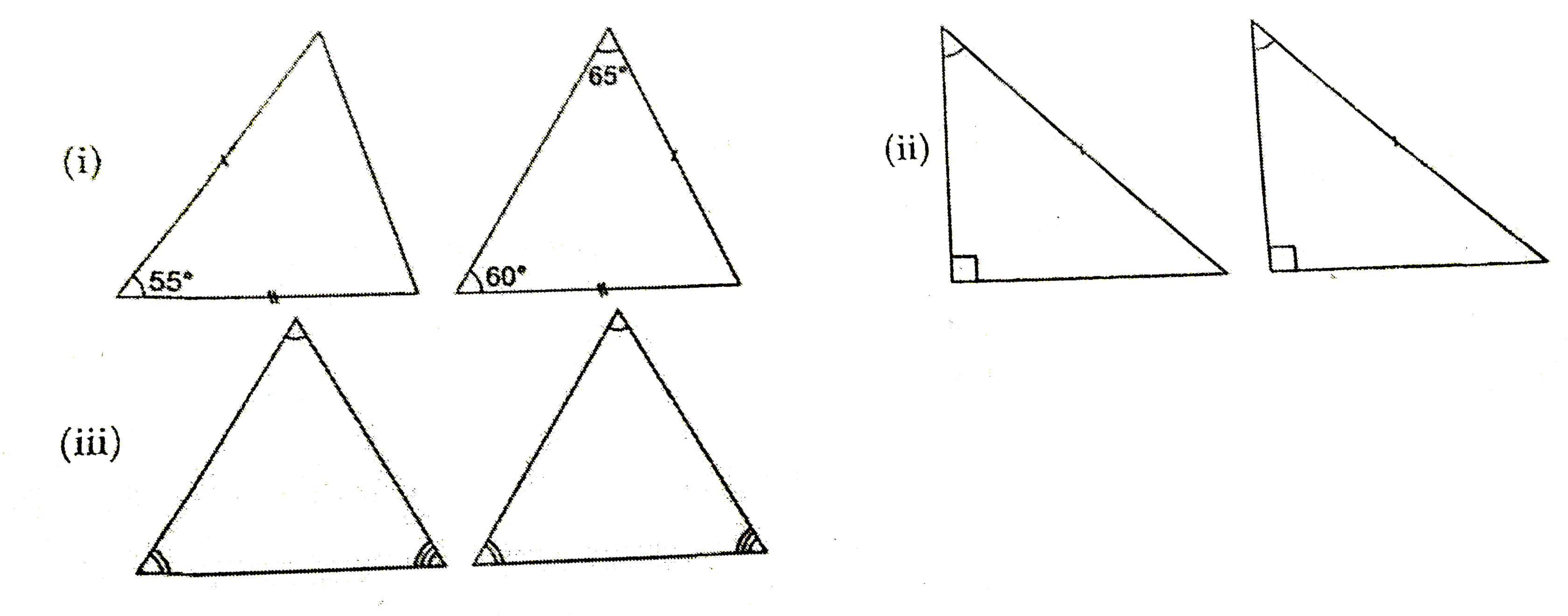
(iv) In ΔABC and ΔDEF,AB=EF,BC=DF and ∠B=∠F
(v) In ΔABC and ΔPQR,AB=QR,AC=PR and ∠B=∠R
(vi) In ΔABC and ΔPQR,∠A=∠P,AC=PR and AB=PQ
(vi) In ΔABC and ΔPQR,AB=QR,∠A=∠Q and AC=QP.View SolutionΔABC , ∠A+∠B=65∘, ∠B+∠C=140∘,then ∠B is equal to
View SolutionIn ΔABC,∠A=x+15∘,∠B=xand∠C=2x−35∘ find, each angle of the triangle.
View SolutionA triangle ABC is such that 3∠A=4∠B=6∠C. Find the three angles.
View SolutionIn ΔABC,∠A−∠B=16∘and∠C−∠A=34∘=34∘ , find all angles of the triangle
View SolutionIn ΔABC,AB=AC and the bisectors of ∠B and ∠C meet AC and AB at point D and E respectively. Prove that BD=CE.
View SolutionIn ΔABC,∠A=2πc3 and ∠B=45∘. Find ∠C in both the systems.
View Solution

