Two fixed, ideantical conducting plates , each of surface area S are charged to -Q and q, respectively, where Qgtqgt0. A third identical plate , free to move is located on the other side of the plate with charge q at a distance d (figure). the third plate is released and collides with the plate . Assume the collision is elastic and the time of collision is sufficient to redistribute charge amongst .
(a). Find the electric field acting on the plate before collision. ltBrgt (b) Find the cahrges on after the collision.
(c) Fid the velocity of the plate after the collision and at a distance d from the plate
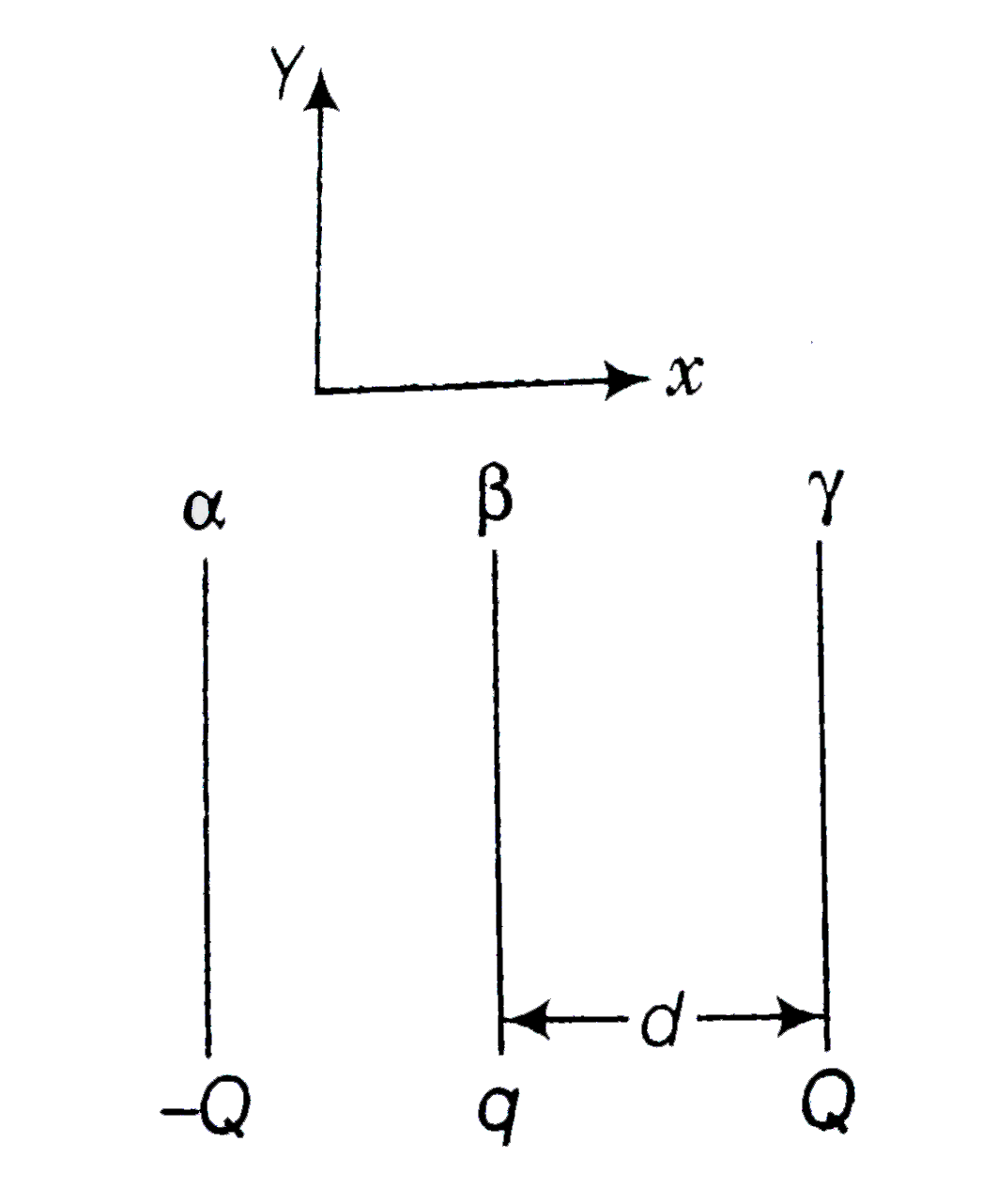
(a). Find electric field at plate before collision is equal to the sum of electric field at plate due to plate .
The electric field at plate due to plate is to the left.
The electric field at plate due to plate is , to the right
Hence, the ent electric field at plate before collision,
, to the left if
(b) During collision, plates are together. Their potentials become same.
Suppose charge on plate is and cahrge on plate is . At any point O, in between the two plates, the electric field must be zero.
Electric field at O due to plate , to the left
Electric field at O due to plate , to the right ltBrgt Electric field at O due to plate , to the left
As the electric field at O is zero, therefore ltBrgt
. . .(i)
As there is no loss of charge o collision, ltBrgt
On solving eqs. (i) and (ii), we get
=charge on plate
=charge on plate
(c) After collision, at a distance d from plate .
Let the velocity of plate be v. After the collision, electric field at plate is
to the right
just before collision, electric field at plate is
If is force on plate before collision, then
Total work done by the electric field is round trip movement of plate
ltBrgt If m is mass of plate , the KE gained by plate
According to work-energy principle,
.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDEXERCISE - Short Answer Type question
6 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - LONG ANSWER TYPE QUESTION
5 Videos
- ELECTROMAGNETIC INDUCTION BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - Long
11 Videos
Similar Questions
Two fixed, identical conducting plates (αandβ), each of surface area S are charged to -Q and q, respectively, where Q > q > 0. A third identical plate (γ), free to move is located on the other side of the plate with charge Q at a distance d (figure). The third plate is released and collides with the plate β. Assume the collision is elastic and the time of collision is sufficient to redistribute charge amongst βandγ.
(a) Find the electric field acting on the plate γ before collision.
(b) Find the charges on βandγ after the collision.
(c) Find the velocity of the plate γ after the collision and at a distance d from the plate β.
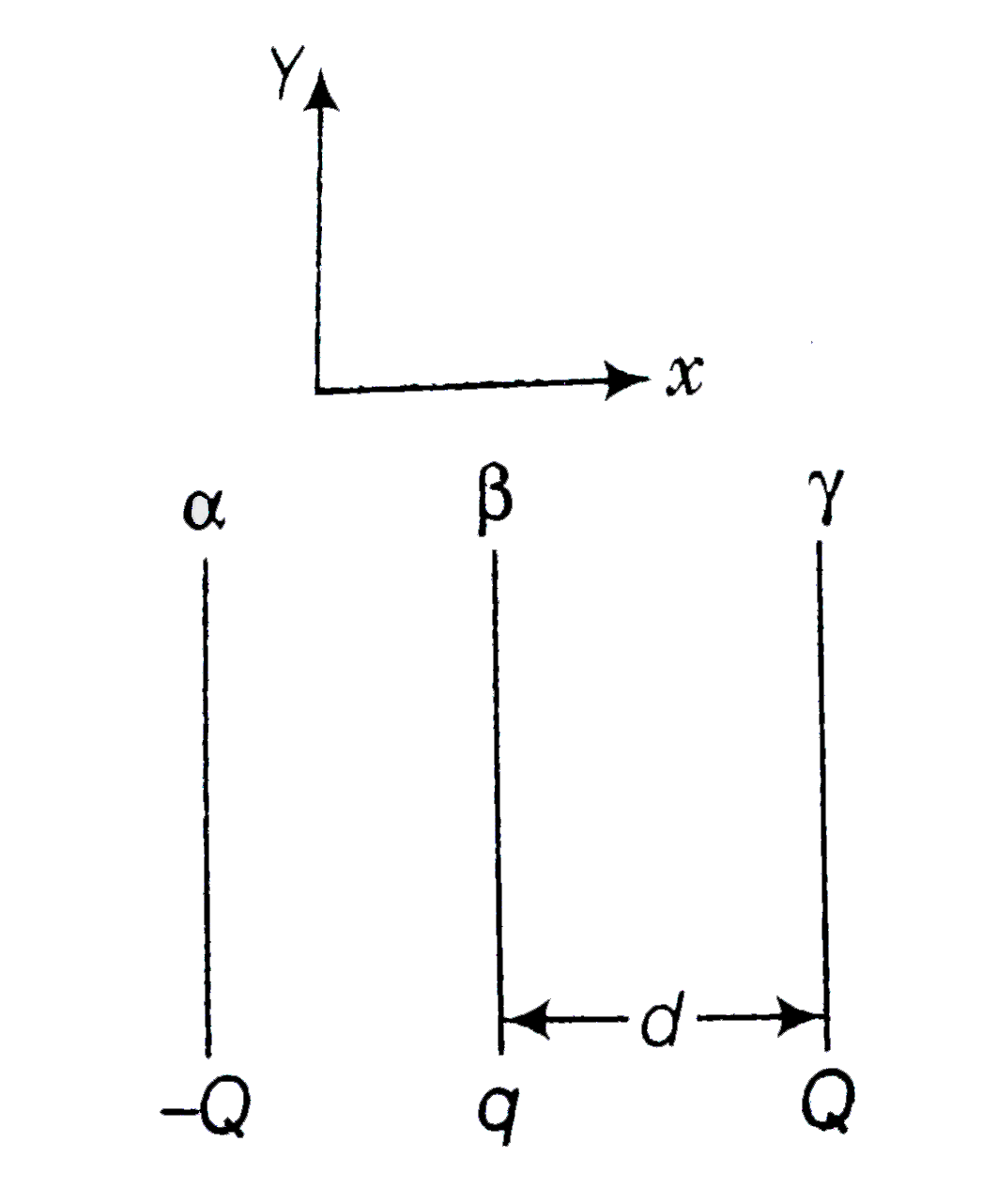
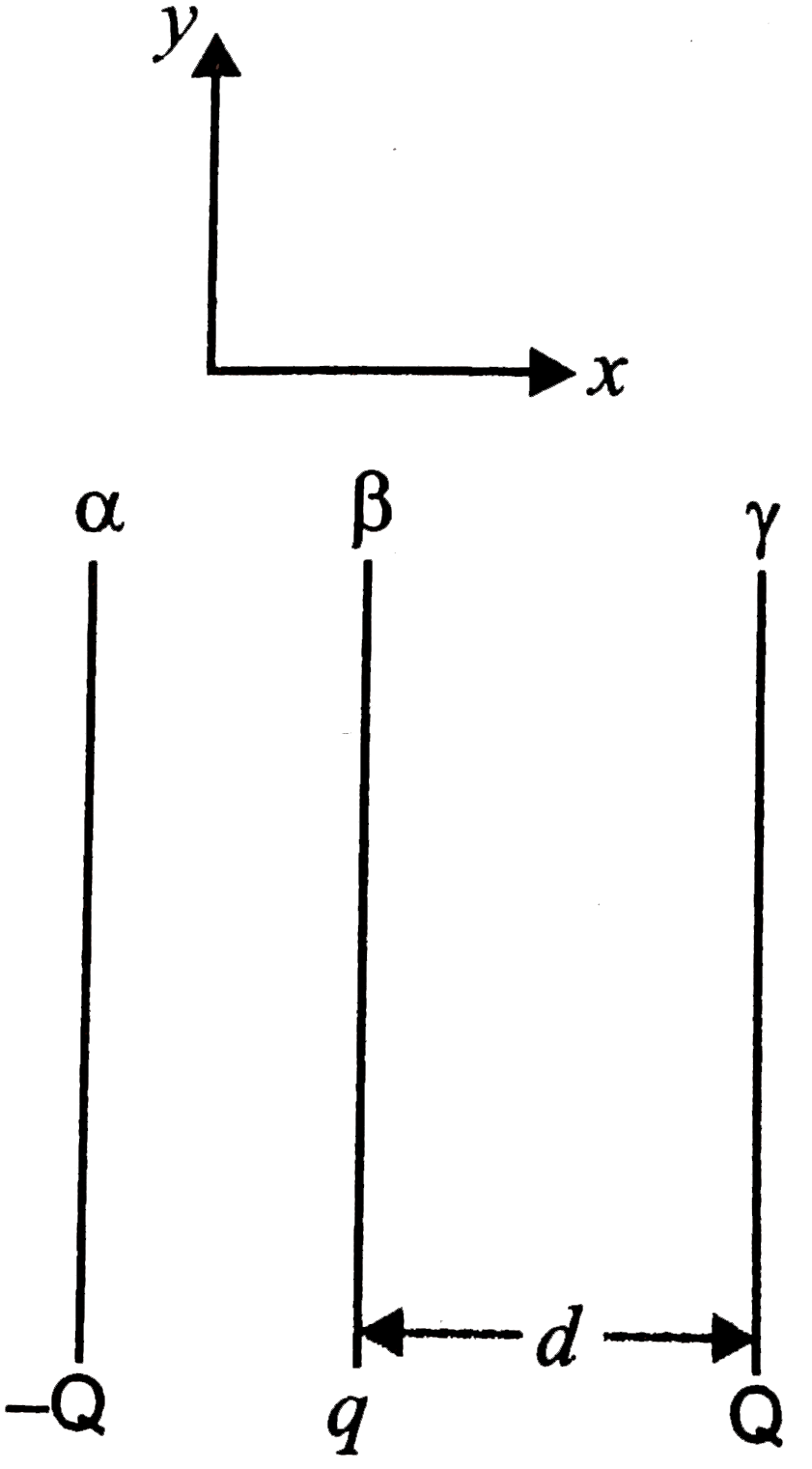
View SolutionTwo fixed, identical conducting plates (αandβ), each of surface area S are charged to −Qandq, respectively, where Q>q>0. A third indentical plate (γ), free to move is located on the other side of the plate with charge q at a distance d, fig. The third plate is released and collidies with the plate β. Assume the collsion is electric and the time of collision is sufficient to redistribute charge amongst βandγ.
(a) Find the electric field acting on the plate γ before collision.
(b) Find the charge on βandγ after the collision.
(c) Find the velocity of the plate γ after the collision and at a distance d from the plate β.
View Solution
Knowledge Check
Two parallel conducting plates, each of area A, are separated by a distance d. Now, the left plate is given a positive charge Q. A positive charge q of mass m is released from a point near the left plate. Find the time taken by the charge to reach the right plate.
 A(√3dmε0AqQ)B(√4dmε0AqQ)C(√2dmε0AqQ)Dnone of these
A(√3dmε0AqQ)B(√4dmε0AqQ)C(√2dmε0AqQ)Dnone of these
Two identical metal plates each having surface area A, having charges q1andq2, are placed facing each other at a separation d. Find the charge appearing on
surface (1),(2),(3), and (4). Assume the size of the plate is much larger than the separation between the plates.
View SolutionTwo identical metal plates each having surface area A, having charges q1andq2, are placed facing each other at a separation d. Find the charge appearing on
surface (1),(2),(3), and (4). Assume the size of the plate is much larger than the separation between the plates.
View SolutionTwo large conducting plates of a parallel plate capacitor are given charges Q and 3Q respectively. If the electric field in the region between the plates is E0 , then the force of interaction between the plates is

View SolutionTwo large conducting plates of a parallel plate capacitor are given charges Q and 3Q respectively. If the electric field in the region between the plates is E0 , then the force of interaction between the plates is

View SolutionForce between two conducting large plates , with charges Q and Q2 as shown in the figure, is (Area of each face of plate =A)
_PHY_E01_022_Q01.png)
View SolutionForce between two conducting large plates , with charges Q and Q2 as shown in the figure, is (Area of each face of plate =A)
_PHY_E01_022_Q01.png)
View SolutionTwo parallel conducting plates, each of area A, are separated by a distance d. Now, the left plate is given a positive charge Q. A positive charge q of mass m is released from a point near the left plate. Find the time taken by the charge to reach the right plate.

View Solution
