Inductance : Self and Mutual
Step-by-Step Solution:
1. Understanding Inductance:
- Inductance is a property of a coil (or inductor) that quantifies its ability to store energy in a magnetic field when an electric current passes through it. It is defined as the ratio of the induced electromotive force (EMF) in the coil to the rate of change of current through it.
2. Self-Inductance:
- Self-inductance occurs in a single coil. When the current flowing through the coil changes, it alters the magnetic field around it. This change in magnetic field induces an EMF in the same coil, opposing the change in current according to Lenz's Law. The formula for self-inductance (L) is given by:
L=−dΦdt
where Φ is the magnetic flux linked with the coil.
3. Mutual Inductance:
- Mutual inductance occurs between two coils. When the current in the first coil changes, it creates a changing magnetic field that can induce an EMF in the second coil. The mutual inductance (M) between two coils is defined as:
M=−dΦ21dt
where Φ21 is the magnetic flux through coil 2 due to the current in coil 1.
4. Key Differences:
- The key difference between self and mutual inductance is that self-inductance involves only one coil and the induced EMF is due to its own changing current, while mutual inductance involves two coils and the induced EMF in one coil is due to the changing current in the other coil.
5. Applications:
- Inductance plays a crucial role in various electrical applications such as transformers (which utilize mutual inductance), inductors in circuits, and energy storage systems.
- Inductance is a property of a coil (or inductor) that quantifies its ability to store energy in a magnetic field when an electric current passes through it. It is defined as the ratio of the induced electromotive force (EMF) in the coil to the rate of change of current through it.
- Self-inductance occurs in a single coil. When the current flowing through the coil changes, it alters the magnetic field around it. This change in magnetic field induces an EMF in the same coil, opposing the change in current according to Lenz's Law. The formula for self-inductance (L) is given by:
L=−dΦdt
where Φ is the magnetic flux linked with the coil.
- Mutual inductance occurs between two coils. When the current in the first coil changes, it creates a changing magnetic field that can induce an EMF in the second coil. The mutual inductance (M) between two coils is defined as:
M=−dΦ21dt
where Φ21 is the magnetic flux through coil 2 due to the current in coil 1.
- The key difference between self and mutual inductance is that self-inductance involves only one coil and the induced EMF is due to its own changing current, while mutual inductance involves two coils and the induced EMF in one coil is due to the changing current in the other coil.
- Inductance plays a crucial role in various electrical applications such as transformers (which utilize mutual inductance), inductors in circuits, and energy storage systems.
Topper's Solved these Questions
Similar Questions
(A): The working of dynamo is based on the principle of self induction.
(R) : Self induction of a coil is numerically equal to the magnetic flux linked with the coil, when a unit current flow through it.
View SolutionIn Fig, the mutual inductance of a coil and a very long straight wire is M, coil has resistance R and self-inductance L. The current in the wire varies according to the law I=at, where a is a constant and t is the time, the time dependence of current in the coil is
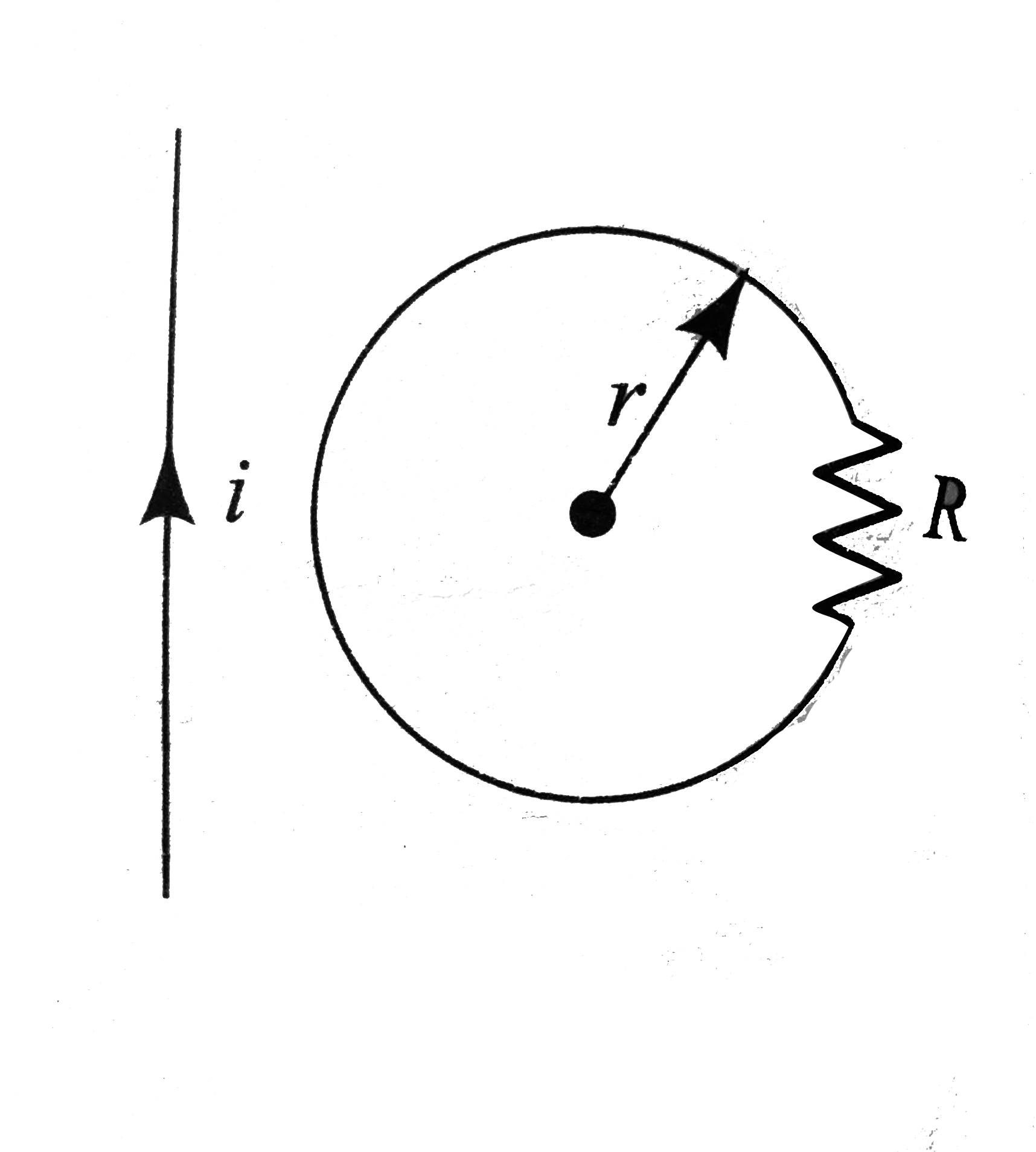
View Solution
Knowledge Check
Two inductors of inductance L each are connected in series with opposite magnetic fluxes. The resultant inductance is
(Ignore mutual inductance)AzeroBLC2LD3L
Two different coils of self inductance L 1 and L 2 are placed close to each other so that the effective flux in one coil is completely linked with other. If M is the mutual inductance between them, then

View SolutionSelf inductance of a soleniod can be increased by
View SolutionA coil of wire of a certain radius has 100 turns and a self inductance of 15 mH. The self inductance of a second similar coil of 500 turns will be
View SolutionA coil of wire of a certain radius has 600 turns and a self-inductance of 108mH. The self-inductance of a 2nd similar coil of 500 turns will be
View SolutionA coil of wire of a certain radius has 600 turns and a self-inductance of 108 mH. The self-inductance of a 2nd similar coil of 500 turns will be
View SolutionInductance of a solenoid is 3H and it consist of 500 truns . If number of turn make twice , then the value of self inductance becomes :
View SolutionFind the mutual inductance of a straight long wire and a rectangular loop, as shown in the figure.
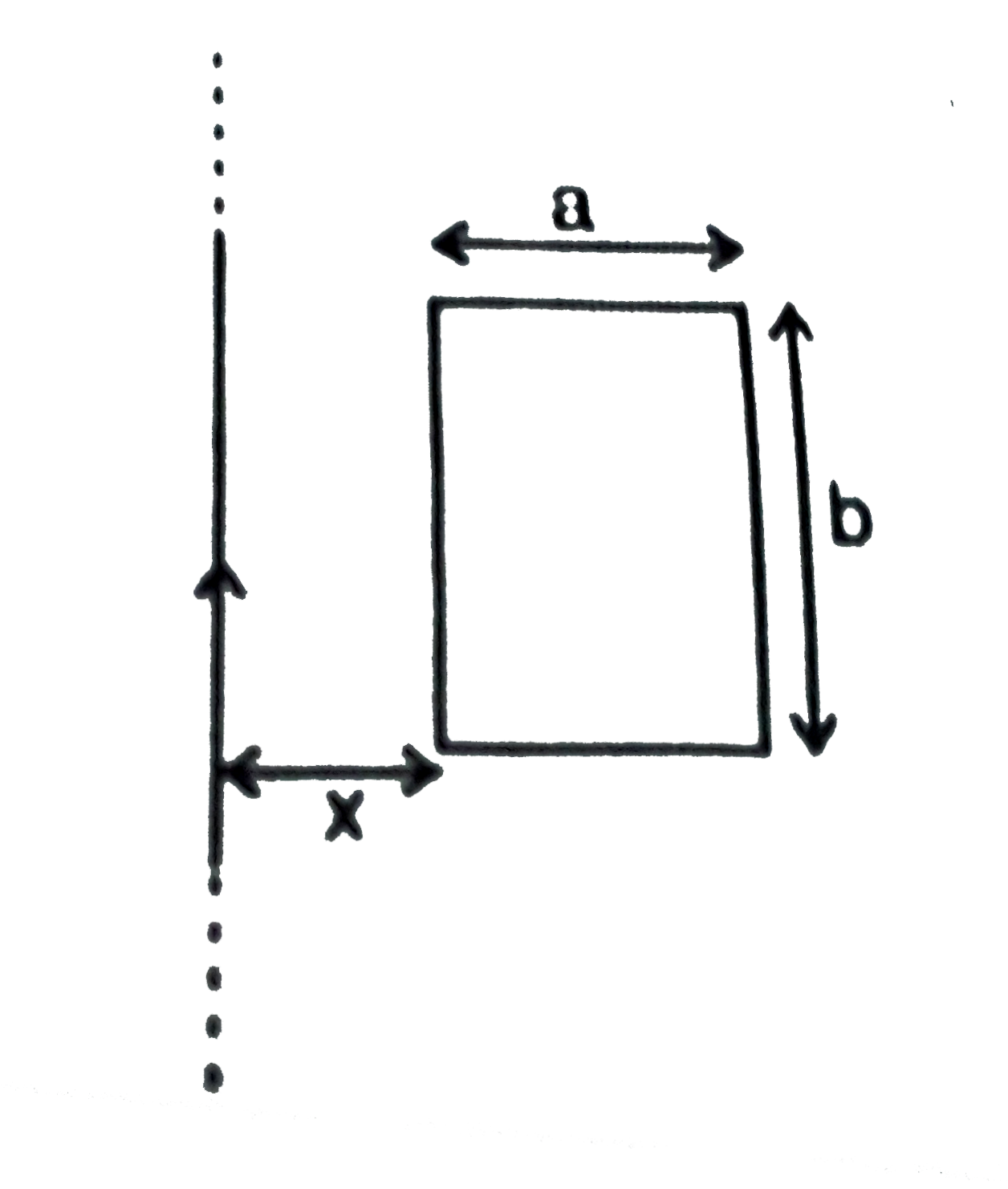
View Solution
