Column I, Column II: possible argument of z=a+ib ab>0 , p. tan−1∣∣∣ba∣∣∣ ab<0 , q. πtan−1∣∣∣ba∣∣∣ a2+b2=0 , r. tan−1ba ab=0 , s. π+tan−1ba , t. not defined , u. 0orπ2
To solve the problem, we need to determine the possible arguments of the complex number z=a+ib based on the conditions provided in the columns. Let's analyze each case step by step.
Step 1: Analyze ab>0
- If ab>0, it means both a and b are either positive or both are negative.
- If both a and b are positive, the complex number lies in the first quadrant, and the argument is given by:
Argument=tan−1(ba)
- If both a and b are negative, the complex number lies in the third quadrant. The argument in this case can be calculated as:
Argument=tan−1(ba)−π
Thus, for ab>0, the possible argument is:
- p:tan−1∣∣∣ba∣∣∣ (for a>0,b>0)
- q:π+tan−1∣∣∣ba∣∣∣ (for a<0,b<0)
Step 2: Analyze ab<0
- If ab<0, it means one of a or b is positive and the other is negative.
- If a<0 and b>0, the complex number lies in the second quadrant, and the argument is:
Argument=π−tan−1∣∣∣ba∣∣∣
- If a>0 and b<0, the complex number lies in the fourth quadrant, and the argument is:
Argument=−tan−1∣∣∣ba∣∣∣
Thus, for ab<0, the possible argument is:
- q:π−tan−1∣∣∣ba∣∣∣ (for a<0,b>0)
- r:−tan−1∣∣∣ba∣∣∣ (for a>0,b<0)
Step 3: Analyze a2+b2=0
- The equation a2+b2=0 implies that both a and b must be zero:
a=0,b=0
In this case, the argument is not defined because the complex number is z=0:
- t:not defined
Step 4: Analyze ab=0
- If ab=0, it means at least one of a or b is zero.
- If a=0 and b≠0, the argument is:
Argument=π2(if b>0)or0(if b<0)
- If b=0 and a≠0, the argument is:
Argument=0(if a>0)orπ(if a<0)
Thus, for ab=0, the possible argument is:
- u:0 or π2 (if a=0,b≠0)
Summary of Results
- For ab>0: p and q
- For ab<0: q and r
- For a2+b2=0: t
- For ab=0: u
- If ab>0, it means both a and b are either positive or both are negative.
- If both a and b are positive, the complex number lies in the first quadrant, and the argument is given by:
Argument=tan−1(ba)
- If both a and b are negative, the complex number lies in the third quadrant. The argument in this case can be calculated as:
Argument=tan−1(ba)−π
Thus, for ab>0, the possible argument is:
- p:tan−1∣∣∣ba∣∣∣ (for a>0,b>0)
- q:π+tan−1∣∣∣ba∣∣∣ (for a<0,b<0)
- If ab<0, it means one of a or b is positive and the other is negative.
- If a<0 and b>0, the complex number lies in the second quadrant, and the argument is:
Argument=π−tan−1∣∣∣ba∣∣∣
- If a>0 and b<0, the complex number lies in the fourth quadrant, and the argument is:
Argument=−tan−1∣∣∣ba∣∣∣
Thus, for ab<0, the possible argument is:
- q:π−tan−1∣∣∣ba∣∣∣ (for a<0,b>0)
- r:−tan−1∣∣∣ba∣∣∣ (for a>0,b<0)
- The equation a2+b2=0 implies that both a and b must be zero:
a=0,b=0
In this case, the argument is not defined because the complex number is z=0:
- t:not defined
- If ab=0, it means at least one of a or b is zero.
- If a=0 and b≠0, the argument is:
Argument=π2(if b>0)or0(if b<0)
- If b=0 and a≠0, the argument is:
Argument=0(if a>0)orπ(if a<0)
Thus, for ab=0, the possible argument is:
- u:0 or π2 (if a=0,b≠0)
- For ab>0: p and q
- For ab<0: q and r
- For a2+b2=0: t
- For ab=0: u
Topper's Solved these Questions
Similar Questions
Let O be the circumcenter, H be the orthocentre, I be the incenter, and I1,I2,I3 be the excenters of acute-angled ABC. Column I Column II Angle subtended by OI at vertex A p. |B−C| Angle subtended by HI at vertex A q. ∣∣∣B−C2∣∣∣ Angle subtended by OH at vertex A r. B+C2 Angle subtended by I2I3atI1 A q. B2−C
View SolutionLet L be the line of intersection of the planes 2x+3y+z=1 and x+3y+2z=2 . If L makes an angles α with the positive x-axis, then cosα equals
View Solution
Knowledge Check
Match the Column I and Column II.,B
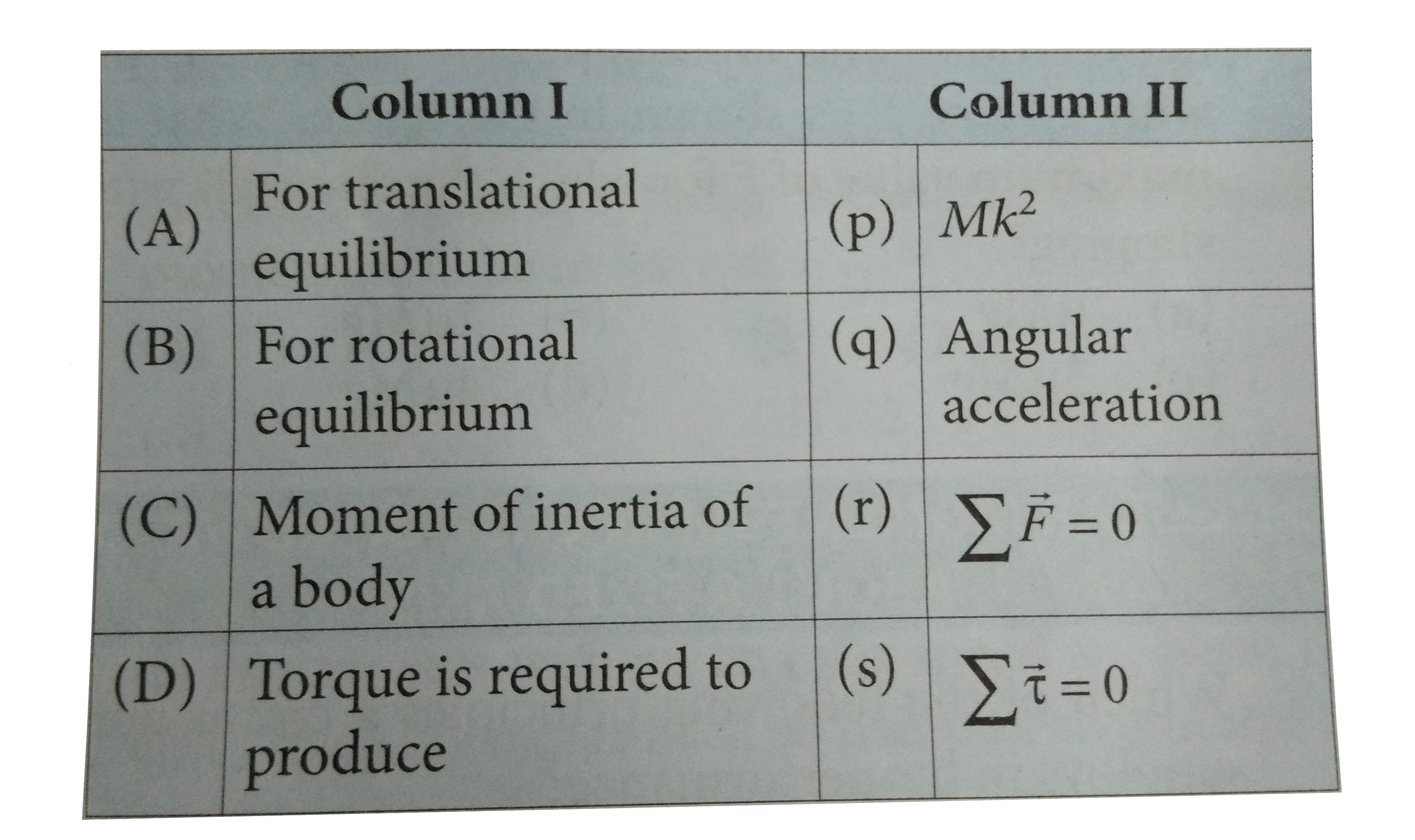 AA-p, B-q, C-r, D-sBA-q, B-r, C-s. D-pCA-r, B-q, C-p, D-pDA-r, B-s, C-p, D-q
AA-p, B-q, C-r, D-sBA-q, B-r, C-s. D-pCA-r, B-q, C-p, D-pDA-r, B-s, C-p, D-q
If ∣∣→A∣∣=2and∣∣→B∣∣=4, then match the relations in column I with the angleθ between →Aand→B in column II.
Column I, Column II
(a) ∣∣→A×→B∣∣=0 , (i) θ=30∘
(b) ∣∣→A×→B∣∣=0 , (ii) θ=45∘
(c ) →A×→B∣=4 , (iii) θ=90∘
(d) ∣∣→A×x→B∣∣=4√2 , (iv) θ=0∘.
View SolutionConsider a ABC is which side ABandAC are perpendicular to x−y−4=0and2x−y−5=0, respectively. Vertex A is (−2,3) and the circumcenter of ABC is (32,52). The equation of line in column I is of the form ax+by+c=0 , where a,b,c∈I. Match it with the corresponding value of c in column II Column I|Column II Equation of the perpendicular bisector of side BA |p. −1 Equation of the perpendicular bisector of side AC |q. 1 Equation of side AC |r. −16 Equation of the median through A |s. −4
View SolutionMatch the statement/expressions in Column I with the statements/expressions in Column II. Column I Column II The minimum value of x2+2x+4x+2 is (p) 0 Let AandBbe3x3 matrices of real numbers, where A is symmetric, B is skew symmetric, and (A+B)(A−B)=(A−B)(A+B) if (AB)t=(−1)kAB, where (AB)t is the transpose of the matrix AB , then the possible values of k are (q) 1 Let a=(log)3(log)32. An integer k satisfying 1<2−k+3(−1)<2, must be less than (r) 2 In sinθ=cosφ, then the possible values of 1π(θ±φ−π2) are (s) 3
View SolutionMatch the column I and column II and choose the correct option
(Column I) a.ADH b.Renin c.ANF d.angiotensin II
(Column II) i.Vasoconstrictor ii.Vasodilator iii.Released from pituitary gland iv.Released by JG cells
1) a(iii),b(iv),c(I),d(ii)
2) a(iv),b(iii),c(ii),d(i)
3) a(iv),b(iii),c(i),d(ii)
4) a(iii),b(iv),c(ii),d(i)
View SolutionMatch Column I with Column II. (a) Physalia (Column I),(i) Devil fish (Column II),(b) Ascaris (Column I),(ii) King crab (Column II),(c) Limulus (Column I),(iii) Roundworm (Column II),(d) Octopus (Column I),(iv) Portuguese man of war (Column II)
View SolutionMatch the columns : (a) Atrial systole (Column I) (i) 0.7 seconds (Column II) , (b) Joint diastole (Column I) (ii) 0.3 seconds (Columnn II) (c) Ventricular systole (Column I) (iii) 0.1 second (Column II) , (d) Atrial diastole (Column I) (iv) 0.4 seconds (Column II)
View SolutionMatch the column I with column II and choose correct option. Column I Column II A. Earthworm 1. Gizzard B. Cockroach 2. Caecum C. Frog 3. Clitellum D. Rat 4. Cloaca
View Solution