If the length of a common internal tangent to two circles is 7, and that of a common external tangent is 11, then the product of the radii of the two circles is (A) 36 (B) 9 (C) 18 (D) 4
To solve the problem, we need to find the product of the radii of two circles given the lengths of their common internal and external tangents.
Let the radii of the two circles be r1 and r2.
1. Given Information:
- Length of common internal tangent Li=7
- Length of common external tangent Le=11
2. Formulas:
- The formula for the length of the common internal tangent is:
Li=√d2−(r1+r2)2
- The formula for the length of the common external tangent is:
Le=√d2−(r1−r2)2
where d is the distance between the centers of the two circles.
3. Setting up equations:
- From the internal tangent:
7=√d2−(r1+r2)2(1)
- From the external tangent:
11=√d2−(r1−r2)2(2)
4. Squaring both equations:
- Squaring equation (1):
49=d2−(r1+r2)2⟹d2=49+(r1+r2)2(3)
- Squaring equation (2):
121=d2−(r1−r2)2⟹d2=121+(r1−r2)2(4)
5. Equating equations (3) and (4):
49+(r1+r2)2=121+(r1−r2)2
6. Rearranging the equation:
(r1+r2)2−(r1−r2)2=121−49
(r1+r2)2−(r1−r2)2=72
7. Using the identity:
(a+b)2−(a−b)2=4ab
Here, let a=r1 and b=r2:
4r1r2=72
8. Solving for the product of the radii:
r1r2=724=18
Thus, the product of the radii of the two circles is 18.
- Length of common internal tangent Li=7
- Length of common external tangent Le=11
- The formula for the length of the common internal tangent is:
Li=√d2−(r1+r2)2
- The formula for the length of the common external tangent is:
Le=√d2−(r1−r2)2
where d is the distance between the centers of the two circles.
- From the internal tangent:
7=√d2−(r1+r2)2(1)
- From the external tangent:
11=√d2−(r1−r2)2(2)
- Squaring equation (1):
49=d2−(r1+r2)2⟹d2=49+(r1+r2)2(3)
- Squaring equation (2):
121=d2−(r1−r2)2⟹d2=121+(r1−r2)2(4)
49+(r1+r2)2=121+(r1−r2)2
(r1+r2)2−(r1−r2)2=121−49
(r1+r2)2−(r1−r2)2=72
(a+b)2−(a−b)2=4ab
Here, let a=r1 and b=r2:
4r1r2=72
r1r2=724=18
Topper's Solved these Questions
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - CENGAGE ENGLISHCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - All Questions
886 Videos
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type
11 Videos
Similar Questions
The length of a common internal tangent to two circles is 5 and a common external tangent is 15, then the product of the radii of the two circles is :
View SolutionThe length of a common internal tangent to two circles is 5 and that of a common external tangent is 13. If the product of the radii of two circles is λ, then the value of λ4 is
View SolutionThe length of the common chord of two circles of radii r1 and r2 which intersect at right angles is
View SolutionThe length of the common chord of two circles of radii 15 and 20, whose centres are 25 units apart, is
View SolutionThe length of the common chord of the two circles of radii 10, 24 whose centres are 26 units apart is
View SolutionIf the distance between the centres of two circles of radio 3,4 is 25 then the length of the tranverse common tangent is
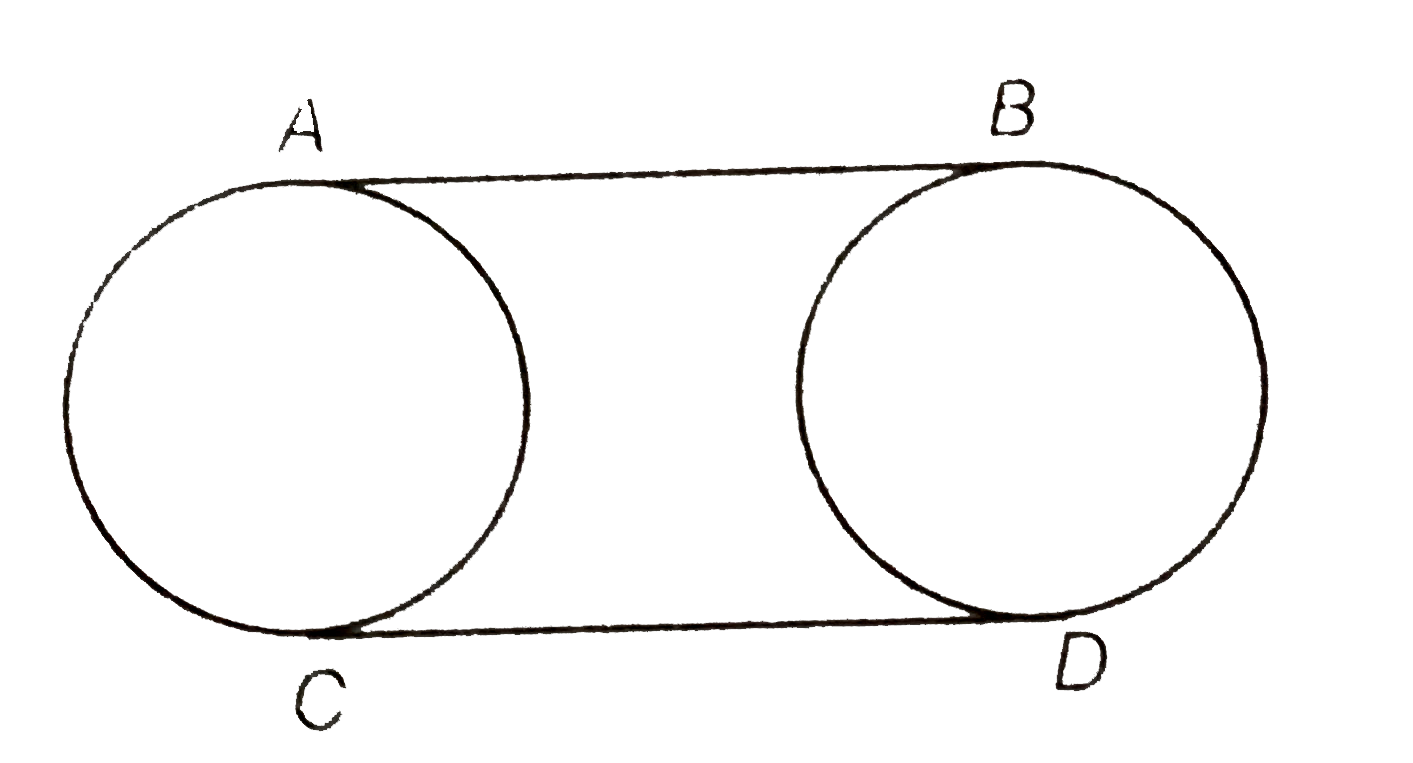
View SolutionIn figure, AB and CD are common tangents to two circles of equal radii. Prove that AB=CD.
View SolutionIf the length of the tangent from two points A,B to a circle are 6,7 respectively. If A,B are conjugate points then AB=
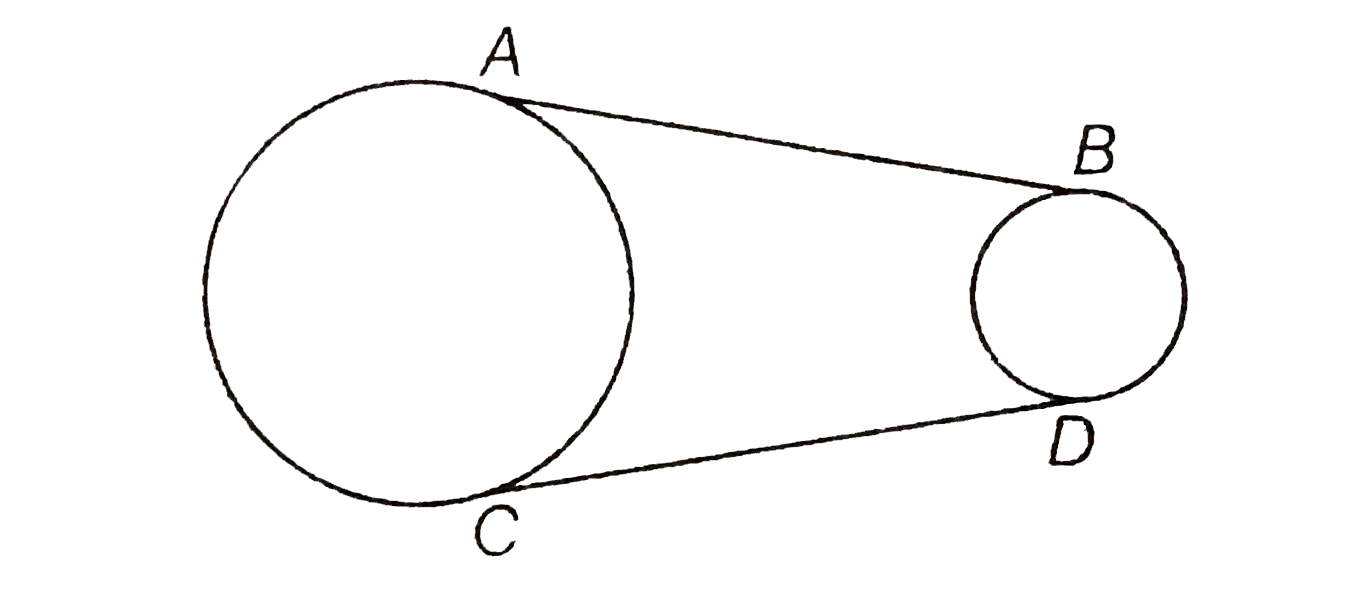
View SolutionIn figure, AB and CD are common tangents to two circles of unequal radii. Prove that AB=CD
View SolutionIn the adjoining figure, common tangents AB and CD to two circles intersect at P.
Prove that AB=CD.

View Solution
