An insurance company selected 2000 drivers at random (i.e., without any preference of one driver over another) in a particular city to find a relationship between age and accidents. The data obtained are given in the following table: Find the probabilities of the following events for a driver chosen at random from the city: (i) The driver being in the age group 18−29 years and having exactly 3 accidents in one year. (ii) The driver being in the age group of 30−50 year and having one or more accidents in a year. (iii) The number of drivers having no accidents in one year
To solve the problem, we will follow these steps for each part of the question:
Given Data:
The total number of drivers surveyed = 2000
Step 1: Analyze the data table
We need to refer to the data table that categorizes drivers based on their age groups and the number of accidents they have had in a year. The age groups are:
1. 18−29 years
2. 30−50 years
3. Over 50 years
For each age group, we will have the frequency of drivers based on the number of accidents (0, 1, 2, 3, and more than 3).
Step 2: Calculate the probabilities
Part (i): Probability of a driver being in the age group 18−29 years and having exactly 3 accidents.
- From the data, the number of drivers aged 18−29 years with exactly 3 accidents = 61.
- Probability (P) = Number of favorable outcomes / Total number of outcomes
- Therefore, P = 61 / 2000
Final Answer for Part (i):
P(18−29 years and exactly 3 accidents)=612000
Part (ii): Probability of a driver being in the age group of 30−50 years and having one or more accidents.
- From the data, we need to sum the frequencies of drivers aged 30−50 years with:
- 1 accident = 125
- 2 accidents = 60
- 3 accidents = 22
- More than 3 accidents = 18
- Total drivers aged 30−50 years with one or more accidents = 125 + 60 + 22 + 18 = 225.
- Therefore, P = 225 / 2000
Final Answer for Part (ii):
P(30−50 years and one or more accidents)=2252000=980
Part (iii): Probability of the number of drivers having no accidents in one year.
- From the data, we need to sum the frequencies of drivers with 0 accidents across all age groups:
- Age 18−29 years = 440
- Age 30−50 years = 505
- Age over 50 years = 360
- Total drivers with no accidents = 440 + 505 + 360 = 1305.
- Therefore, P = 1305 / 2000
Final Answer for Part (iii):
P(no accidents)=13052000=261400
Summary of Answers:
1. P(18−29 years and exactly 3 accidents)=612000
2. P(30−50 years and one or more accidents)=980
3. P(no accidents)=261400
The total number of drivers surveyed = 2000
We need to refer to the data table that categorizes drivers based on their age groups and the number of accidents they have had in a year. The age groups are:
1. 18−29 years
2. 30−50 years
3. Over 50 years
- From the data, the number of drivers aged 18−29 years with exactly 3 accidents = 61.
- Probability (P) = Number of favorable outcomes / Total number of outcomes
- Therefore, P = 61 / 2000
P(18−29 years and exactly 3 accidents)=612000
- From the data, we need to sum the frequencies of drivers aged 30−50 years with:
- 1 accident = 125
- 2 accidents = 60
- 3 accidents = 22
- More than 3 accidents = 18
- Total drivers aged 30−50 years with one or more accidents = 125 + 60 + 22 + 18 = 225.
- Therefore, P = 225 / 2000
P(30−50 years and one or more accidents)=2252000=980
- From the data, we need to sum the frequencies of drivers with 0 accidents across all age groups:
- Age 18−29 years = 440
- Age 30−50 years = 505
- Age over 50 years = 360
- Total drivers with no accidents = 440 + 505 + 360 = 1305.
- Therefore, P = 1305 / 2000
P(no accidents)=13052000=261400
1. P(18−29 years and exactly 3 accidents)=612000
2. P(30−50 years and one or more accidents)=980
3. P(no accidents)=261400
Topper's Solved these Questions
Similar Questions
An insurance company selected 2000 drivers at random (i.e., without any preference of one driver over another) in a particular city to find a relationship between age and accidents. The data obtained are given in the following table:A g e\ o
View SolutionAn insurance company selected 2000 drivers at random in a particular city to find a relationship between age and accidents. The obtained are given in the following table: Age of Drivers (in years) Accidents in one year 0 1 2 3 over 3 18-29 30-50 above 50 440 505 360 160 125 45 110 60 35 61 22 15 35 18 9 Find the probability of the following events for a driver chosen at random from the life city: i) being 18-29 years of age and having exactly 3 accidents in one year. ii) being 30-50 years of age and having one or more accidents in a year. iii) having no accidents in one year.
View SolutionThe ages of 37 students in a class are given in the following table: Find the median
View SolutionTo know the opinion of the students about Mathematics, a survey of 200 students was conducted. The data is recorded in the following table: Find the probability that a student chosen at random (i) likes Mathematics (ii) does not like it.
View SolutionFind the probability that the leap year selected at random will contain of exactly 52 Wednesdays.
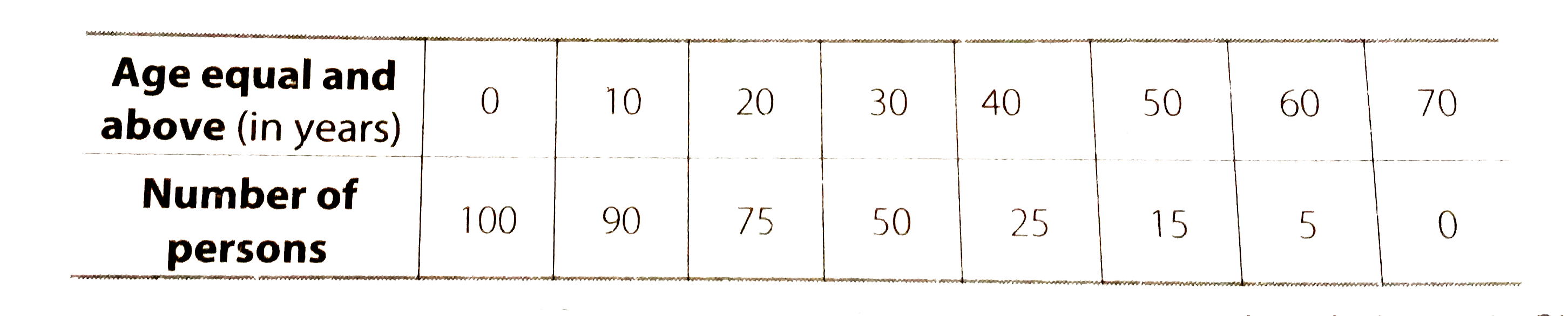
View SolutionFind the mean age of 100 residents of a town from the following data.
View SolutionA company selected 4000 households at random and surveyed them to find out a relationship between income level and the number of televisions sets in a home. The information, so obtained is listed in the following table.
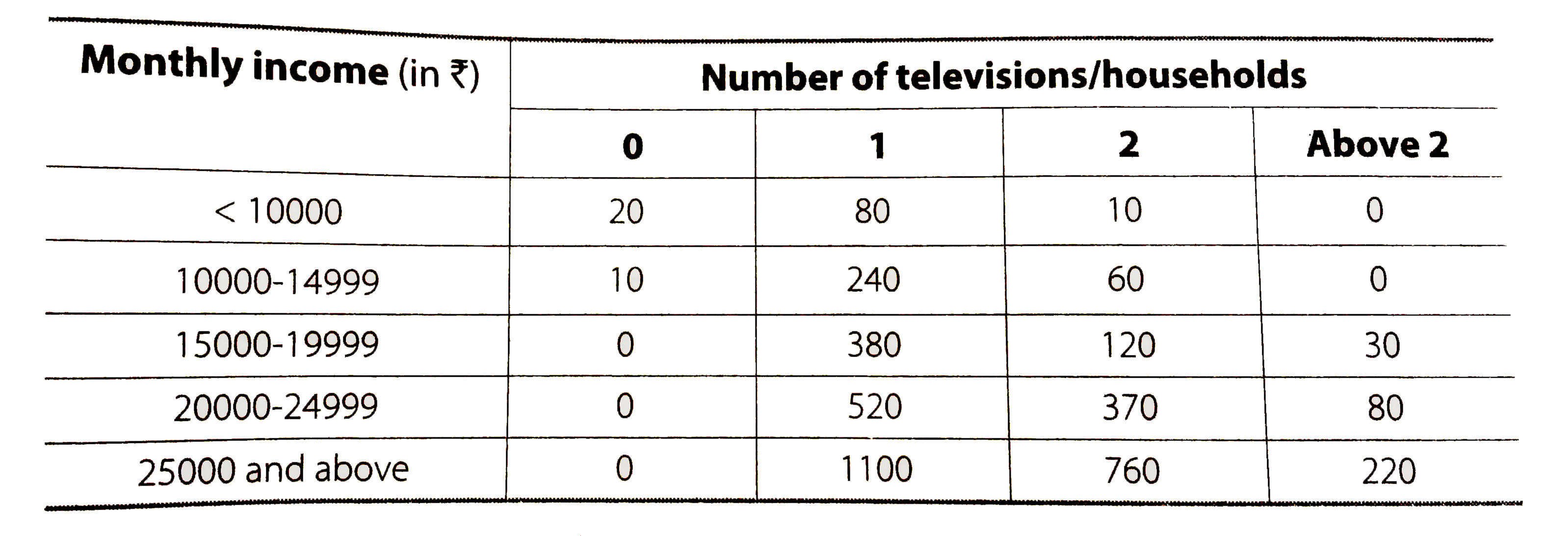
Find the probability
i) of a household earning Rs 10000- Rs 14999 per year and having exactly one television.
ii) of a household earning Rs 25000 and more per year owning 2 televisions.
iii) of a household not having any television.
View SolutionFind the probability that a leap year, selected at random, will contain 53 Sundays.
View SolutionFind the probability that a leap year, selected at random, will contain 53 Sundays.
View SolutionTwo integers are chosen at random and multiplied. Find the probability that the product is an even integer.
View Solution
