Four cows are tethered at four corners of a square plot of side 50m, so that they just cannot reach on another. What area will be left ungrazed?
To solve the problem of finding the area left ungrazed by the cows tethered at the corners of a square plot, we can follow these steps:
1. Understand the Problem: We have a square plot with side length 50 m, and cows are tethered at each corner. Each cow can graze in a circular area, but since they are tethered at the corners, their grazing areas will overlap in the center of the square.
2. Calculate the Area of the Square:
Area of the square=side2=502=2500m2
3. Determine the Radius of Grazing: Since the cows are tethered at the corners and cannot reach each other, the radius of the grazing area for each cow is half the side of the square:
r=502=25m
4. Calculate the Area of One Quadrant: The grazing area for each cow is a quarter of a circle (quadrant) since they are at the corners. The area of a full circle is given by πr2, so the area of one quadrant is:
Area of one quadrant=14πr2=14π(252)=14π(625)=625π4≈491.07m2
5. Calculate the Total Grazing Area: Since there are four cows, the total grazing area is:
Total grazing area=4×Area of one quadrant=4×625π4=625π≈1963.5m2
6. Calculate the Ungrazed Area: The ungrazed area is the area of the square minus the total grazing area:
Ungrazed area=Area of the square−Total grazing area=2500−625π≈2500−1963.5≈536.5m2
Final Answer:
The area left ungrazed is approximately 536.5m2.
---
Area of the square=side2=502=2500m2
r=502=25m
Area of one quadrant=14πr2=14π(252)=14π(625)=625π4≈491.07m2
Total grazing area=4×Area of one quadrant=4×625π4=625π≈1963.5m2
Ungrazed area=Area of the square−Total grazing area=2500−625π≈2500−1963.5≈536.5m2
The area left ungrazed is approximately 536.5m2.
Topper's Solved these Questions
Similar Questions
Find the area of a square plot of side 8 m.
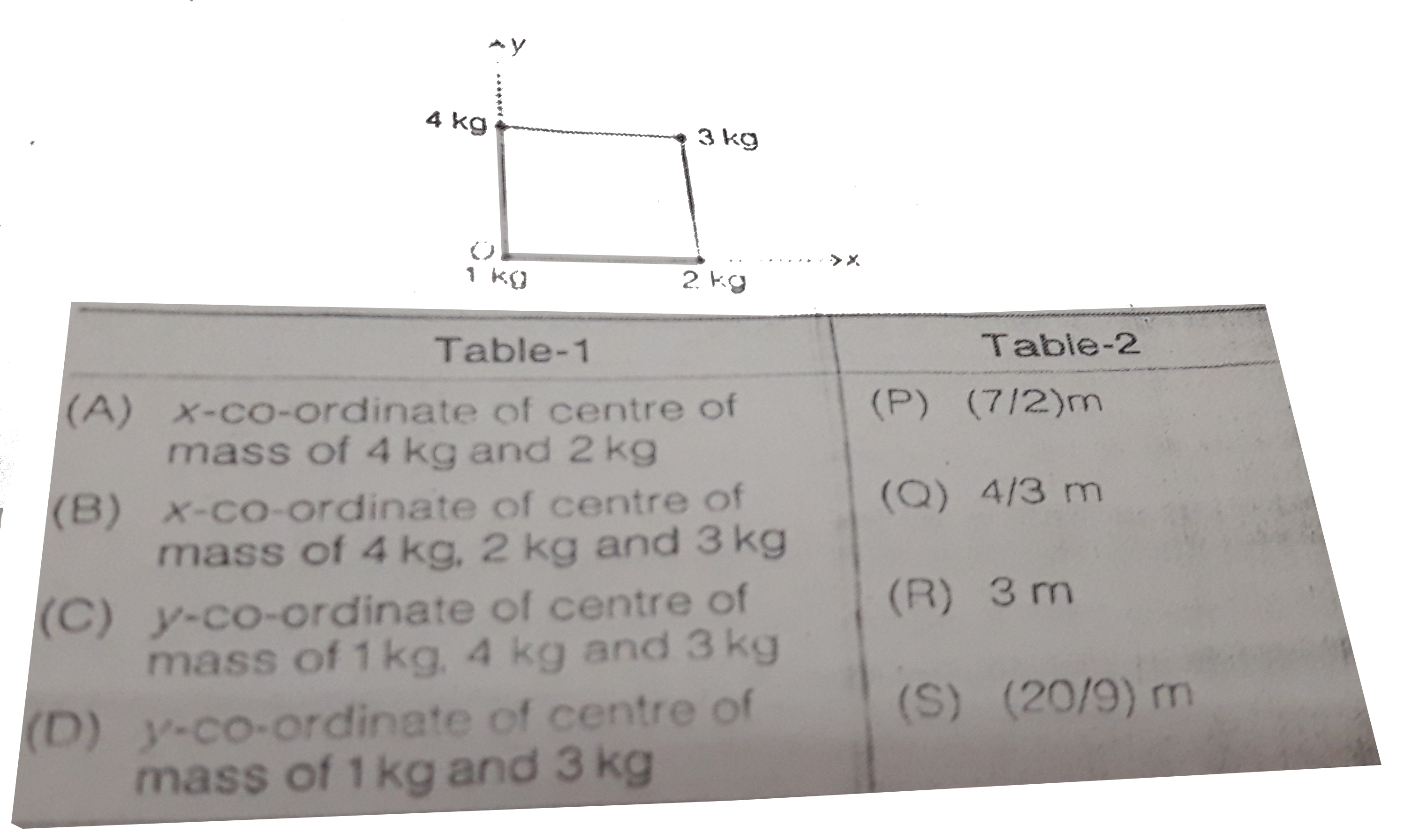
View SolutionFour point are placed at four comerse of a squared of side 4 m as shown. Match the following table.
View SolutionFour point charges are placed at the corners of a square of side l. Calculate potential at the centre of square.

View SolutionFour charges of same magnitude q are placed at four corners of a square of side a . The value electric potential at the centre of the souare will be (where k = 14πε0 )
View SolutionFour spheres A, B, C, D, each of mass m and diameter 2 a are placed with their centres at die four corners of a square of side b. What is the moment of inertia of the system about any side of the square ?
View SolutionFour particle of mass m each are placed at four corners of a square ABCD of side a. Point O is the centre of the square. Moment of inertia of all four particles about an axis passing through
View SolutionFour identical point charges q are placed at four corners of a square of side a. Find the potential energy of the charge system.
 .
.
View SolutionFour point masses are plaaced at four corners of a square as shows. When positions of mand 2m are interchanged

View SolutionFour point masses are placed at the corners of a square of side 2 m as shown in the figure. Find the centre of mass of the system w.r.t. the centre of square.

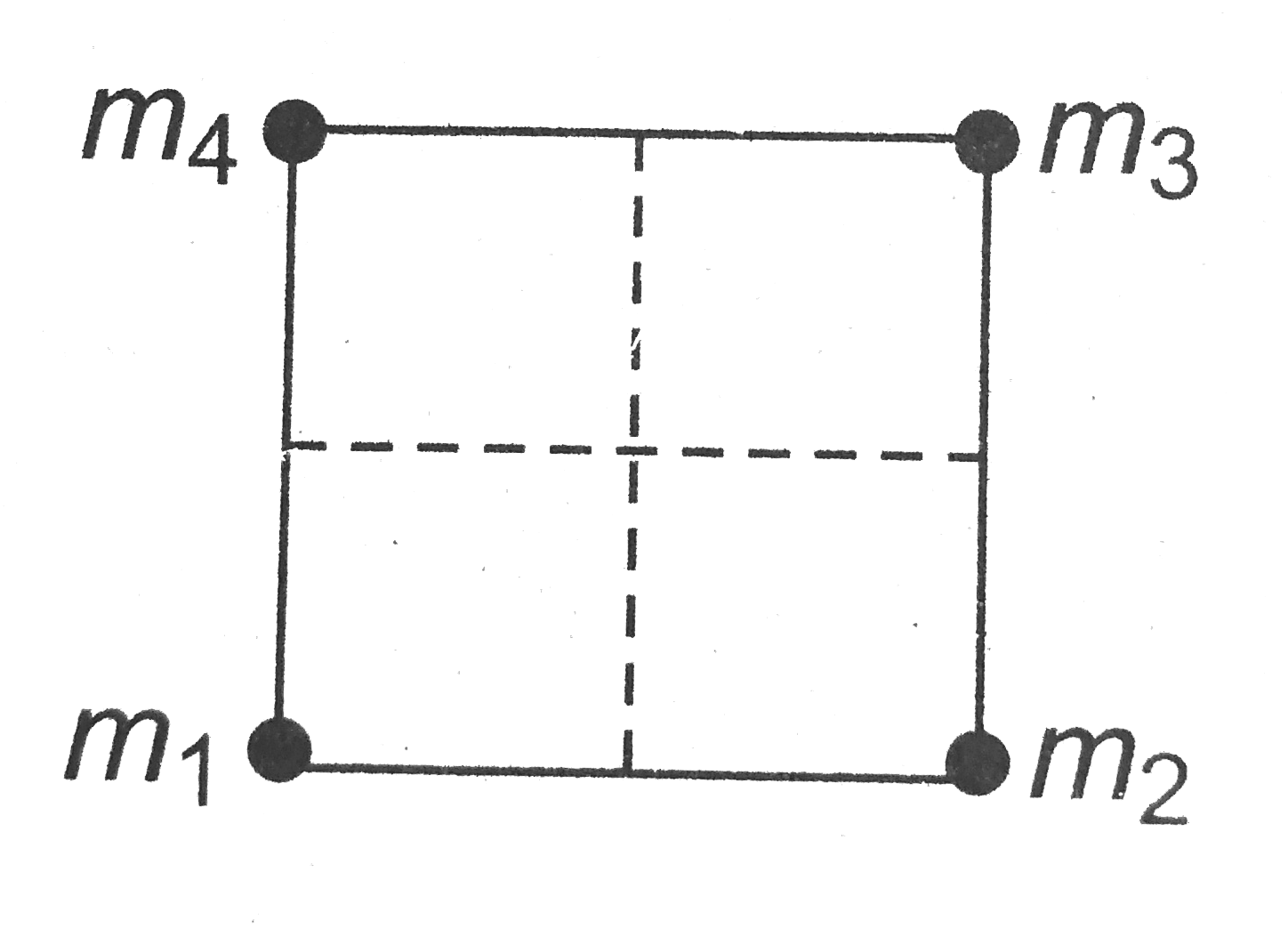
View SolutionFour paticle of masses m1=2m,m2=4m,m3=mandm4 are placed at four corners of a square. What should be the value of m4 so that the centres of mass of all the four particle are exactly at the centre of the square ?
View Solution
