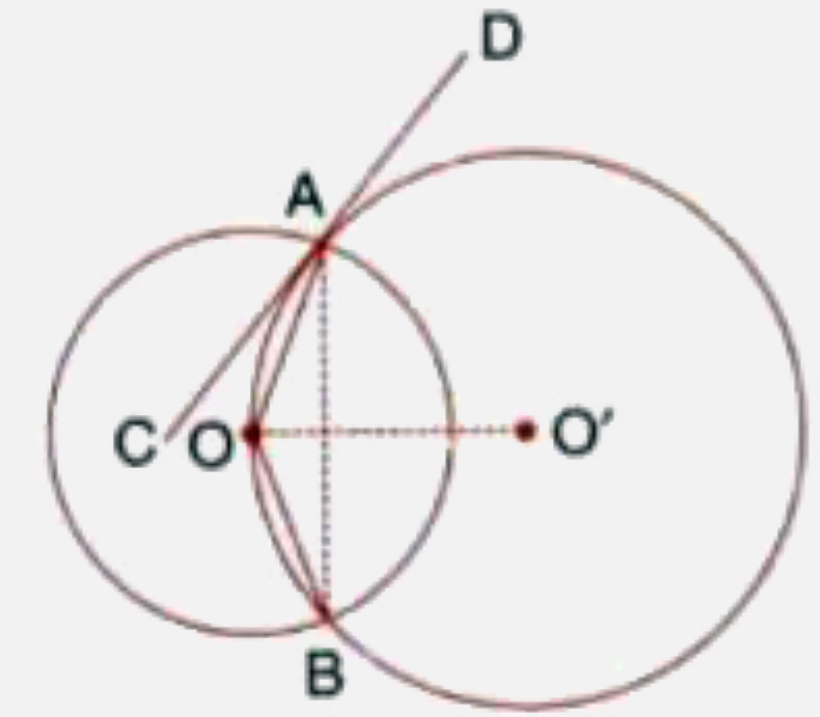
. In the given figure, two equal circles, with centres O and O', touch each other at X. OO' produced me the circle with centre O' at A. AC is tangent to the circle with centreO, at the point C. O'D is perpendicular to AC. Find the value of DO'CO.
To solve the problem step-by-step, we will analyze the given information and apply the properties of similar triangles.
Step 1: Understand the Configuration
We have two equal circles with centers O and O' that touch each other at point X. The line OO' is extended to point A, which is on the circumference of the circle with center O'. AC is a tangent to the circle with center O at point C, and O'D is perpendicular to AC.
Step 2: Identify the Triangles
We will focus on two triangles:
1. Triangle AOD (where D is on the line O'D)
2. Triangle AOC (where C is the point of tangency)
Step 3: Establish Angles
Since AC is a tangent to the circle at point C, we know that:
- Angle OCA = 90 degrees (because the radius at the point of tangency is perpendicular to the tangent).
Also, since O'D is perpendicular to AC:
- Angle O'DA = 90 degrees.
Thus, we have:
- Angle O'DA = Angle OCA = 90 degrees.
Step 4: Identify Common Angle
Both triangles AOD and AOC share angle A, which is common to both triangles.
Step 5: Apply AA Similarity Criterion
Since we have two angles equal (Angle O'DA = Angle OCA and Angle A is common), we can conclude that:
- Triangle AOD is similar to triangle AOC (by AA criterion).
Step 6: Set Up the Proportions
From the similarity of triangles, we can write the following proportion:
O′DOC=O′AOA
Let’s denote:
- O'A = r (the radius of the circle),
- OA = O'A + O'X + OX = r + r + r = 3r (since OX and O'X are equal as both circles are equal).
Step 7: Substitute Values
Now substituting the values into the proportion:
O′DOC=r3r
This simplifies to:
O′DOC=13
Step 8: Find the Required Ratio
We need to find the value of DO′CO. Since O'D is equal to O'D and OC is equal to OC, we can rewrite:
DO′CO=O′DOC=13
Final Answer
Thus, the value of DO′CO is 13.
---
We have two equal circles with centers O and O' that touch each other at point X. The line OO' is extended to point A, which is on the circumference of the circle with center O'. AC is a tangent to the circle with center O at point C, and O'D is perpendicular to AC.
We will focus on two triangles:
1. Triangle AOD (where D is on the line O'D)
2. Triangle AOC (where C is the point of tangency)
Since AC is a tangent to the circle at point C, we know that:
- Angle OCA = 90 degrees (because the radius at the point of tangency is perpendicular to the tangent).
- Angle O'DA = 90 degrees.
- Angle O'DA = Angle OCA = 90 degrees.
Both triangles AOD and AOC share angle A, which is common to both triangles.
Since we have two angles equal (Angle O'DA = Angle OCA and Angle A is common), we can conclude that:
- Triangle AOD is similar to triangle AOC (by AA criterion).
From the similarity of triangles, we can write the following proportion:
O′DOC=O′AOA
Let’s denote:
- O'A = r (the radius of the circle),
- OA = O'A + O'X + OX = r + r + r = 3r (since OX and O'X are equal as both circles are equal).
Now substituting the values into the proportion:
O′DOC=r3r
This simplifies to:
O′DOC=13
We need to find the value of DO′CO. Since O'D is equal to O'D and OC is equal to OC, we can rewrite:
DO′CO=O′DOC=13
Thus, the value of DO′CO is 13.
Topper's Solved these Questions
Similar Questions
In the figure, two equal chords AB and CD of a circle with centre O, intersect each other at E, Prove that AD=CB
View SolutionIn figure-3, PQ is tangent to the circle with centre at O, at the point B. if ∠AOB=100∘, then ∠ABP is equal to
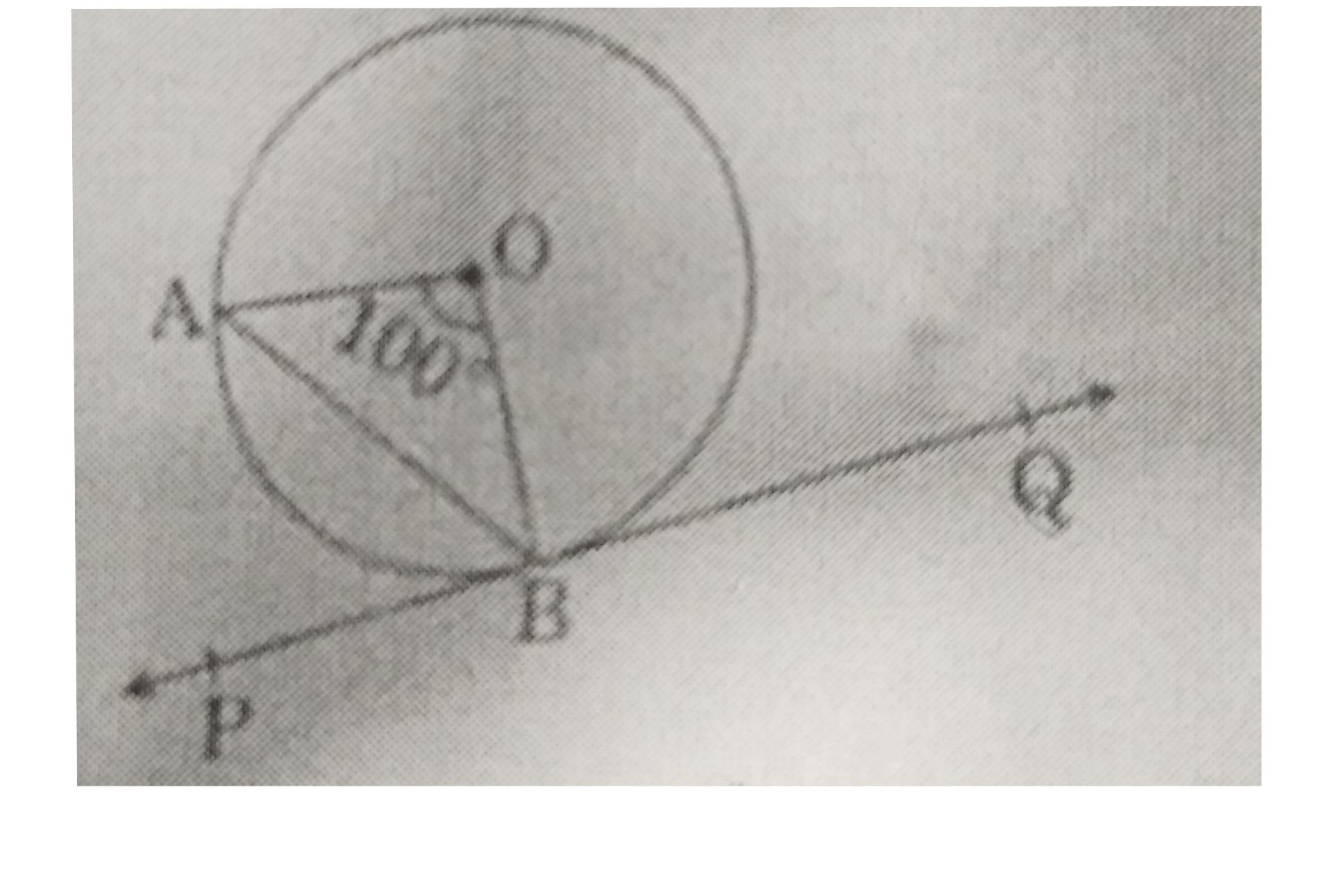
View SolutionIn figure PQ is tangent to the circle with centre at O at the point B , if ∠AOB=100∘, then ∠ABP is equal to

View SolutionIn the given figure, a square is inscribed in a circle with centre O. Find :
∠BOD .Is BD a diameter of the circle
View SolutionTwo circles with centres O and O'are drawn to intersect each other at points A and B. Centre O of one circle lies on the circumference of the other circle and CD is drawn tangent to the circle with centre O' at A. prove that OA bisects angle BAC.
View SolutionPQ is a tangent to a circle with centre O at the point P . If △OPQ is an isosceles triangle, then ∠OQP is equal to :
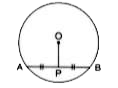
View SolutionThe given figure shows a circle with centre O. P is mid-point of chord AB.
Show that OP is perpendicular to AB.
View SolutionIn the given figure, a square is inscribed in a circle with centre O. Find :
∠OCB . Is BD a diameter of the circle
View SolutionIn the given figure, a square is inscribed in a circle with centre O. Find :
∠COD . Is BD a diameter of the circle
View SolutionIn the given figure, a square is inscribed in a circle with centre O. Find :
∠BOC . Is BD a diameter of the circle
View Solution