Question

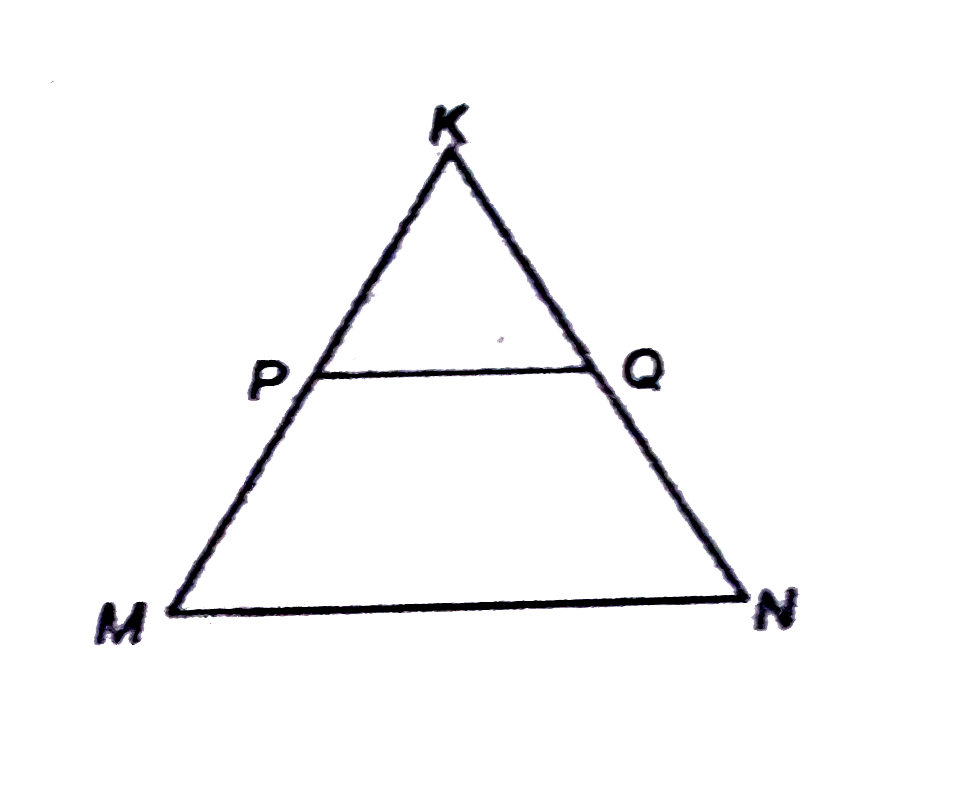
In Figure ${\displaystyle PQ}$
is parallel to ${\displaystyle MN\stackrel{.}{}}$
if ${\displaystyle \frac{KP}{PM}=4/13}$
and ${\displaystyle KN=20.4cm\stackrel{.}{}}$
Find KQ.

Explanation

To solve the problem step by step, we will use the Basic Proportionality Theorem (also known as Thales' theorem), which states that if a line is drawn parallel to one side of a triangle, it divides the other two sides proportionally.

1. Identify the Given Information:
- We have two parallel lines: \( PQ \parallel MN \).
- The ratio \( \frac{KP}{PM} = \frac{4}{13} \).
- The length \( KN = 20.4 \) cm.

2. Set Up the Proportionality:
- According to the Basic Proportionality Theorem:
\( \frac{KP}{PM} = \frac{KQ}{QN} \)
- We can express \( QN \) in terms of \( KQ \):
\( QN = KN - KQ \)
- Thus, we can rewrite the proportion:
\( \frac{KP}{PM} = \frac{KQ}{KN - KQ} \)

3. Substitute the Known Values:
- Substitute \( \frac{KP}{PM} = \frac{4}{13} \) and \( KN = 20.4 \):
\( \frac{4}{13} = \frac{KQ}{20.4 - KQ} \)

4. Cross-Multiply to Solve for \( KQ \):
- Cross-multiplying gives:
\( 4(20.4 - KQ) = 13KQ \)
- Expanding this:
\( 81.6 - 4KQ = 13KQ \)

5. Combine Like Terms:
- Rearranging the equation:
\( 81.6 = 13KQ + 4KQ \)
- This simplifies to:
\( 81.6 = 17KQ \)

6. Solve for \( KQ \):
- Dividing both sides by 17:
\( KQ = \frac{81.6}{17} \)
- Calculating the value:
\( KQ = 4.8 \text{ cm} \)

Final Answer:
Hence, the value of \( KQ \) is \( 4.8 \) cm.